What is the Tetrahedron Problem in H.E. Huntley's 'The Divine Proportion'?

[Dschumanji]

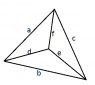
I was skimming through the book "The Divine Proportion a Study in Mathematical Beauty" by H.E. Huntley and found an interesting passage labeled "The Tetrahedron Problem." The problem is stated like this:

The faces of a tetrahedron are all scalene triangles similar to one another, but not all congruent, with integral sides. The longest side does not exceed 50. Show its network. The limitation to integral values being waived, show that the ratio of the length of the longest to that of the shortest edge has a limiting value, and find it.

This is how his solution begins:

Two triangles may have five parts of the one congruent with five parts of the other without being congruent triangles. If the triangles are not congruent, their congruent parts cannot include the three sides. Hence the triangles must be equiangular, and it is easily shown that the lengths of the sides must be in geometrical progression...

I've been scratching my head wondering what the hell this guy is trying to convey with the first two sentences (no images are provided in the text). What makes it even worse is the absence of a proof that the sides of whatever he is talking about MUST be in geomtric progression. Could anyone shed some light on this?

 

[uart]

Yeah he does a bad job of explaining it, but putting it simply he's looking at how many sides can two similar triangles have in common without being congruent.

Obviously if they're similar all then all angles are the same (equiangular) but he goes further to say that they can also have 2 equal sides if the sides are in a geometric ratio.

For example consider the two triangles with sides (1, 1.5, 2.25) and (1.5, 2.25 and 3.375). These are similar because they have all three sides in the same ratio (1:1.5) but clearly they are not congruent. Those two triangles also have 5 things in common, two sides and three angles. Clearly this is the "best" (most things in common) we can do without making the two triangles congruent.

Hope that helps.

 

[Dschumanji]

That is extremely helpful, Uart! Thank you so much!

 

[willem2]

I don't think the proof that the sides must be in a geometric procession is
all that obvious.

suppose you have a tetrahedron with edges a,b,c,d,e,f

It's always possible to rotate the tetrahedron, so a there is no larger side than a,
and then reflect it, so that b<c. b=c isn't possible, because triangle (a,b,c) is scalene.

This means that b,c,d and f are larger than a, because they are a part of a triangle that also has a as a side.

since a is the smallest side of triangle (a,b,c) as well as triangle (a,f,d) we must have

(f/a = b/a and e/a = c/a) => (f=b and e = c) or
(e/a = b/a and f/a = c/a) => (e=b and f=c)

e = b isn't possible since triang;e (b,d,e) is scalene, so the only remaining option is

f = b and d = c

If we now look at triangle (b,d,e), the ratio d/b = c/b must be equal to b/a or to c/a

c/b = c/a gives a = c which isn't possible with scalene triangles, so c/b = b/a and b^2 = ac.
b is the geometric mean of a and c. This proves a,b,c is a geometric series, with ratio: r = $\sqrt {\frac {c} {a}}$

b = f = ra
c = d = (r^2)a

since c>a, r>1

since d/b is equal to b/a, e/b must be equal to c/a or a/c

e/b = a/c => ec = ab => e(r^2)a = a (ra) => re = a, so e<a, but a was the smallest side.

e/b = c/a => bc = ae => (r^3)(a^2) = ae => e = a(r^3), so e is the next term of the geometric series.

 

[CellCoree]

I would approach this problem by first clarifying the terminology and concepts being used. A tetrahedron is a three-dimensional shape with four triangular faces. A scalene triangle is a triangle with all sides of different lengths. Similar triangles have the same shape but may be different sizes. Congruent triangles have the same shape and size. Integral values refer to whole numbers.

Based on the information provided, it seems that the author is trying to find the network of a tetrahedron with similar but not congruent scalene triangles as faces. The limitation of the longest side not exceeding 50 adds an additional constraint to the problem.

The author's solution begins by stating that two triangles can have five congruent parts without being congruent triangles. This may be confusing, but it is a known fact in geometry. Two triangles can have the same angles, but different side lengths. This is known as the Angle-Angle-Side (AAS) congruence theorem. The author then states that if the triangles are not congruent, their congruent parts cannot include the three sides. This is also a known fact, as the Side-Angle-Side (SAS) congruence theorem states that two triangles with two congruent sides and an included angle are congruent. This means that the triangles in question must be equiangular, meaning they have the same angles.

The author then mentions that it can be easily shown that the lengths of the sides must be in a geometric progression. This means that the side lengths increase or decrease by a constant ratio. For example, if the first side is 2 units long, the second side would be 4 units long, and the third side would be 8 units long. This is a common property of similar triangles.

To fully understand the author's solution, a proof would be needed to show why the side lengths must be in a geometric progression. Without this proof, it may be difficult to fully understand the solution. It is also important to note that the author mentions the limitation of integral values being waived, which means that they are not necessarily required for the solution.

In conclusion, the author is discussing a problem involving a tetrahedron with similar but not congruent scalene triangles as faces. The solution involves the triangles being equiangular and the side lengths being in a geometric progression. Without a proof, it may be difficult to fully understand the solution, but it is based on known properties