- #1
Math Amateur
Gold Member
MHB
- 3,990
- 48
I am baffled by some aspects of the torus ... I hope someone can help ...
I am puzzled by some aspects of Singh's treatment of the torus in Example 2.2.5 ( Tej Bahadur Singh: Elements of Topology, CRC Press, 2013) ... ...
Singh's Example 2.2.5 reads as follows:
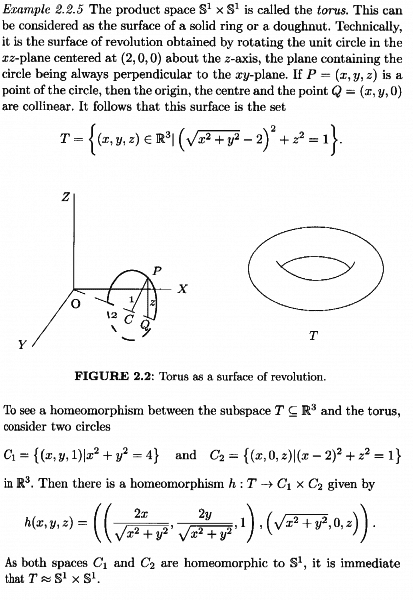
My questions related to the above example of Singh's are as follows:Question 1
[itex]C_1[/itex] as defined above seems to me to be a circle at 'height' [itex]z = 1[/itex], around the [itex]z[/itex]-axis ... why (for what reason?) did Singh choose [itex]C_1[/itex] to be at 'height' [itex]z = 1[/itex]?
Why not choose [itex]C_1[/itex] as [itex]\{ (x,y, 0) \ | \ x^2 + y^2 = 4 \}[/itex]?
Such a choice seems more natural if you are rotating [itex]C_2[/itex] around the [itex]z[/itex]-axis, since [itex]C_1[/itex] is at level [itex]z = 0[/itex] ... ...Question 2
[itex]h \ : \ T \rightarrow C_1 \times C_2[/itex]
maps [itex](x,y,z)[/itex] onto two three dimensional points in Euclidean [itex]3[/itex]-space and so essentially maps [itex](x,y,z)[/itex] into Euclidean [itex]6[/itex]-space ... ..
... BUT ...
[itex]T[/itex] is homeomorphic to [itex]S^1 \times S^1[/itex] which is embedded in Euclidean [itex]4[/itex]-space ... ... how can this be ...
I hope someone can clarify the above issues/questions ...
Peter
I am puzzled by some aspects of Singh's treatment of the torus in Example 2.2.5 ( Tej Bahadur Singh: Elements of Topology, CRC Press, 2013) ... ...
Singh's Example 2.2.5 reads as follows:
My questions related to the above example of Singh's are as follows:Question 1
[itex]C_1[/itex] as defined above seems to me to be a circle at 'height' [itex]z = 1[/itex], around the [itex]z[/itex]-axis ... why (for what reason?) did Singh choose [itex]C_1[/itex] to be at 'height' [itex]z = 1[/itex]?
Why not choose [itex]C_1[/itex] as [itex]\{ (x,y, 0) \ | \ x^2 + y^2 = 4 \}[/itex]?
Such a choice seems more natural if you are rotating [itex]C_2[/itex] around the [itex]z[/itex]-axis, since [itex]C_1[/itex] is at level [itex]z = 0[/itex] ... ...Question 2
[itex]h \ : \ T \rightarrow C_1 \times C_2[/itex]
maps [itex](x,y,z)[/itex] onto two three dimensional points in Euclidean [itex]3[/itex]-space and so essentially maps [itex](x,y,z)[/itex] into Euclidean [itex]6[/itex]-space ... ..
... BUT ...
[itex]T[/itex] is homeomorphic to [itex]S^1 \times S^1[/itex] which is embedded in Euclidean [itex]4[/itex]-space ... ... how can this be ...
I hope someone can clarify the above issues/questions ...
Peter
Attachments
Last edited:
