- #1
gevni
- 25
- 0
Hi, Can someone please help me in understanding few parameters of cubic equation formula for solving polynomial of degree three. I attached the formula in the screenshot.
My questions are:
(1) what is ". " dot in the end of the formula and what does it mean?
(2) I want to use it only for real number not complex, so do the formula remain the same?
(3) It states the solution is for this kind of equation\[ ax^3+ax^2+cx+d=0 \] what if I have equation like that \[ ax^3+ax^2-cx+d=0 \]? Do i have to put negative value with c parameter in the below formula or whatever the case formula remain same?
(4) It is just one formula then how I can get three roots by using this?
(5) I want to use this formula for getting the minimum possible value of x and if it results in 3 roots then which root show minimum value of x.
I am very thankful if someone help me understanding this formula or any resource that can help me understanding this.
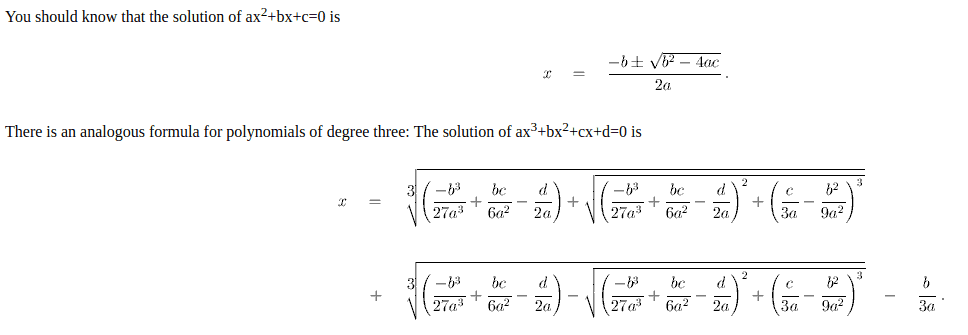
My questions are:
(1) what is ". " dot in the end of the formula and what does it mean?
(2) I want to use it only for real number not complex, so do the formula remain the same?
(3) It states the solution is for this kind of equation\[ ax^3+ax^2+cx+d=0 \] what if I have equation like that \[ ax^3+ax^2-cx+d=0 \]? Do i have to put negative value with c parameter in the below formula or whatever the case formula remain same?
(4) It is just one formula then how I can get three roots by using this?
(5) I want to use this formula for getting the minimum possible value of x and if it results in 3 roots then which root show minimum value of x.
I am very thankful if someone help me understanding this formula or any resource that can help me understanding this.
Last edited: