Automorphisms of Central Simple Algebras/Bresar Example 1.27

[Math Amateur]

I am reading Matej Bresar's book, "Introduction to Noncommutative Algebra" and am currently focussed on Chapter 1: Finite Dimensional Division Algebras ... ...

I need help with some aspects of Example 1.27...

Example 1.27 (including a short preamble and Definition 1.26) reads as follows:

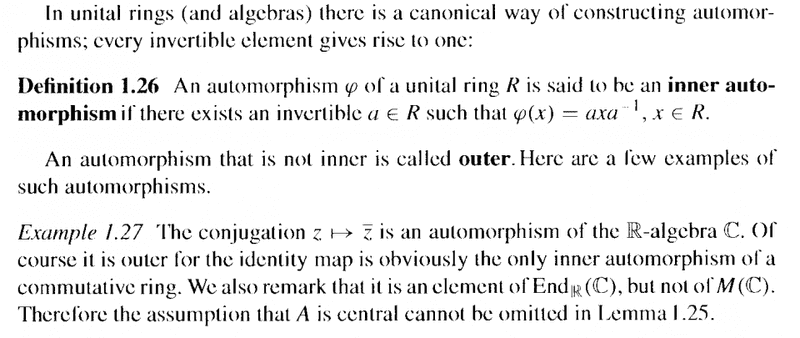
My questions on Example 1.27 comprise the following:
Question 1

In the above example from Bresar we read the following:

" ... ... Of course it is outer ... for the identity map is obviously the only inner automorphism of a commutative ring. ... ... "

I have two questions regarding this remark ...

(a) ... why does Bresar assert that "the identity map is obviously the only inner automorphism of a commutative ring" ... surely a commutative ring may have some units (invertible elements other than $1$ ...) and so may have some inner automorphisms ... BUT Bresar is asserting that this is not the case ... can someone please clarify this issue ...(b) ... Bresar seems to be talking about $\mathbb{C}$ ... but he is referring to "a commutative ring" in the quote above ... but why? ... $\mathbb{C}$ is a field ... ?

Can someone please clarify what Bresar means ...

Question 2

In the above example from Bresar we read the following:

" ... ... We also remark that it is an element of $\text{End}_\mathbb{R} ( \mathbb{C} )$ but not $M( \mathbb{C} )$. ... "I have two questions on this remark ... as follows ...(a) ... ... Bresar proved in Lemma 1.25 (see previous post) that for

$M(A) = \text{End}_F (A)$ ...

so ... why isn't it true that $M( \mathbb{C} ) = \text{End}_\mathbb{R} ( \mathbb{C} )$ ... ?(b) ... can someone please explain exactly why the conjugation automorphism is an element of $\text{End}_\mathbb{R} ( \mathbb{C} )$ but not of $M( \mathbb{C} )$ ... ?Help will be much appreciated ... ...

Peter=============================================================================

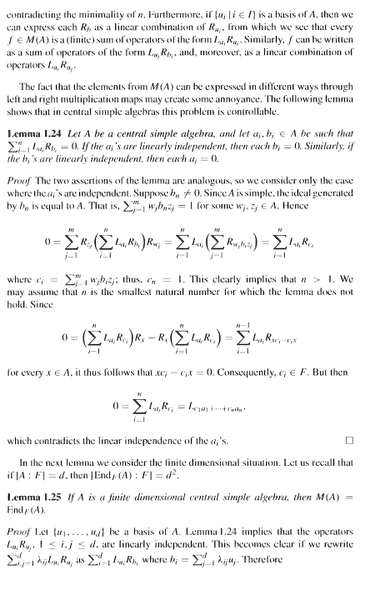
So that readers of this post can appreciate the notation and the context I am providing Bresar Section 1.5 ( which includes Lemmas 1.24 and 1.25) and also the start of Bresar Section 1.6 ... ... as follows:

 

[Delta2]

I think I can answer question 1 easily but mind that I am a bit rusty on abstract algebra.

if the ring is commutative then any inner automorphism is the identity since by definition of the inner automorphism $\phi(x)=axa^{-1}=aa^{-1}x=x$. The second step in the series of equalities is because the ring is commutative.

Also any field is a commutative ring (by the definition of the field if I am not mistaken) so I hope the above answer this question.

 

[zinq]

It is necessary to sit with pencil (or your favorite writing implement) and paper in order to understand the answers to questions like yours, Math Amateur.

M(C) is the multiplication algebra of C, generated by left and right multiplications of an element (call it z) of C, by other elements of C. First verify that conjugation is an endomorphism of the R-algebra C. (Here it is absolutely necessary to know what the terms conjugation, R, R-algebra, and C mean.) Then verify that no matter which left and right multiplications you use to get an element of M(C), you will never obtain complex conjugation.