Bar pivoted at end-conservation of energy

[natasha13100]

Homework Statement

This is actually a multi-part question, but I only need help on the following part right now.
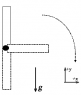
A uniform bar of mass m and length L is pivoted at one end and is held vertically as shown. After the bar is released, it swings downward with no friction in the pivot.
At the instant the bar is at the bottom of the swing, find the magnitudes of the angular velocity and angular acceleration.
I have no problem finding angular velocity. I use the following symbols:
t=torque
I_p=moment of inertia of the pivot
a=tangential acceleration
v=tangential velocity
α=angular acceleration
ω=angular velocity
K=kinetic energy
U=potential energy

Homework Equations

for sure:
I_p=1/3mL²
U_i+K_i=K_trans,f+K_rot,f+U_f
U_grav=mgh
K_trans=1/2mv²
K_rot=1/2Iω²
maybe:
t=rFsinθ
t=Iα
a=Rα
F=G+t
G=mg
I_cm=1/12mL²

The Attempt at a Solution

U_i=mgL/2
U_f=-mgL/2
K_i=0
K_trans,f=0
K_rot,f=1/2I_pω²=1/6mL²ω²
mgL=1/6mL²ω²
6g/L=ω²
ω=√(6g/L)
mg=I_pα
α=mg/I_p=mg/(1/3mL²)=3g/L²

 

[voko]

What makes you think that $mg = I_p \alpha$?

Secondly, at which point is the angular velocity maximal?

 

[natasha13100]

The only force acting on this system is gravity. I figured either I could use F=ma and t=Ia for the center of mass or t=Ia for the pivot. I'm probably not right.
I am not too sure when the angular velocity is maximal, but I would think it would be maximal at the bottom.

 

[voko]

The only force acting on this system is gravity. I figured either I could use F=ma and t=Ia for the center of mass or t=Ia for the pivot.

The latter formula is correct, assuming "t" stands for torque. But you used $I_p \alpha = mg$, and $mg$ is definitely not torque - it is a force!

I am not too sure when the angular velocity is maximal, but I would think it would be maximal at the bottom.

Think about it from the energy conservation standpoint.

 

[natasha13100]

Well kinetic energy is maximized when potential energy is minimized. I think angular acceleration increases up until the bar is horizontal because t=rFsin(theta) and sin(theta) increases from theta=90 to theta=0 (assuming the horizontal is theta=0). Then the angular acceleration decreases until the bar is in the downward position. That is why I though the angular velocity would be maximal when the bar was in the downward position. If angular velocity is maximal, angular acceleration must be 0.

 

[natasha13100]

conservation of energy:
if I use the pivot as the origin,
mgL/2=Iw^2+mgL/2*cos(theta)
Iw^2=mgL/2*(1-cos(theta))
w is maximized when cos(theta) is maximized which occurs when cos(theta)=-1 which is at the bottom

 

[voko]

I am a little confused by the angles. There was a mention of a diagram in the description, but non was provided. I am not sure about the initial configuration and how the angle is measured.

 

[natasha13100]

oops. I'll put the diagram in now.

 

[voko]

Thanks for the picture, but it is still unclear how $\theta$ is defined.

 

[natasha13100]

I ended up figuring it out on my own based on some thought (#5) and the conservation of energy ended up agreeing once I got my signs straight (#6). However, the second part is what's tripping me up. I thought it would be easy once I understood the first part, but I'm missing something. The linear speed of the free end of the bar I when the rod is in the downward position I found by v=w*R. However, I can't seem to understand how to get the horizontal and vertical components of the acceleration of the free end of the bar. a=R*alpha so I thought a=0. I really wish I could understand the theory behind angular and tangential acceleration. Do you have any tips?

 

[natasha13100]

theta is the angle between the horizontal line extending from the pivot and the rod with counterclockwise being positive and clockwise being negative

 

[voko]

Did you obtain ZERO angular acceleration in the downward position? It seems so. Then I am unsure why you think that zero tangential acceleration in that same position is problematic.

 

[natasha13100]

I did obtain zero angular acceleration, but zero acceleration at the free end of the rod when it is in the downward position was incorrect. The horizontal component was 0 but the vertical component is nonzero. I am guessing this is kind of like a pendulum where the string keeps going after reaching the bottom point of its swing because the problem is asking for acceleration not tangential acceleration. The tangential acceleration is 0 (I believe).

 

[voko]

Tangential acceleration is zero because angular acceleration is zero.

Normal acceleration is of course not zero, because the free end follows a circular trajectory. Since you know the velocity of the free end and the radius of the circle, finding the normal acceleration should be a simple matter.

 

[natasha13100]

Would I be able to consider this as uniform circular motion? I believe so because the force due to gravity doesn't make much difference near the bottom of the swing. In this case, it would simply be a=v^2/r

 

[voko]

How do you define uniform circular motion? Would the definition be satisfied at the bottom?

 

[natasha13100]

With uniform circular motion the tension in the string or in this case the force exerted by the pivot is the net force acting on the object. The magnitude of the velocity doesn't change but its direction does. Since gravity is negligible very close to the bottom of the swing, the rod can be considered as having uniform circular motion if I am not mistaken.

 

[voko]

Even though your conclusion is correct, and the logic can be sort of justified, it is not exactly what you should say. Uniform circular motion is circular motion with zero tangential acceleration. You do not need to think about gravity, tension or anything else. Circular and zero tangential acceleration, all it takes. And that is definitely the case at the bottom.

 

[natasha13100]

so out of curiousity, what would I have done if I was looking at the rod when it is horizontal? I can't say the angular acceleration is zero there. In fact, angular acceleration would be at its highest.
In that case mgL/2=Iw^2/2=mL^2w^2/6
g=Lw^2/3
w=sqrt(3g/L)
I would guess t=rfsin(theta)=mgL/2 and t=Ia=mL^2w^2(alpha)/6 so mL^2w^2(alpha)/6=mgL/2.
mLg/2(alpha)=mLg/2
alpha=1
or
alpha=v^2/R=w^2R^2/R=w^2R=3g/L*L=3g

 

[voko]

Normal acceleration is always given by the formula $v^2 / r$, for any motion, not necessarily uniform and not necessarily circular.

 

[natasha13100]

okay. thanks :)