- #1
nhrock3
- 415
- 0
i know the law (fg)'=f'g+fg'
but here there is epsilon
and it turns to someother sign i don't know what it says.
i can't understand the transition
?
There's no epsilon in what you posted. Are you talking about [itex]\prod[/itex]? Note that this is upper-case pi, which is different from the constant [itex]\pi[/itex], lower-case pi.nhrock3 said: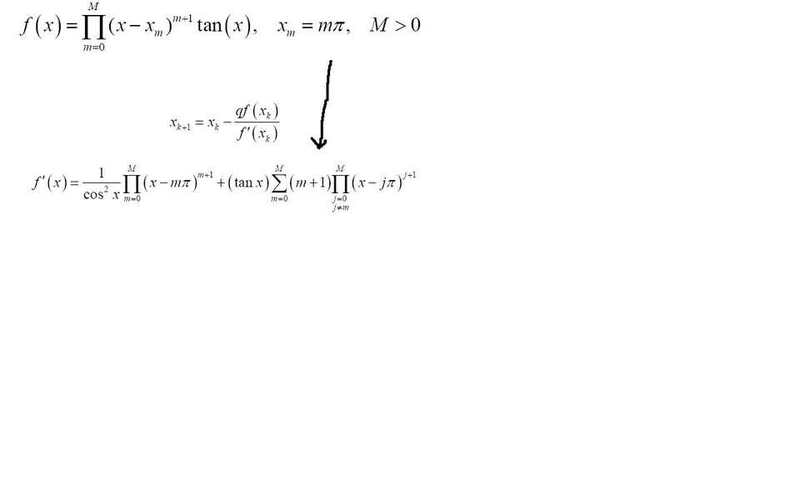
i know the law (fg)'=f'g+fg'
but here there is epsilon
and it turns to someother sign i don't know what it says.
i can't understand the transition
?
The basic derivative laws in numerical analysis include the power rule, product rule, quotient rule, chain rule, and the derivative of a constant. These laws are used to find the derivative of a function at a specific point.
The derivative laws in numerical analysis are used to approximate the derivative of a function at a specific point. This is useful in many applications, such as optimization problems or predicting the behavior of a system.
Yes, these laws can be used for all types of functions, including polynomial, exponential, logarithmic, and trigonometric functions. However, some functions may require additional techniques or approximations to find their derivatives.
Numerical methods for finding derivatives can introduce errors due to rounding and truncation. These errors can accumulate and affect the accuracy of the final result. It is important to use proper techniques and take into account the precision of the numbers being used to minimize these errors.
While these laws are powerful tools for finding derivatives, they do have some limitations. They may not work for all functions, and they may not provide an exact solution. Additionally, they may not be able to handle discontinuous functions or functions with sharp turns. In these cases, other numerical methods may be necessary.