Dividing by a parameter that is zero

[Dr_Pill]

Hi,

I'm studying calculus of variations, this is a bit of a recap of regular calculus.

You got a function, but you plug a vector that is parameterized so your function becomes parameterized, then you take the derivative in 3.5, and then they say: put the parameter to zero in 3.6, but then you divide by zero, I don't get it.

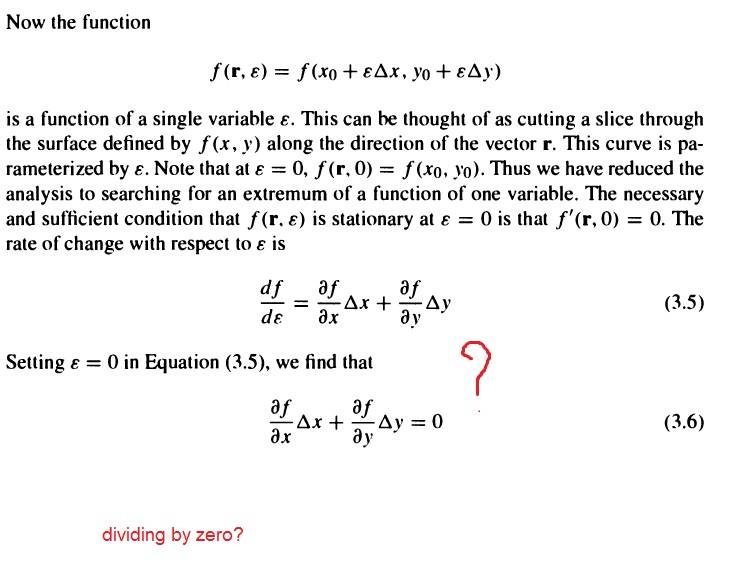
Cheers

 

[CompuChip]

Where are you dividing by $\varepsilon$ then?

You are just using the condition that
$$f'(\vec r, 0) = \left. \frac{df}{d\epsilon} \right|_{\epsilon = 0}$$
i.e. $\varepsilon = 0$ is a stationary point to set the left hand side to zero.

 

[verty]

It doesn't set dε to 0, it sets $\frac{df}{dε}$ to 0. The rate of change of f is 0 at ε = 0 because f is stationary at that point.

 

[HallsofIvy]

Are you under the impression that $\frac{df}{de}$ involves dividing by e? It does not.
$$\frac{df}{de}= \lim_{h\to 0}\frac{f(e+h)- f(e)}{h}$$

 

[Dr_Pill]

My god.What a mistake.

It's been a while since I've encountered calculus.
I better review the basics thoroughly than tackling some more advanced stuff.

Excuses!