Interior and Closure in a Topological Space .... .... remark by Willard

[Math Amateur]

TL;DR

I need help in order to prove a result stated by Willard linking the notions of interior and closure in a topological space ...

I am reading Stephen Willard: General Topology ... ... and am currently focused on Chapter 2: Topological Spaces and am currently focused on Section 3: Fundamental Concepts ... ...

I need help in order to fully understand a result or formula given by Willard concerning a link between closure and interior in a topological space ... ..The relevant text reads as follows:

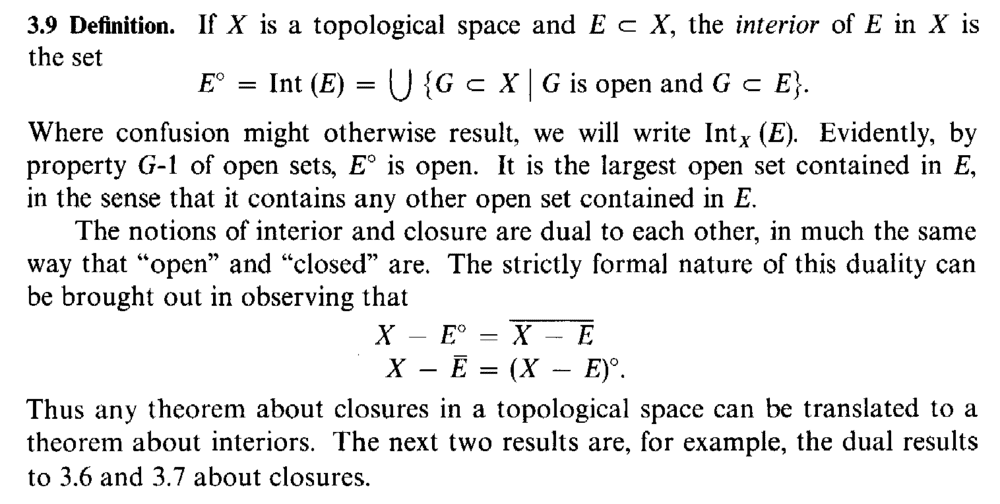
In the above text by Willard we read the following:

" ... ... The strictly formal nature of this duality can be brought out in observing that

$X - E^{ \circ } = \overline{ X - E }$ ... ... "Can someone please demonstrate (formally and rigorously) that ... given the definitions and results regarding closure and interior used by Willard ... $X - E^{ \circ } = \overline{ X - E }$ ... ...The definitions and results regarding closure used by Willard are as follows:

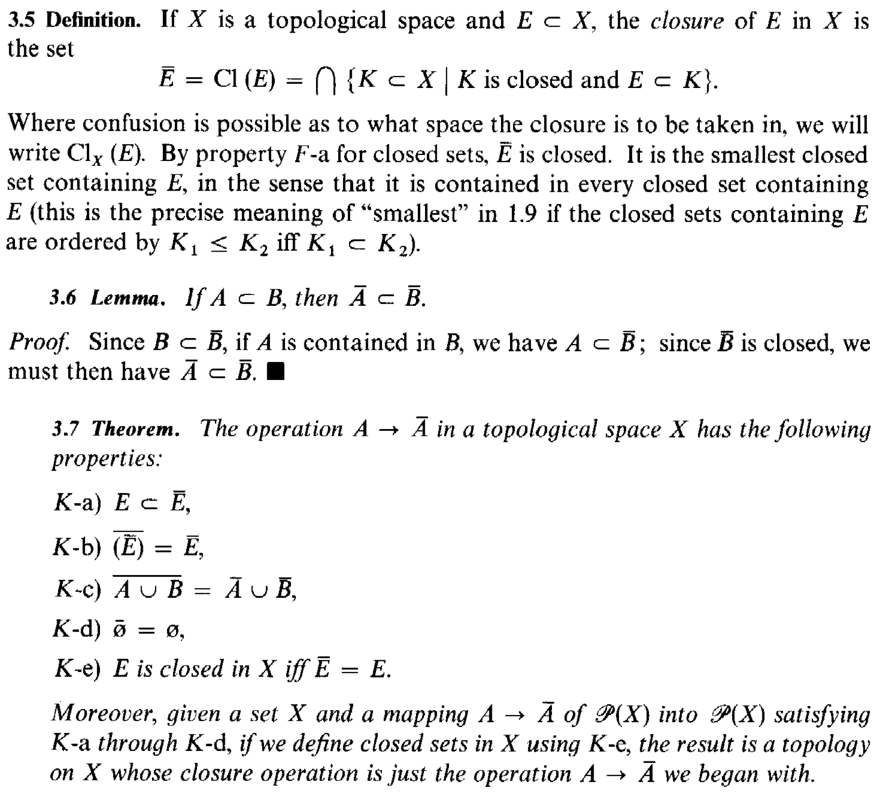
Help will be much appreciated ... ...

Peter

 

[Infrared]

Let's follow the definitions:
We have $p\in X-E^\circ$ if and only if every open set containing $p$ intersects $X-E$ nontrivially if and only if $p$ is in the closure of $X-E.$

The first biconditional is just the negation of your definition of interior. The second is the following fact: Let $X$ be a topological space and let $E\subset X$. Then $p\in \overline{E}$ if and only if every neighborhood of $p$ intersects $E$ nontrivially. Is this fact okay, or do you also want an argument here?

Edit: A little more direct argument using your definitions is:

$X-E^\circ=X-\bigcup_{G\subset E \ \text{open}} G=\bigcap_{G\subset E \text{ open}} (X-G)=\bigcap_{F\supset X-E \text{ closed}}F=\overline{X-E}.$

 

[Math Amateur]

Thanks so much Infrared ...

Working through your post just now ...

Very much appreciate your help ...

Peter