- #1
Amaelle
- 310
- 54
- Homework Statement
- look at the image
- Relevant Equations
- stockes theorem
Greetings
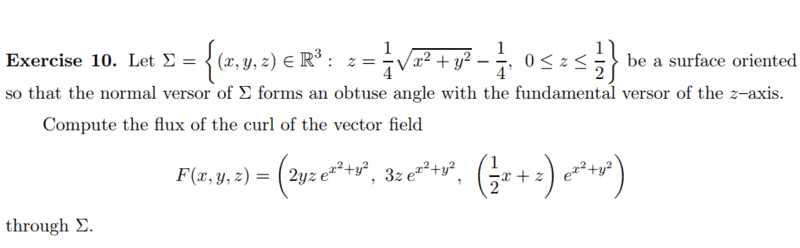
the solution is the following which I understand
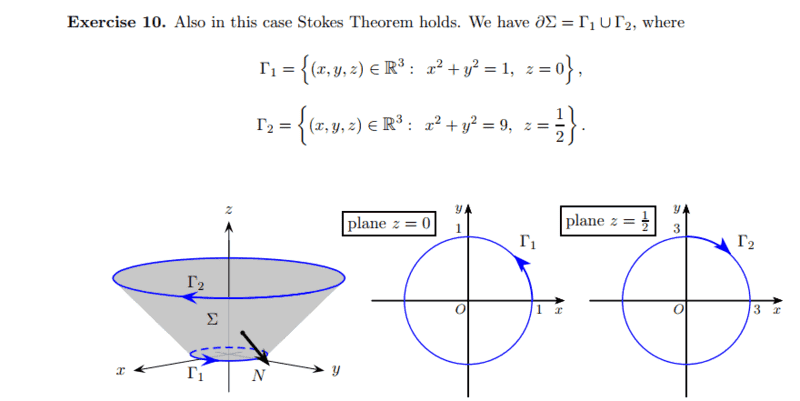
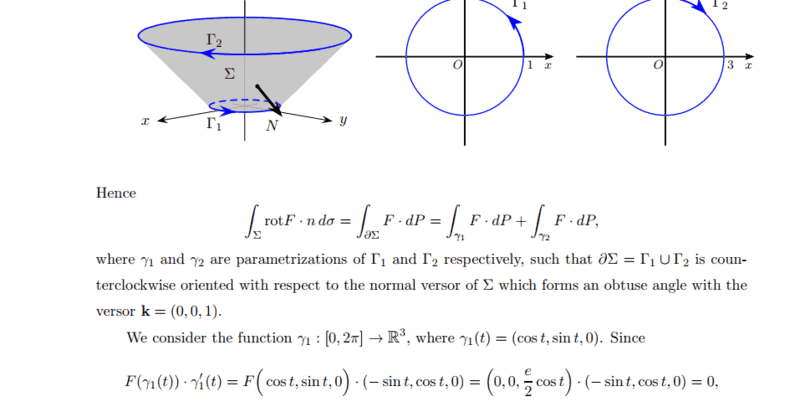
I do understand why the current orientation of the Path is positive regarding to stocks (the surface should remain to the left) but I don´t understand why the current N vector of the surface is positive regarding stockes theorem
thank you!
the solution is the following which I understand
I do understand why the current orientation of the Path is positive regarding to stocks (the surface should remain to the left) but I don´t understand why the current N vector of the surface is positive regarding stockes theorem
thank you!