Projectile Motion here then simulated in a different gravity

[Tomas1337]

So I'm working on an experiment where I do a projectile motion simulation here on Earth and want to know how it will fair in a low gravity environment such as in the moon with one common parameter in between, Force.

Imagine, here on earth, I have a mass that I push up with a force(F) for it to jump y meters. I record the mass, the height at which it reached and the time it took for it to reach that height which will then allow me to compute for my Force(F).

Now I want to know, if I used this same Force(F) on a different mass under a different gravity, how high it will reach?

I`ve come up so far with this.

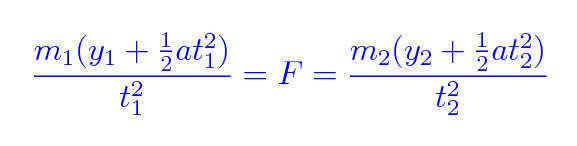
My problem is, when I do solve for the Force, I still have 2 missing parameters which is y and t which is preventing me from solving each variable. Any suggestions?

 

[A.T.]

Imagine, here on earth, I have a mass that I push up with a force(F) for it to jump y meters

Force alone is insufficient information. You need duration or distance of force application.

This thread might be relevant to you:
https://www.physicsforums.com/threa...ump-21-times-higher-than-on-the-earth.774140/

 

[Tomas1337]

Hi, A.T.!

Thank you for your reply and pointing the thread out to me. Learned a lot about the duration the force is applied. The only most important information to my experiment that helped me understand my problem was:

I think bahamagreen's comparision to a compressed spring would be an ok representation of the problem. The energy of the compressed spring $E_c$ must be spent on partly lifting an object with a mass while extending the spring $E_{ext}=mg\delta$ and partly on the objects flight $E_f=mgh$. The only difference between moon and Earth here are the value of g, beeing 6 times larger for earth. (I neglect the possible difference in $\delta$).
In other words we get:
$E_{f}=E_{c}-E_{ext}$
so from this you can show that the ratio of jumping heights are:

$\frac{h_m}{h_e}=\frac{g_e}{g_m} \frac{E_c-mg_m\delta}{E_c - mg_e\delta}$ (index m=moon, e=earth)

In reality, my experiment is not a finger pushing but a spring of some sort which will be attached inside the mass. The spring is this:

My theory is that the impulse of the spring when it `snaps` will pass on to the object which it is attached to and will then make the whole thing jump.

Any ideas on how I can proceed? The snap is instantaneous so I can`t (dont know how) to do record the duration on which the force acts on it`s joints...

 

[A.T.]

The snap is instantaneous

For a very short impulse, the take off velocity will be approximately the same in both cases. So the maximal height is proportional to g.

 

[Tomas1337]

I thought as much if it were the same mass yeah, any idea on how to factor in different masses of the objects? Using the equation above would require me to Solve for the Spring`s potential energy which in my case is... kind of hard since it`s not a conventional spring. Is there a more straightforward way?

 

[A.T.]

any idea on how to factor in different masses of the objects?

Take off velocity is inversely proportional to mass. The maximal height is proportional to velocity squared.

 

[Tomas1337]

Okay so, sorry i`m really having a hard time here.

Can you verify if this is correct? Based on what you said... And if it is, I still don`t get the derivation.

 

[A.T.]

I still don`t get the derivation.

Assuming the short impulse Δp is independent of m and g:

v = Δp / m
h = v² / (2*g) = Δp² / (2*g*m²)

 

[Tomas1337]

Got it!
I think I finally got it right.
Assuming impulse is the same for both cases and the mass and gravity do not affect impulse at all.

So the relation is*

Am I right?