Question about how to derive Sine

  • Context: Undergrad 
  • Thread starter Thread starter alex308
  • Start date Start date
  • Tags Tags
    Derive Sine
Click For Summary

Discussion Overview

The discussion revolves around the derivation and understanding of the sine function, particularly in the context of right triangles and the unit circle. Participants explore methods to find the sine of an angle, specifically using a 73° angle as an example, and seek to understand the underlying principles without relying on calculators.

Discussion Character

  • Exploratory
  • Technical explanation
  • Conceptual clarification
  • Debate/contested
  • Mathematical reasoning

Main Points Raised

  • One participant describes a method involving the slope of the hypotenuse and the point-slope formula to find sine values, questioning how to determine the slope for any angle.
  • Another participant provides the relationship between sine, cosine, and tangent using the unit circle, suggesting that sine and cosine are defined by the coordinates on the circle.
  • There is a discussion about the difficulty of deriving sine and cosine from first principles, with some participants asserting that these values are defined rather than derived.
  • One participant mentions that most sine values are not constructible numbers, suggesting a limitation in deriving exact values.
  • Another participant proposes using circle construction to prove the sine addition identity as a more useful approach.
  • Several participants discuss the possibility of approximating sine values, with suggestions to use calculus or power series expansions for this purpose.

Areas of Agreement / Disagreement

Participants express differing views on the feasibility of deriving sine values exactly, with some asserting that it is not possible for most angles, while others explore methods for approximation. The discussion remains unresolved regarding the best approach to derive or approximate sine values.

Contextual Notes

Participants note that the x and y coordinates on the unit circle are definitions of sine and cosine, which may limit the ability to derive these values from first principles. There is also mention of the challenge posed by non-constructible numbers in finding exact sine values.

Who May Find This Useful

This discussion may be useful for students and individuals interested in the foundational concepts of trigonometry, those seeking to understand the derivation of trigonometric functions, and anyone looking for methods to approximate sine values without a calculator.

alex308
Messages
3
Reaction score
0
So I know that sine of an angle is the side opposite of the angle in a right triangle divided by the hypotenuse. What i want to know is: if i have an angle in a right triangle how do i find the sides of the triangle so i can find Sine.

I spent my study hall trying to figure it out and this is as far as i got. I'm simply using a random angle of 73° to depict my logic.

Sine.jpg


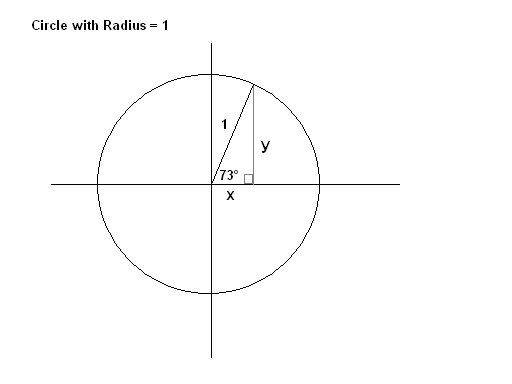
I figured out that if i could just find the slope of the hypotenuse, i would be able to use point-slope formula to find an equation for the hypotenuse. I could then determine where the hypotenuse intersects the circle which would give me the values of X and Y. I would then be able to calculate the Sine of the angle.

I suppose it all boils down to how do i find the slope of the hypotenuse of a right triangle.
The only one i could figure out was that 45° has a slope of 1 and as theta approaches 90° the slope approaches infinite.

I don't know if this is the original method of deriving Sine but it seems like it could work if i could find the slope of any angle. My intention is to find all the Y values on the circle so that i can plot a sine wave. If anyone can lead me in the right direction with my work or if they could tell me a more logical way of finding sine i would appreciate it.
 
Mathematics news on Phys.org
x=r*cos(theta)
y=r*sin(theta)

slope=y/x=tan(theta)=sin(theta)/cos(theta)

does that help, or are you trying to derive this from simpler methods?
 
I'm not sure what you mean by "derive" The x and y coordinates on the unit circle are the definition respectively of the cosine and sine functions of the angle.

You can then either define or derive that the slope of the hypotenuse is the tangent of the angle and vis versa that it is the ratio of sine over cosine.

I tell my students to keep straight which is which to remember to keep them in alphabetical order x =cosine, y = sine.

So you can't really "derive" sine and cosine from first principles because that they are the coordinates is the definition i.e. is the "first principles" from which we derive other relations.
 
You could spend days doing this and not get very far. The problem is that most values of the sine function are not constructable numbers.

What you can do is something a bit more useful. Try to use your circle construction to prove the identity:

\sin(a + b) = \sin a \cos b + \cos a \sin b
 
jambaugh said:
I'm not sure what you mean by "derive" The x and y coordinates on the unit circle are the definition respectively of the cosine and sine functions of the angle.

You can then either define or derive that the slope of the hypotenuse is the tangent of the angle and vis versa that it is the ratio of sine over cosine.

I realize that the x and y cordinates are the definitions of cosine and sine. My quarrel is how do i determine the x and y values.

What I meant by derive was how do i come up with the x and y values for this triangle and for all rational degrees around the circle so i can sketch a sine wave.

This is all in an attempt to understand what a calculator is doing when i graph sin(x) or even where it comes up with an answer for sin(73).

If anybody could walk me step be step through finding the sine of 73° without a calculator i would appreciate it.

I know that if someone could just show me how to find the SLOPE of the line that forms the angle between it and then the x-axis then i would have the answer to my question.
 
Unless, you are willing to accept an approximate value, the answer, as you have been told several times now, is that you can't- almost all trig values are NOT "constructible numbers".
 
HallsofIvy said:
Unless, you are willing to accept an approximate value, the answer, as you have been told several times now, is that you can't- almost all trig values are NOT "constructible numbers".

Well that is all well and good but then please explain to me how I could approximate these values.
 
Do you know calculus? That is the best way to obtain approximate values.
 

Similar threads

Undergrad Geometry problem of interest with a 3-4-5 triangle
  • · Replies 59 ·
2
Replies
59
Views
230K
MHB How can I find the area of a triangle with a given angle and two sides?
  • · Replies 2 ·
Replies
2
Views
1K
High School Is the sine function defined at pi/2?
  • · Replies 9 ·
Replies
9
Views
2K
Undergrad Can the same argument be used for both radians and degrees in the sine function?
  • · Replies 3 ·
Replies
3
Views
2K
Undergrad Possible simple formula for ellipse circumference
  • · Replies 6 ·
Replies
6
Views
3K
High School Deriving law of sines from cross product
  • · Replies 4 ·
Replies
4
Views
5K
Undergrad How would one calculate the distance and bearing of a signal source?
  • · Replies 7 ·
Replies
7
Views
3K
High School Cosine or Sine of (angle+angle) always equal to 1?
  • · Replies 4 ·
Replies
4
Views
2K
MHB Find the height and base of a trapezium
  • · Replies 1 ·
Replies
1
Views
2K
Undergrad How was the Sine function programmed? The actual equation?
  • · Replies 6 ·
Replies
6
Views
4K