- #1
unscientific
- 1,734
- 13
Taken from Hobson's book:
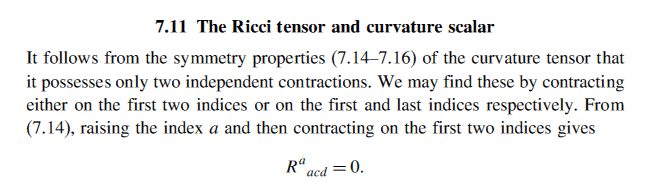
How is this done? Starting from:
[tex] R_{abcd} = -R_{bacd}[/tex]
Apply ##g^{aa}## followed by ##g^{ab}##
[tex]g^{aa}g^{aa} R_{abcd} = -g^{ab}g^{aa}R_{bacd}[/tex]
[tex]g^{ab}R^a_{bcd} = -g^{ab}g^{aa}R_{bacd} [/tex]
[tex]R^{aa}_{cd} = - g^{ab}g^{aa} R_{bacd} [/tex]
Applying ##g_{aa}## to both sides:
[tex]g_{aa}R^{aa}_{cd} = - g^{ab}g^{aa} g_{aa} R_{bacd} [/tex]
[tex] R^a_{acd} = -g^{ab} R_{bacd} [/tex]
[tex] R^a_{acd} = - R^a_{acd} = 0 [/tex]
Is there a quicker way?
How is this done? Starting from:
[tex] R_{abcd} = -R_{bacd}[/tex]
Apply ##g^{aa}## followed by ##g^{ab}##
[tex]g^{aa}g^{aa} R_{abcd} = -g^{ab}g^{aa}R_{bacd}[/tex]
[tex]g^{ab}R^a_{bcd} = -g^{ab}g^{aa}R_{bacd} [/tex]
[tex]R^{aa}_{cd} = - g^{ab}g^{aa} R_{bacd} [/tex]
Applying ##g_{aa}## to both sides:
[tex]g_{aa}R^{aa}_{cd} = - g^{ab}g^{aa} g_{aa} R_{bacd} [/tex]
[tex] R^a_{acd} = -g^{ab} R_{bacd} [/tex]
[tex] R^a_{acd} = - R^a_{acd} = 0 [/tex]
Is there a quicker way?
Last edited: