- #1
mrjohns
- 13
- 0
I'm studying the hydrogen atom and have this question. Apparently it can be solved without perturbation theory, however I'm having trouble justifying it.
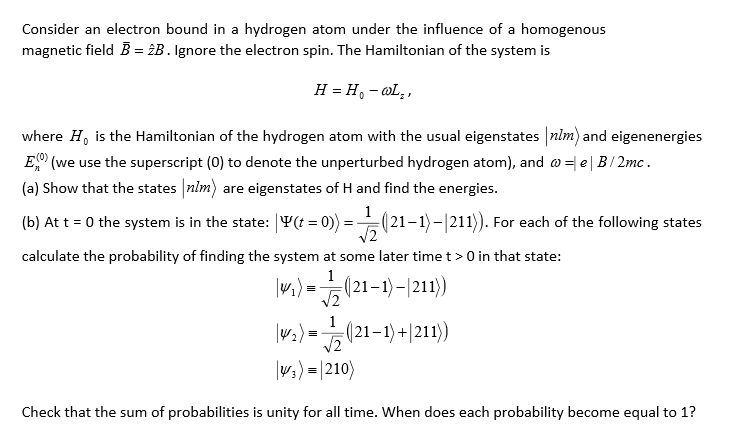
2. The attempt at a solution
Avoiding perturbation theory I simply get:
E = E(n) - constant*(mh) where m is the angular momentum number not mass
Which seems a little too easy.
For the second part I can see the initial t=0 wavefunction is normalised no problem, but when I apply the time evolution operator e^(iEt)/h things get messy.
Am I right in thinking the probability for the third state | 2 1 0 > is just zero for all time?
Homework Statement
2. The attempt at a solution
Avoiding perturbation theory I simply get:
E = E(n) - constant*(mh) where m is the angular momentum number not mass
Which seems a little too easy.
For the second part I can see the initial t=0 wavefunction is normalised no problem, but when I apply the time evolution operator e^(iEt)/h things get messy.
Am I right in thinking the probability for the third state | 2 1 0 > is just zero for all time?