- #1
etotheipi
- Homework Statement
- Calculating work done during adiabatic compression (please see problem statement below)
- Relevant Equations
- Ideal gas laws, internal energy
I'm having a little trouble with part a) of this question:
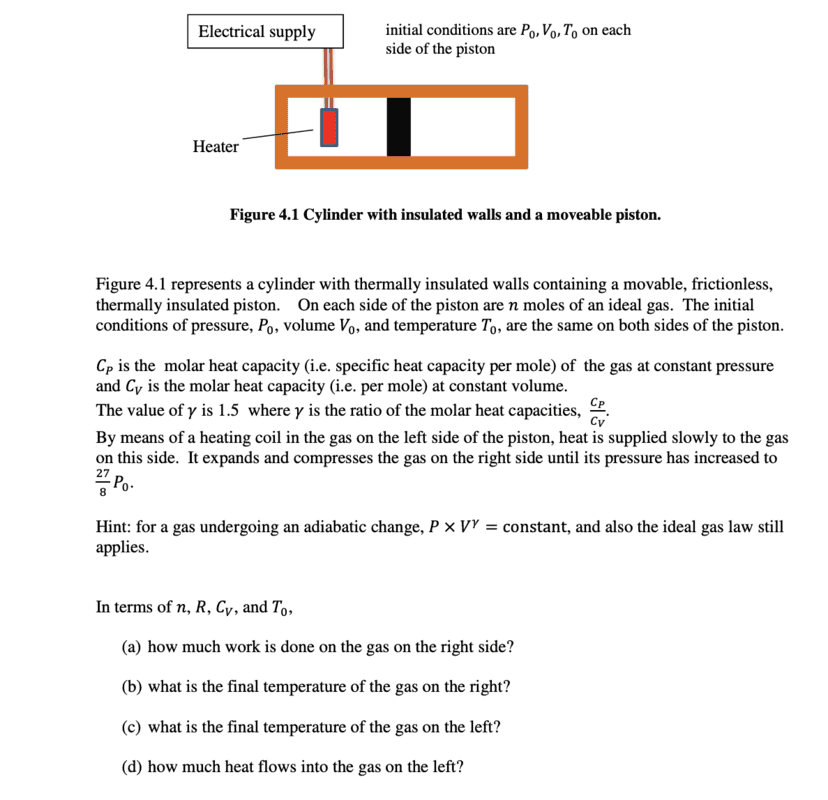
Since it is stated that the heating is slow, I thought it was reasonable to assume the process is reversible which means that the pressure in both sides should be equal. Consequently, $$W = - \int_{V_{0}}^{V_{1}} P dV = - \int_{V_{0}}^{V_{1}} kV^{- \gamma} dV = \frac{1}{\gamma-1}(P_{1}V_{1} - P_{0}V_{0})$$ For ##\gamma = 1.5##, the outside coefficient is ##2##.
However, if I use the first law (with ##Q=0## due to the insulated/adiabatic condition), I get $$W = \Delta U = \frac{3}{2}(nRT_{1} - nRT_{0}) = \frac{3}{2}(P_{1}V_{1} - P_{0}V_{0})$$I can't figure out why the two coefficients of ##(P_{1}V_{1} - P_{0}V_{0})## are different!
After this is sorted out, I assume it will just be a case of doing some rearrangement (i.e. w/ ##\frac{P_{0}V_{0}}{T_{0}} = \frac{P_{1}V_{1}}{T_{1}}## etc.) to get it into the required form?
Since it is stated that the heating is slow, I thought it was reasonable to assume the process is reversible which means that the pressure in both sides should be equal. Consequently, $$W = - \int_{V_{0}}^{V_{1}} P dV = - \int_{V_{0}}^{V_{1}} kV^{- \gamma} dV = \frac{1}{\gamma-1}(P_{1}V_{1} - P_{0}V_{0})$$ For ##\gamma = 1.5##, the outside coefficient is ##2##.
However, if I use the first law (with ##Q=0## due to the insulated/adiabatic condition), I get $$W = \Delta U = \frac{3}{2}(nRT_{1} - nRT_{0}) = \frac{3}{2}(P_{1}V_{1} - P_{0}V_{0})$$I can't figure out why the two coefficients of ##(P_{1}V_{1} - P_{0}V_{0})## are different!
After this is sorted out, I assume it will just be a case of doing some rearrangement (i.e. w/ ##\frac{P_{0}V_{0}}{T_{0}} = \frac{P_{1}V_{1}}{T_{1}}## etc.) to get it into the required form?
Last edited by a moderator: