- #1
Waxterzz
- 82
- 0
For a regular LR circuit (L and R in series) and with a AC voltage:
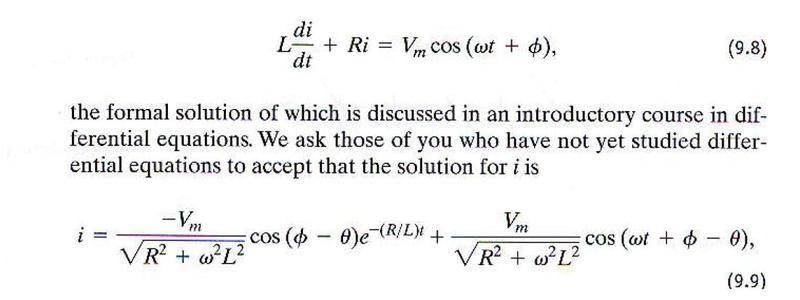
I tried to derive the solution myself.
https://www.dropbox.com/s/jmsu9j0vt91ze8x/LRcircuit.jpg
So first I solved with undetermined coefficients, plugged them in, and then solved with Cramer's Rule.
Then I added the term (the solution for the homogeneous case) with the solution I got with undertermined coefficients.
Then I used initial value: the current i(t) at t=0 is 0.
Then I got the general solution: an exponential term, a cosine term and a sine term. But my solution is quite different from the book.
Can anyone help me?
I tried to derive the solution myself.
https://www.dropbox.com/s/jmsu9j0vt91ze8x/LRcircuit.jpg
So first I solved with undetermined coefficients, plugged them in, and then solved with Cramer's Rule.
Then I added the term (the solution for the homogeneous case) with the solution I got with undertermined coefficients.
Then I used initial value: the current i(t) at t=0 is 0.
Then I got the general solution: an exponential term, a cosine term and a sine term. But my solution is quite different from the book.
Can anyone help me?