A question about Alice and Bob in SR

[MathematicalPhysicist]

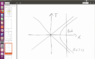
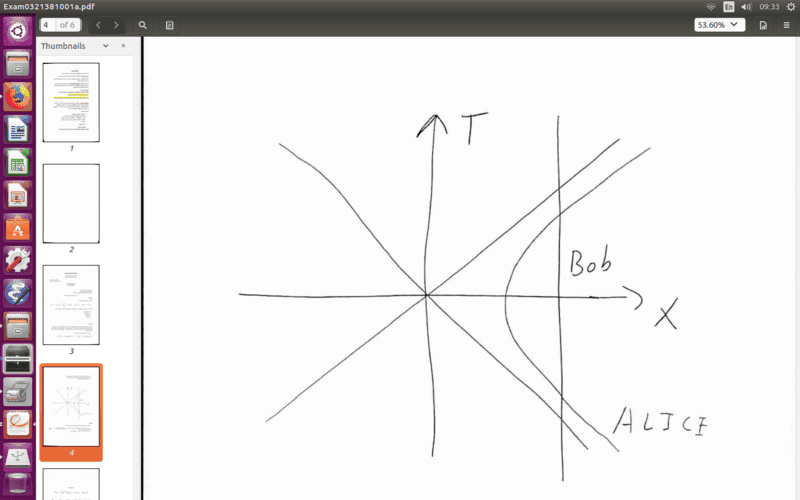
Bob is an observer at rest in Minkowski space at $x=L$.
Alice moves in a constant acceleration (in her system).
her path is depicted in the attached file, when Alice passes by Bob they synchronize their watches and Bob activates an apparatus that signals pulses to Alice.
Every short time period $\Delta t$ (which is calculated in Bob's system) a pulse is ejected.

The path of Alice is described by $X(\tau)=\frac{1}{a}\cosh(a\tau)$, $T(\tau)=\frac{1}{a}\sinh(a\tau)$.

I have a few questions:
1. What does Alice's watch show when they meet again?
2. What does Bob's watch show when they meet again?
3. How many pulses arrive to Alice?

My wrong answers are as follow:
1.We take $c=1$, so $\tau=\Delta t \cdot \gamma \cdot 2$, $v= a\Delta t \cdot 2$; so $\tau = 2\Delta t \frac{1}{\sqrt{1-(a\Delta t)^2\cdot 4}}$; so the watch of Alice will show the time:
$$T(\tau)= 1/a \cdot \sinh(\frac{2a\Delta t}{\sqrt{1-(a\Delta t)^2\cdot 4}}$$
2. I wrote that Bob's watch will show $2\Delta t$, but it's wrong.
3. The number of pulses arriving to Alice are $N=T(\tau)/\Delta t$, where $T(\tau)$ is the same as I got in question 1.

I will appreciate it

if you will guide me how to answer these questions correctly, thanks!

 

[Ibix]

$\tau$ is Alice's proper time - the time measured on her wristwatch. $t$ is the coordinate time in Bob's rest frame, so corresponds to Bob's proper time. So all you need to do is work out the $\tau$ and $t$ values at the two times they meet and you have everything you need.

 

[Orodruin]

Alice moves in a constant acceleration (in her system).

I assume that by this you mean to say that Alice has constant proper acceleration.

1.We take $c=1$, so $\tau=\Delta t \cdot \gamma \cdot 2$, $v= a\Delta t \cdot 2$; so $\tau = 2\Delta t \frac{1}{\sqrt{1-(a\Delta t)^2\cdot 4}}$; so the watch of Alice will show the time:
$$T(\tau)= 1/a \cdot \sinh(\frac{2a\Delta t}{\sqrt{1-(a\Delta t)^2\cdot 4}}$$

I am not sure what you are doing here. It is unclear how you relate the pulse to the time passed. Are you computing the time passed between pulses or the time passed between two simultaneities according to Bob?

The easier approach is to note that $\tau$ is the proper time of Alice. Solve for the meeting events and use that the time coordinate is Bob's proper time.

Hint: The relation $\sinh(\cosh^{-1}(x)) = \sqrt{x^2 - 1}$ might come in handy ...

2. I wrote that Bob's watch will show $2\Delta t$, but it's wrong.

Again, you need to be careful and more specific about what $\Delta t$ is. It is not clear from your description.

 

[MathematicalPhysicist]

I assume that by this you mean to say that Alice has constant proper acceleration.

Yes.

I am not sure what you are doing here. It is unclear how you relate the pulse to the time passed. Are you computing the time passed between pulses or the time passed between two simultaneities according to Bob?

The easier approach is to note that $\tau$ is the proper time of Alice. Solve for the meeting events and use that the time coordinate is Bob's proper time.

Hint: The relation $\sinh(\cosh^{-1}(x)) = \sqrt{x^2 - 1}$ might come in handy ...

Do you mean something like this: $1/a \sinh (a\tau)=\tau$?

Again, you need to be careful and more specific about what $\Delta t$ is. It is not clear from your description.

Bob is sending pulses to Alice in constant increments of time $\Delta t$.

 

[MathematicalPhysicist]

$\tau$ is Alice's proper time - the time measured on her wristwatch. $t$ is the coordinate time in Bob's rest frame, so corresponds to Bob's proper time. So all you need to do is work out the $\tau$ and $t$ values at the two times they meet and you have everything you need.

So I need to equate: $X(\tau)=at^2/2$, and then I need to salvage $\tau$ from this equation, am I correct?

 

[Orodruin]

Yes.



Do you mean something like this: $1/a \sinh (a\tau)=\tau$?

Bob is sending pulses to Alice in constant increments of time $\Delta t$.

Again, it is not clear from your description what these light pulses have to do with the computation of the proper times. (They don't, you could use them but you need to be much more careful and it will become much more messy than just using that $\tau$ is the proper time of Alice and $t$ the proper time of Bob.)

$(1/a)\sinh(a\tau) = \tau$ makes no sense. You need to find the events on the world line $x = L$ that intersect the world line of Alice. The difference in $\tau$ between those events is the proper time elapsed for Alice, the difference in $t$ between those events is the proper time elapsed for Bob.

 

[MathematicalPhysicist]

@Orodruin , so I need to find $X(\tau)=L$ and then plug the $\tau$ I find from this equation into $T(\tau)$, and the difference I find, i.e. $T(\tau)-\tau$ is the time elapsed in Alice's frame of reference.

Is Bob's duration of time should be $(T(\tau)-\tau)/\gamma$ or is it $(T(\tau)-\tau)\cdot \gamma$?

And $\gamma = \frac{1}{\sqrt{1-(a\tau)^2}}$ in units of $c=1$.

 

[Orodruin]

You should not be using $\gamma$ at all. Bob's duration is the difference in the time coordinate, nothing else.

 

[MathematicalPhysicist]

You should not be using $\gamma$ at all. Bob's duration is the difference in the time coordinate, nothing else.

So it should be $\Delta t$.

 

[Orodruin]

So it should be $\Delta t$.

What do you mean by $\Delta t$ now? Previously you were using in connection to some light signals and it was unclear what you meant by it. In general, yes the proper time of Bob is the difference in coordinate time (in Bob's rest frame) between the two events.

 

[MathematicalPhysicist]

When Bob meets Alice for the first time he then initiates a continuous stream of pulses which are ejected from him towards Alice in increments of time of $\Delta t$.

 

[Orodruin]

When Bob meets Alice for the first time he then initiates a continuous stream of pulses which are ejected from him towards Alice in increments of time of $\Delta t$.

Then that has absolutely nothing to do with the time computed by Bob. I suggest you forget about those light pulses. They are completely unnecessary to compute the elapsed proper time.

 

[MathematicalPhysicist]

Then that has absolutely nothing to do with the time computed by Bob. I suggest you forget about those light pulses. They are completely unnecessary to compute the elapsed proper time.

You wrote: "the difference in $t$ between those events is the proper time elapsed for Bob."
But how to calculate this difference then?

 

[Orodruin]

You wrote: "the difference in $t$ between those events is the proper time elapsed for Bob."
But how to calculate this difference then?

The difference between the $t$ coordinates of those events. They have nothing to do with any $\Delta t$ artificially imposed by sending out light signals. They only depend on the solutions to the world line intersections.

 

[MathematicalPhysicist]

The difference between the $t$ coordinates of those events. They have nothing to do with any $\Delta t$ artificially imposed by sending out light signals. They only depend on the solutions to the world line intersections.

So the difference in Bob's proper time should be: $t=L/(a\tau)$, am I correct?

And for the last question, of how many pulses did Alice receive?

I just need to compute $(T(\tau)-\tau)/\Delta t$, or is it something else?

 

[stevendaryl]

You wrote: "the difference in $t$ between those events is the proper time elapsed for Bob."
But how to calculate this difference then?

You have the two equations:

$x = \frac{1}{a} cosh(a \tau)$
$t = \frac{1}{a} sinh(a \tau)$

Put them together using the fact that $cosh^2 - sinh^2 = 1$:

$x^2 - t^2 = \frac{1}{a^2}$

So that allows you to compute Bob's time, $t$ for when Alice reaches the point $x$.

$t = \pm \sqrt{x^2 - \frac{1}{a^2}}$

The two times that Alice meets Bob are the times when $x=L$. So those two times are:

$t = \pm \sqrt{L^2 - \frac{1}{a^2}}$

The minus sign corresponds to the first time they meet, and the plus sign corresponds to the second time. So you don't need $\tau$ to compute $\Delta t$. Just subtract the two.

 

[m4r35n357]

If Alice moves with constant proper acceleration how can they meet a second time?

 

[MathematicalPhysicist]

You have the two equations:

$x = \frac{1}{a} cosh(a \tau)$
$t = \frac{1}{a} sinh(a \tau)$

Put them together using the fact that $cosh^2 - sinh^2 = 1$:

$x^2 - t^2 = \frac{1}{a^2}$

So that allows you to compute Bob's time, $t$ for when Alice reaches the point $x$.

$t = \pm \sqrt{x^2 - \frac{1}{a^2}}$

The two times that Alice meets Bob are the times when $x=L$. So those two times are:

$t = \pm \sqrt{L^2 - \frac{1}{a^2}}$

The minus sign corresponds to the first time they meet, and the plus sign corresponds to the second time. So you don't need $\tau$ to compute $\Delta t$. Just subtract the two.

So Just to recapitulate the answers:
1. Alice's watch shows: $T(\tau)-\tau = \frac{1}{a}\sqrt{(aL)^2-1}-\frac{1}{a}\cosh^{-1}(aL)$.
2. Bob's watch shows: $2\sqrt{L^2-1/a^2}$.
3. I think Alice's receives $(T(\tau)-\tau)/\Delta t$, am I wrong?

 

[stevendaryl]

So Just to recapitulate the answers:
1. Alice's watch shows: $T(\tau)-\tau = \frac{1}{a}\sqrt{(aL)^2-1}-\frac{1}{a}\cosh^{-1}(aL)$.
2. Bob's watch shows: $2\sqrt{L^2-1/a^2}$.
3. I think Alice's receives $(T(\tau)-\tau)/\Delta t$, am I wrong?

I don't know where you're getting #1. The time $\tau$ on Alice's watch when she is at location $x$ is simply $\tau = \pm \frac{1}{a} cosh^{-1}(ax)$. So the two times when she meets Bob are:

$\tau = \pm \frac{1}{a} cosh^{-1}(aL)$

So for Alice, the time between their meetings is just:

$2 \frac{1}{a} cosh^{-1}(aL)$

For Bob, the time between meetings is given by your #2.

The number of pulses that Alice receives between those times are equal to the number of pulses Bob sends, because she receives every one of them.

 

[MathematicalPhysicist]

I don't know where you're getting #1. The time $\tau$ on Alice's watch when she is at location $x$ is simply $\tau = \pm \frac{1}{a} cosh^{-1}(ax)$. So the two times when she meets Bob are:

$\tau = \pm \frac{1}{a} sinh^{-1}(aL)$

So for Alice, the time between their meetings is just:

$2 \frac{1}{a} sinh^{-1}(aL)$

For Bob, the time between meetings is given by your #2.

The number of pulses that Alice receives between those times are equal to the number of pulses Bob sends, because she receives every one of them.

I thought that I need to equate: $X(\tau)=L$ and then insert the tau that I find into $T(\tau)$, and then the difference between $T(\tau)-\tau$ is the time that elapsed in Alice's frame, so you say that it's wrong?

 

[stevendaryl]

I thought that I need to equate: $X(\tau)=L$ and then insert the tau that I find into $T(\tau)$, and then the difference between $T(\tau)-\tau$ is the time that elapsed in Alice's frame, so you say that it's wrong?

Let's review: Bob is using an inertial coordinate system $x, t$. Alice is traveling in such a way that if her clock shows time $\tau$, then her coordinates (according to Bob) are given by:

$x = \frac{1}{a} cosh(a \tau)$
$t = \frac{1}{a} sinh(a \tau)$

So it follows that when Alice's x-coordinate is $L$, then her clock must show a time $\tau$ such that:

$L = \frac{1}{a} cosh(a \tau)$

So $\tau = \pm \frac{1}{a} cosh^{-1}(aL)$

That's it. That tells the times $\tau$ at which Alice meets Bob.

[edit]

You're exactly right that you use $X(\tau) = L$. That implies that $\tau = \pm \frac{1}{a} cosh^{-1}(aL)$, as I said.

Your other expression, $T(\tau) - \tau$ doesn't make any sense. $T(\tau)$ is Bob's time, and $\tau$ is Alice's time. Subtracting them doesn't make any sense. To find out the elapsed time for Bob, you just use: $T(\tau_2) - T(\tau_1)$ where $\tau_1$ is Alice's time for the first meeting and $\tau_2$ is Alice's time for the second meeting. But you already computed that in a previous post: Bob's elapsed time is $2 \sqrt{L^2 - \frac{1}{a^2}}$

 

[m4r35n357]

That's it. That tells the times $\tau$ at which Alice meets Bob.

re. post #17, is there any physical interpretation of the negative time solution in this case, coz I can't think of one?

 

[stevendaryl]

re. post #17, is there any physical interpretation of the negative time solution in this case, coz I can't think on one?

Look, at some point, your clock says $t=0$. That means that earlier times had $t < 0$. and later times will have $t > 0$.

So a negative value of time just means a time that was earlier than the moment $t=0$.

If Alice is traveling so that $x = \frac{1}{a} cosh(a \tau)$, and Bob is traveling so that $x = L$, then when $\tau=0$, the distance between Alice and Bob is $L - \frac{1}{a}$.

So the original equations set it so that Alice meets Bob once earlier than $\tau=0$ and once later than $\tau = 0$.

 

[m4r35n357]

So the original equations set it so that Alice meets Bob once earlier than $\tau=0$ and once later than $\tau = 0$.

I am referring the diagram in the OP, which appears to show Alice approaching from x -> - infinity, meeting Bob as some coordinate (Bob) time, say 0, then receding again to x -> infinity. One meeting.

So either you are misinterpreting the diagram, or I am misinterpreting the equations ;)

[EDIT] just realized I am misinterpreting the diagram - pardon me!

 

[MathematicalPhysicist]

Let's review: Bob is using an inertial coordinate system $x, t$. Alice is traveling in such a way that if her clock shows time $\tau$, then her coordinates (according to Bob) are given by:

$x = \frac{1}{a} cosh(a \tau)$
$t = \frac{1}{a} sinh(a \tau)$

So it follows that when Alice's x-coordinate is $L$, then her clock must show a time $\tau$ such that:

$L = \frac{1}{a} cosh(a \tau)$

So $\tau = \pm \frac{1}{a} cosh^{-1}(aL)$

That's it. That tells the times $\tau$ at which Alice meets Bob.

[edit]

You're exactly right that you use $X(\tau) = L$. That implies that $\tau = \pm \frac{1}{a} cosh^{-1}(aL)$, as I said.

Your other expression, $T(\tau) - \tau$ doesn't make any sense. $T(\tau)$ is Bob's time, and $\tau$ is Alice's time. Subtracting them doesn't make any sense. To find out the elapsed time for Bob, you just use: $T(\tau_2) - T(\tau_1)$ where $\tau_1$ is Alice's time for the first meeting and $\tau_2$ is Alice's time for the second meeting. But you already computed that in a previous post: Bob's elapsed time is $2 \sqrt{L^2 - \frac{1}{a^2}}$

So so the number of pulses she receives are: $2/(a\Delta t)\cosh^{-1}(aL)$, is this correct?

 

[Ibix]

So so the number of pulses she receives are: $2/(a\Delta t)\cosh^{-1}(aL)$, is this correct?

No. How many pulses does Bob send? Could Alice not receive a pulse? Could she receive a pulse Bob didn't send? So how many pulses does she receive?

 

[MathematicalPhysicist]

@Ibix so she receives zero pulses?

Because none of the pulses he sends arrive to her.

 

[Ibix]

@Ibix so she receives zero pulses?

Because none of the pulses he sends arrive to her.

I'm confused. What do you think would stop them arriving?

 

[MathematicalPhysicist]

I don't see how to compute the number of pulses she receives, I thought it should be the time between their occurrences of they meetings, divided by the time interval $\Delta t$.

 

[Ibix]

I don't see how to compute the number of pulses she receives, I thought it should be the time between their occurrences of they meetings, divided by the time interval $\Delta t$.

It is. But whose time interval? Alice and Bob don't agree on the duration.

When answering the question, think whose clock is responsible for measuring $\Delta t$.

 

[MathematicalPhysicist]

It is. But whose time interval? Alice and Bob don't agree on the duration.

When answering the question, think whose clock is responsible for measuring $\Delta t$.

Bob's, because $\Delta t$ is according to his clock.

So if I am not mistaken the number of pulses arriving to Alice are: $(2/a\Delta t)\sqrt{L^2-1/a^2}$.

 

[Ibix]

Bob's, because $\Delta t$ is according to his clock.

So if I am not mistaken the number of pulses arriving to Alice are: $(2/a\Delta t)\sqrt{L^2-1/a^2}$.

Exactly - the same number as emitted.