- #1
Nitram
- 7
- 0
Hi All,
I've been going through Shankar's 'Principles of Quantum Mechanics' and I don't quite understand the point the author is trying to make in this exercise. I get that this wavefunction is not a solution to the Schrodinger equation as it is not continuous at the boundaries and neither is its first derivative. However, I don't see how this fits together with the rest of the question. What is the significance of the operator series not converging and what is the paradox that the author speaks of? An explanation in simple terms would be really appreciated!
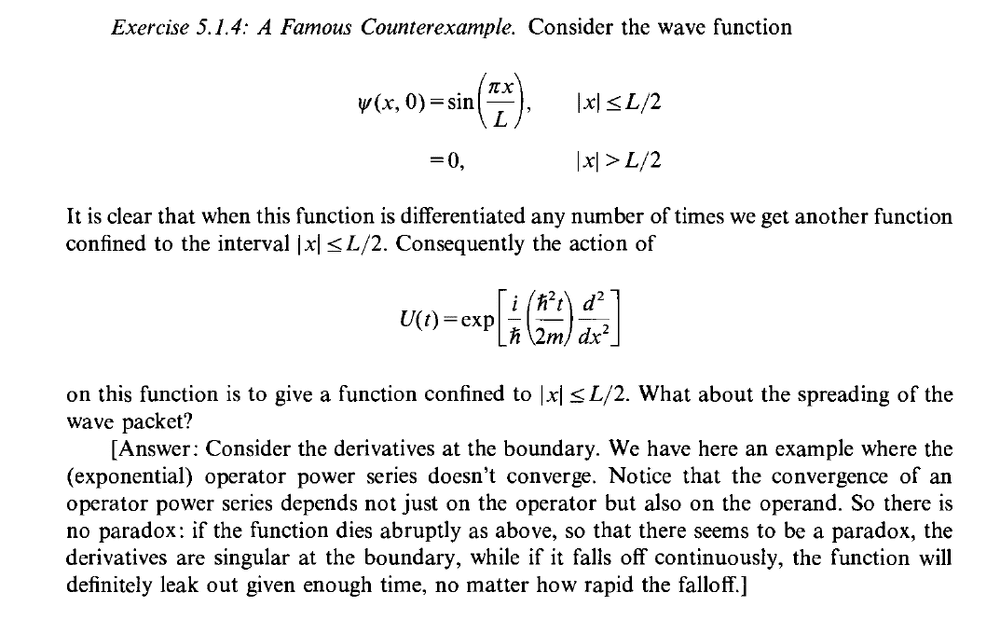
I've been going through Shankar's 'Principles of Quantum Mechanics' and I don't quite understand the point the author is trying to make in this exercise. I get that this wavefunction is not a solution to the Schrodinger equation as it is not continuous at the boundaries and neither is its first derivative. However, I don't see how this fits together with the rest of the question. What is the significance of the operator series not converging and what is the paradox that the author speaks of? An explanation in simple terms would be really appreciated!