(Apparently) simple question rearding module homomorphisms

[Math Amateur]

I am reading Dummit and Foote Chapter 10: Introduction to Module Theory.

I am having difficulty seeing exactly why a conclusion to Proposition 27 that D&F claim is "immediate":

I hope someone can help.

Proposition 27 and its proof read as follows:

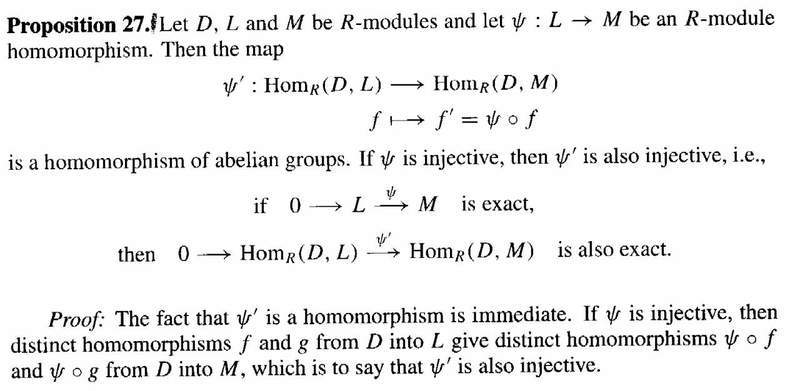
In the first line of the proof (see above) D&F state the following:

"The fact that \psi is a homomorphism is immediate."

Can someone please explain exactly why \psi is a homomorphism?

Would appreciate some help.

Peter

 

[micromass]

Any thoughts? What is it exactly that you need to prove?

Also, could you please shrink the images next time. It's really annoying.

 

[Math Amateur]

Tried to resize image - new image is displayed below.

Peter

 

[Math Amateur]

Another size option would be as follows:

Peter

 

[homeomorphic]

I'd recommend you try to figure it out for yourself, using the definition of phi' they give at the top.

 

[Math Amateur]

Thanks.

Problem is now solved.

Peter

 

[adjacent]

Thanks.

Problem is now solved.

Peter

If you don't mind,can you show us your answer?

 

[Math Amateur]

I received help on the MHB forum.

The solution was as follows:

"We have to verify that for:



\psi'(f+g) = \psi'(f) + \psi'(g) in \text{Hom}_R(D,M).

To do this, let's take an arbitrary element d \in D.

Then:

(\psi'(f+g))(d) = (\psi \circ (f+g))(d) = \psi((f+g)(d)) = \psi(f(d)+g(d)) = \psi(f(d)) + \psi(g(d)) (since \psi is a module homomorphism)

= (\psi \circ f)(d) + (\psi \circ g)(d) = (\psi'(f))(d) + (\psi'(g))(d) = (\psi'(f) + \psi'(g))(d).

Since these two functions are equal for every d \in D they are the same element of \text{Hom}_R(D,M)."

The solution is due to Deveno on the Math Help Boards, Linear and Abstract Algebra forum.

Peter