Are all wave functions with a continuum basis non-normalizable?

[TheCanadian]

For example, I am following the below proof:

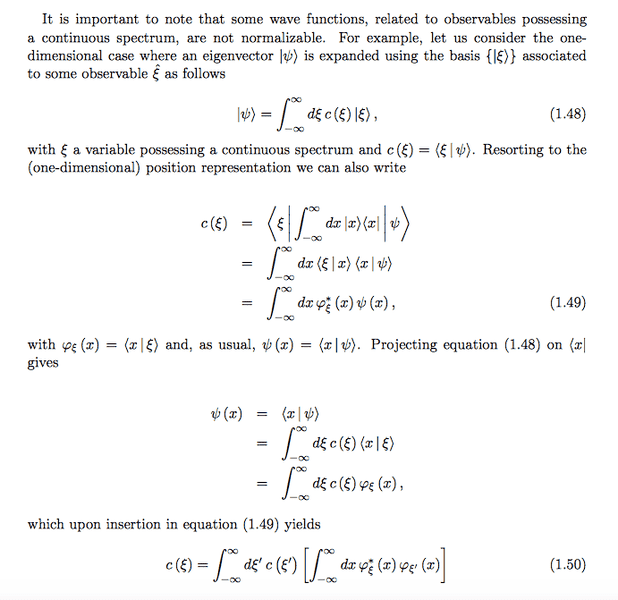
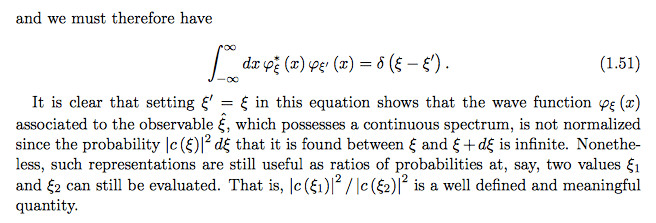
Although the above derivation involves a projection on the position basis, it appears one can generalize this result by using any complete basis. So despite it not being explicitly mentioned here, are all wave functions with any continuum basis non-normalizable?

 

[blue_leaf77]

If your question is reworded to

are all basis functions with any continuum spectrum non-normalizable?

then the answer would be yes.

 

[vanhees71]

Yes, because if you have two eigenstates of a self-adjoint operator in the continuous spectrum then
$$\langle a_1|a_2 \rangle=\int_{\mathbb{R}^3} \mathrm{d}^3 \vec{x} \langle a_1|\vec{x} \rangle \langle \vec{x} |a_2 \rangle=\int_{\mathbb{R}^3} \mathrm{d}^3 \vec{x} u_{a_1}^*(\vec{x}) u_{a_2}(\vec{x})=\delta(a_1-a_2).$$
So the eigenfunctions are "normalizable to a $\delta$ distribution" and thus are not normalizable in the usual sense, because they are not square integrable. They don't belong to the Hilbert space but to the dual of the domain (which is also the co-domain) of the self-adjoint operator and thus a distribution (in the sense of generalized function).

 

[jtbell]

are all wave functions with any continuum basis non-normalizable?

As I understand it, a wave packet (e.g. a Gaussian one) constructed from plane-wave states is an example of a wave function with a continuum basis, which is indeed normalizable.

 

[PeroK]

As I understand it, a wave packet (e.g. a Gaussian one) constructed from plane-wave states is an example of a wave function with a continuum basis, which is indeed normalizable.

Any function can be constructed from the basis functions. The OP confused the properties of the wave function with the properties of the basis functions.

 

[TheCanadian]

If your question is reworded to

then the answer would be yes.

Any function can be constructed from the basis functions. The OP confused the properties of the wave function with the properties of the basis functions.

Thank you for the responses. If the wave function is a linear superposition of the basis functions (which are non-normalizable) in this space, is the wave function not non-normalizable in this space, too? For example, if the wave function is given by

$$ {\lvert \psi \rangle } = \int^\infty_{-\infty} d\xi c(\xi) {\lvert \xi \rangle }$$

and $c(\xi)$ is not normalizable, then wouldn't the wave function in this space be non-normalizable itself?

 

[George Jones]

Yes, e.g., $c\left(\xi\right) = 1$ gives a state that is not norrmalizable, but $c\left(\xi\right)$ does not have to be non-normalizable, e.g.,
$$c\left(\xi\right) = A e^{-\xi^2},$$
in which case $\left| \psi \right>$ is normalizable.

 

[atyy]

Thank you for the responses. If the wave function is a linear superposition of the basis functions (which are non-normalizable) in this space, is the wave function not non-normalizable in this space, too? For example, if the wave function is given by

$$ {\lvert \psi \rangle } = \int^\infty_{-\infty} d\xi c(\xi) {\lvert \xi \rangle }$$

and $c(\xi)$ is not normalizable, then wouldn't the wave function in this space be non-normalizable itself?

The non-normalizable basis functions are relevant when we talk about the position observable. However, a non-normalizable basis function is not an admissible wave function, so it cannot be the wave function of a system either before or after a sharp position measurement. For discrete variables, "collapse onto an eigenvector" is sometimes applicable, but it is not the most general rule. For continuous variables, the usual "collapse onto an eigenvector" rule is never applicable. The most general rule for state reductions is given in https://arxiv.org/abs/0706.3526, eg. Eq 3, 4, 9, 10.

 

[PeroK]

Thank you for the responses. If the wave function is a linear superposition of the basis functions (which are non-normalizable) in this space, is the wave function not non-normalizable in this space, too? For example, if the wave function is given by

$$ {\lvert \psi \rangle } = \int^\infty_{-\infty} d\xi c(\xi) {\lvert \xi \rangle }$$

and $c(\xi)$ is not normalizable, then wouldn't the wave function in this space be non-normalizable itself?

A finite linear combination of non-normalizable functions would, in general, be non-normalizable. But, the wave-function in this case is not a linear combination of the basis functions, in the strict sense. It's constructed from the integral you posted, which you might call a generalised linear combination with a continuous index. In any case, by integrating in this way you produce normalisable functions.

 

[George Jones]

For example, if the wave function is given by

$$ {\lvert \psi \rangle } = \int^\infty_{-\infty} d\xi c(\xi) {\lvert \xi \rangle }$$

Maybe I use non-standard terminology, but I wouldn't call this a "wave function", I would use "ket" or "state vector" or ... I reserve "wave function" for something like

$$\begin{align}
\psi \left( \xi \right) &= \left< \xi | \psi \right>\\
&= \int_{-\infty}^\infty d \xi' c \left( \xi' \right) \left< \xi | \xi' \right>\\
&= \int_{-\infty}^\infty d \xi' c \left( \xi' \right) \delta \left( \xi - \xi' \right)\\
&= c \left( \xi \right)
\end{align}$$

Yes, e.g., $c\left(\xi\right) = 1$ gives a state that is not norrmalizable, but $c\left(\xi\right)$ does not have to be non-normalizable, e.g.,
$$c\left(\xi\right) = A e^{-\xi^2},$$
in which case $\left| \psi \right>$ is normalizable.

Let me flesh out some of the details of the above examples.

For concreteness, start with $\xi = x$ and $c\left( x \right) = 1$ in the first example in the quote so that

$$\left| \psi \right> = \int_{-\infty}^\infty dx \left| x \right>.$$

Then, from the beginning of this post, the position space wave function is

$$\psi \left( x \right) = \left< x | \psi \right> = 1,$$

which is not normalizable since the integral over all space of the constant 1 diverges.

The momentum space wave function for the same ket $\left| \psi \right>$ is

$$\begin{align}
\psi \left( p \right) &= \left< p | \psi \right>\\
&= \int_{-\infty}^\infty dx \left< p | x \right>\\
&= \int_{-\infty}^\infty dx e^{-ipx}\\
&= 2 \pi \delta \left( p \right)\\
&= 2 \pi \delta \left( p - 0 \right)\\
&= 2 \pi \left< p | p=0 \right>,
\end{align}$$

since $\left< p_1 | p_2 \right> = \delta \left( p_1 - p_2 \right)$. This mean, for my example, $\left| \psi \right> = \left| p=0 \right>$.

Note that $\psi \left( x \right) = 1$ is consistent with the position space momentum eigenstate $\psi \left( x \right) = e^{ipx}$ for $p=0$.

I have rushed this in order to finish before going to a meeting that I now have to attend, so I hope that I haven't made too many mistakes. Also, this means that I haven't been able to give any of the details of the example from my previous post, which is normalizable.