Correcting results to fit Rutherford's Scattering Formula

[Fabris]

I'm a Physics undergraduate at University and in my labs module I had to recreate the Rutherford Scattering experiment. The basic setup was similar to this:

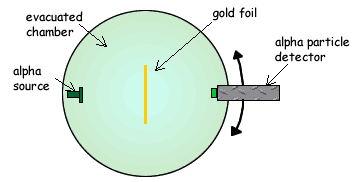
My problem is that this setup only records data in one plane and not 3D. In reality the particles are scattered in a sort of cone similar to this:

One of the tasks is to correct for this so that I can compare my results to Rutherford's scattering formula. I have no idea how to do it and after looking all over the internet all I could come up with talks about solid angle which I haven't even covered in University. I think I may be over-complicating this issue and I'm hoping someone can help me.

 

[Charles Link]

The differential scattering cross section for the whole target (assuming the alpha particle beam illuminated the whole target) is given by $\\$ $\frac{d \sigma}{d \Omega}=\frac{(\frac{d N_{sc}}{dt \, d \Omega})}{(\frac{d N_{inc}}{dt \, d \sigma})}$ . (Note that $N_{sc}=N_{inc}$, i.e. there is a one-to-one mapping between incident particles and scattered particles. Any particles that go in the forward direction, essentially unscattered, are included in the mathematics. Notice also how $d \sigma$ goes from the denominator of the denominator(on the right side of the equation) to the numerator (on the left side of the equation), and $d \Omega$ in the denominator of the numerator winds up in the denominator.) $\\$ Now $d \Omega=\frac{A_{receiver}}{R^2}$ where $R$ is the distance to the receiver from the target. (Here we are computing the solid angle that the receiver subtends.) This should allow you to compute $\frac{dN_{sc}}{dt \, d \Omega}$. It is ok that you only measured in one plane at an angle $\theta$ off-axis. One thing you need to determine is where Rutherford's $\theta$ is measured from, i.e. if it gets scattered back at you, is $\theta =0$ or is $\theta=180$ for this case? $\\$ In addition, $\frac{d N_{inc}}{(dt \, d\sigma)}=\frac{d N_{inc}}{(dt \, A_{receiver})}$. (I assume you initially put the receiver at the target and measured the counts per second, etc.) $\\$ Putting it all together, you should be able to compute $\frac{d \sigma}{d \Omega}$ as a function of $\theta$. This will give you $\frac{d \sigma}{d \Omega}$ for the whole target. If you want to compute $\frac{d \sigma}{d \Omega}$ for a single gold atom, you need to know how many atoms are in your target and divide by this number. $Number \, of \, atoms=[\frac{(mass \, in \, grams \, of \, target)}{(A.W._{gold})}]6.02 E+23$. $\\$ Editing: One additional note: If you want to get an estimate of the size (area) of the scatterer $\sigma$, i.e. of a single atom, you can take integrate $\frac{d \sigma}{d \Omega}$ for a single atom over the $4 \pi$ solid angle, leaving off the unscattered part. i.e. $\sigma=\int \frac{d \sigma}{d \Omega} \, sin(\theta) \, d \theta \, d \phi$. (For the case of scattering off of a solid target, e.g. bee-bees incident on a hard spherical target, this last formula is quite precise, but for the case of an inverse square law type force that gets screened by electrons, etc., computing $\sigma$ is simply an approximation. Notice for the case of bee-bees onto a hard spherical target, why you would not count anything that was unscattered=i.e. that missed the target. The computation for $\sigma$ for this case gives the projected area of the target, i.e. $\sigma= \pi a^2$ where $a$ is the radius of the spherical target.) $\\$ I could mention one additional item that might be of interest about the mathematics of the scattering theory. Essentially, there is a mapping of regions in the incident plane $\sigma$ to regions $\Omega$ i.e. locations $\Omega (\theta, \phi)$ of the space of the scattered beam. Thereby the function $\frac{d \sigma }{d \Omega}$ can be computed if one knows how the particles, such as bee-bees, get scattered off of the hard spherical target. i.e. where they wind up in the scattered space depends precisely on where they hit the target. $\sigma$ maps into $\Omega$. The $\sigma(r)$ can be viewed as any $\sigma$ inside a radius of $r$, and the $\Omega(\theta,\phi)$ as anything inside the cone. Then $\frac{d \sigma}{d \Omega}$ is how fast the $\sigma$ region changes compared to the scattered cone $\Omega$. When $\frac{d \sigma}{d \Omega}$ is integrated over the whole $4 \pi$ of $d \Omega$, the result is the complete target area $\sigma$.

 

[Fabris]

The differential scattering cross section for the whole target (assuming the alpha particle beam illuminated the whole target) is given by $\\$ $\frac{d \sigma}{d \Omega}=\frac{(\frac{d N_{sc}}{dt \, d \Omega})}{(\frac{d N_{inc}}{dt \, d \sigma})}$ . (Note that $N_{sc}=N_{inc}$, i.e. there is a one-to-one mapping between incident particles and scattered particles. Any particles that go in the forward direction, essentially unscattered, are included in the mathematics. Notice also how $d \sigma$ goes from the denominator of the denominator(on the right side of the equation) to the numerator (on the left side of the equation), and $d \Omega$ in the denominator of the numerator winds up in the denominator.) $\\$ Now $d \Omega=\frac{A_{receiver}}{R^2}$ where $R$ is the distance to the receiver from the target. (Here we are computing the solid angle that the receiver subtends.) This should allow you to compute $\frac{dN_{sc}}{dt \, d \Omega}$. It is ok that you only measured in one plane at an angle $\theta$ off-axis. One thing you need to determine is where Rutherford's $\theta$ is measured from, i.e. if it gets scattered back at you, is $\theta =0$ or is $\theta=180$ for this case? $\\$ In addition, $\frac{d N_{inc}}{(dt \, d\sigma)}=\frac{d N_{inc}}{(dt \, A_{receiver})}$. (I assume you initially put the receiver at the target and measured the counts per second, etc.) $\\$ Putting it all together, you should be able to compute $\frac{d \sigma}{d \Omega}$ as a function of $\theta$. This will give you $\frac{d \sigma}{d \Omega}$ for the whole target. If you want to compute $\frac{d \sigma}{d \Omega}$ for a single gold atom, you need to know how many atoms are in your target and divide by this number. $Number \, of \, atoms=[\frac{(mass \, in \, grams \, of \, target)}{(A.W._{gold})}]6.02 E+23$. $\\$ Editing: One additional note: If you want to get an estimate of the size (area) of the scatterer $\sigma$, i.e. of a single atom, you can take integrate $\frac{d \sigma}{d \Omega}$ for a single atom over the $4 \pi$ solid angle, leaving off the unscattered part. i.e. $\sigma=\int \frac{d \sigma}{d \Omega} \, sin(\theta) \, d \theta \, d \phi$. (For the case of scattering off of a solid target, e.g. bee-bees incident on a hard spherical target, this last formula is quite precise, but for the case of an inverse square law type force that gets screened by electrons, etc., computing $\sigma$ is simply an approximation. Notice for the case of bee-bees onto a hard spherical target, why you would not count anything that was unscattered=i.e. that missed the target. The computation for $\sigma$ for this case gives the projected area of the target, i.e. $\sigma= \pi a^2$ where $a$ is the radius of the spherical target.) $\\$ I could mention one additional item that might be of interest about the mathematics of the scattering theory. Essentially, there is a mapping of regions in the incident plane $\sigma$ to regions $\Omega$ i.e. locations $\Omega (\theta, \phi)$ of the space of the scattered beam. Thereby the function $\frac{d \sigma }{d \Omega}$ can be computed if one knows how the particles, such as bee-bees, get scattered off of the hard spherical target. i.e. where they wind up in the scattered space depends precisely on where they hit the target. $\sigma$ maps into $\Omega$. The $\sigma(r)$ can be viewed as any $\sigma$ inside a radius of $r$, and the $\Omega(\theta,\phi)$ as anything inside the cone. Then $\frac{d \sigma}{d \Omega}$ is how fast the $\sigma$ region changes compared to the scattered cone $\Omega$.

Thank you for the answer but my lab work is no where near as advanced as what you have written. The goal of the experiment was to collect data at varying angles and to validate Rutherford's formula:

All the constant are taken out and put into one (A) to give:

The collected data has to be plotted next onto this theoretical curve and then the values of B and A are to be determined graphically (trial and error for the best match). The main reason for this experiment is to become proficient at data analysis and to get used to writing scientific reports. Now my problem is I don't know how to correct for the fact that the data was collected in only one plane.

I didn't collect data at 0 degrees (going straight through) as the instructions specifically requested to omit this reading. Also I don't understand how that reading could be of any use when Rutherford's scattering formula has an asymptote at 0 degrees.

 

[Charles Link]

It looks like all they are looking for from you is to try to curve fit $N(\theta)= A/sin^4(\theta/2)$ for a range where you got reasonably good data. At $\theta=0$ this number goes up the y-axis, but you could try a graph of $N(\theta)$ vs. $\theta$ from $\theta=180$ (back-scattered) to some angle $\theta= 45$ degrees, etc. i.e. compare theoretical to experimental and choose $A$ for the best fit to your experiment. $\\$ Editing... If you measured the angle in the laboratory, the $B$ in your formula should be equal to zero. I'd enjoying seeing a few of your data points of $N (\theta)$ vs. $\theta$. $\\$ Incidentally, it was with Rutherford's experiment that they came to conclude there were actually nuclei in atoms. Up until this time, they thought solids were pretty much uniformly dense, like jello, and they never expected that a piece of gold foil would be able to deflect one of these alpha particles appreciably.

 

[Fabris]

It looks like all they are looking for from you is to try to curve fit $N(\theta)= A/sin^4(\theta/2)$ for a range where you got reasonably good data. At $\theta=0$ this number goes up the y-axis, but you could try a graph of $N(\theta)$ vs. $\theta$ from $\theta=180$ (back-scattered) to some angle $\theta= 45$ degrees, etc. i.e. compare theoretical to experimental and choose $A$ for the best fit to your experiment. $\\$ Editing... If you measured the angle in the laboratory, the $B$ in your formula should be equal to zero. I'd enjoying seeing a few of your data points of $N (\theta)$ vs. $\theta$.

Does that mean that I do not need to correct the data in any way? The lab script says "In order to compare your results with “Rutherford’s scattering formula”, you must first make a correction for the fact that we have only sampled the scattering in the horizontal plane, whereas the α-particles are actually scattered in a 3D cone." and then "Consider what factors make a correction to the angular scale necessary.". I am perfectly fine with all other aspects of the experiment and didn't even think I would need to change the data in any way, however, these two sentences threw me off-guard.

And yes I am aware that B should be 0, however, in the apparatus used the source and detector were not aligned perfectly and therefore the actual peak was around 2-3 degrees.

Edit: What you said about fitting my data onto the curve is exactly what they are asking.

 

[Charles Link]

Does that mean that I do not need to correct the data in any way? The lab script says "In order to compare your results with “Rutherford’s scattering formula”, you must first make a correction for the fact that we have only sampled the scattering in the horizontal plane, whereas the α-particles are actually scattered in a 3D cone." and then "Consider what factors make a correction to the angular scale necessary.". I am perfectly fine with all other aspects of the experiment and didn't even think I would need to change the data in any way, however, these two sentences threw me off-guard.

And yes I am aware that B should be 0, however, in the apparatus used the source and detector were not aligned perfectly and therefore the actual peak was around 2-3 degrees.

The angle $\theta$ is the polar angle. Had you collected data in 3-D, you would have found a complete $\phi$ symmetry. That is why $\phi$ does not appear in the Rutherford formula=it's independent of $\phi$. Your laboratory angle is precisely $\theta$, neglecting any misalignments, etc.

 

[Fabris]

The angle $\theta$ is the polar angle. Had you collected data in 3-D, you would have found a complete $\phi$ symmetry. That is why $\phi$ does not appear in the Rutherford formula=it's independent of $\phi$. Your laboratory angle is precisely $\theta$, neglecting any misalignments, etc.

Ok, thanks a lot. :)

 

[Charles Link]

One additional comment: You may have taken data for angles $\theta$ on both sides of the peak at $\theta=0$ degrees. In the polar coordinate system of interest, these are actually the same angle for $\theta$ on both sides of the peak, but the azimuthal angle $\phi$ differs by $\pi$ radians or 180 degrees at these two locations.