Directional & Partial Derivatives .... working from the definition

[Math Amateur]

I am reading the book "Several Real Variables" by Shmuel Kantorovitz ... ...

I am currently focused on Chapter 2: Derivation ... ...

I need help with an element of the proof of Kantorovitz's Proposition on pages 61-62 ...

Kantorovitz's Proposition on pages 61-62 reads as follows:

I am trying to understand the above proof in terms of the definitions of directional and partial derivatives (in terms of limits) ... but I am having trouble understanding equation (2.3) above ... specifically I am concerned regarding how (2.3) follows from Kantorovitz's definition of partial derivative ...

I will explain my difficulties in terms of Kantorovitz's definitions as he develops them on page 60 ... as follows:

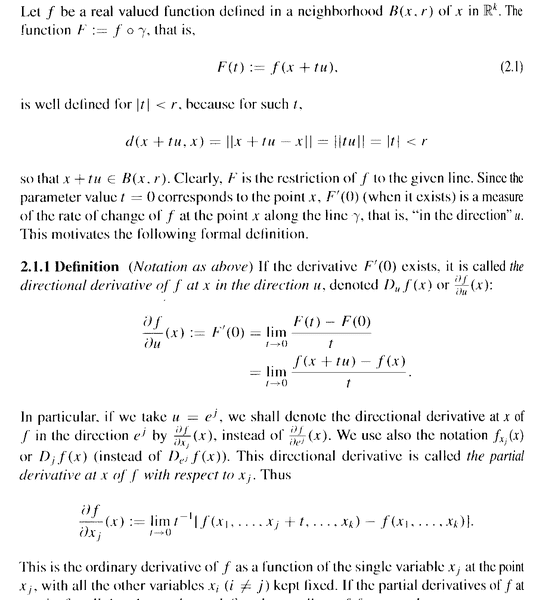Now ... I am trying to understand how the definition of partial derivative applies to equation (2.3) in the proof of the proposition ... so for equation (2.1) of the definition we put $u = e^j$ (because we are dealing with partial derivatives) ... ... and so (2.1) becomes:$F(t) = f( x + t e^j)$

so then for $F_j$ in the proof (see the expression that is above the expression (2.3)) ... we have

$F_j (t) = f ( x + h^{j-1} + te^j )$and we appear to be dealing (for some reason?) with $( x + h^{j-1} )$ instead of $x$ ...

... which is OK ... just put $x = x + h^{j-1}$ ...... BUT ...In Definition 2.1.1 Kantorovitz defines the partial derivative this way:$\frac { \partial f }{ \partial x_j } := F'(0) = \lim_{ t \rightarrow 0 } \frac{ F(t) - F(0) }{t}$$= \lim_{ t \rightarrow 0 } \frac{ f ( x + h^{j-1} + te^j ) - f(x) }{t}$... ... is the above correct?Now ... my question is as follows: (pertaining largely to equation (2.3) )

What is the definition of $F_j'(t)$ ... and working strictly and rigorously from the definition how do we obtain

$F'_j (t) = \frac { \partial f }{ \partial x_j } f ( x + h^{j-1} + te^j )$

Hope someone can help ...

Peter***NOTE***

I have to say I find it somewhat confusing in trying to work from the definition of partial derivative, that Kantorovitz gives the definition for partial and directional derivative in terms of expressions where $t$ tends to zero ... and then equation (2.3) above is a partial derivative with $t$ as a variable ... as in $F'_J(t)$ ... surely $t \rightarrow 0$ as per the definition ...

 

[andrewkirk]

\begin{align*}
F'_j(t)
&=\frac d{dt}f(x+h^{j-1}+te^j)\\
&=\lim_{s\to 0}\left(
\frac{f(x+h^{j-1}+(t+s)e^j)-f(x+h^{j-1}+te^j)}{s}\right)\\
&=\lim_{s\to 0}\left(
\frac{f(x+h^{j-1}+te^j+se^j)-f(x+h^{j-1}+te^j)}{s}\right)\\
&=\lim_{s\to 0}\left(
\frac{f(x+h^{j-1}+te^j+se^j)-f(x+h^{j-1}+te^j)}{s}\right)\\
&=\lim_{s\to 0}\left(
\frac{f(y+se^j)-f(y)}{s}\right)
\quad\quad\textrm{[where $y=x+h^{j-1}+te^j$]}\\
&=\frac{\partial f}{\partial u}(y)
\quad\quad\textrm{[where $u=e^j$, and refer Definition 2.1.1]}\\
&=\frac{\partial f}{\partial x_j}(y)
\quad\quad\textrm{[just changing notation from Directional Derivative to Partial Derivative, per Definition 2.1.1]}\\
&=\frac{\partial f}{\partial x_j}(x+h^{j-1}+te^j)
\quad\quad\textrm{[substituting back for $y$]}
\end{align*}

 

[Math Amateur]

\begin{align*}
F'_j(t)
&=\frac d{dt}f(x+h^{j-1}+te^j)\\
&=\lim_{s\to 0}\left(
\frac{f(x+h^{j-1}+(t+s)e^j)-f(x+h^{j-1}+te^j)}{s}\right)\\
&=\lim_{s\to 0}\left(
\frac{f(x+h^{j-1}+te^j+se^j)-f(x+h^{j-1}+te^j)}{s}\right)\\
&=\lim_{s\to 0}\left(
\frac{f(x+h^{j-1}+te^j+se^j)-f(x+h^{j-1}+te^j)}{s}\right)\\
&=\lim_{s\to 0}\left(
\frac{f(y+se^j)-f(y)}{s}\right)
\quad\quad\textrm{[where $y=x+h^{j-1}+te^j$]}\\
&=\frac{\partial f}{\partial u}(y)
\quad\quad\textrm{[where $u=e^j$, and refer Definition 2.1.1]}\\
&=\frac{\partial f}{\partial x_j}(y)
\quad\quad\textrm{[just changing notation from Directional Derivative to Partial Derivative, per Definition 2.1.1]}\\
&=\frac{\partial f}{\partial x_j}(x+h^{j-1}+te^j)
\quad\quad\textrm{[substituting back for $y$]}
\end{align*}

Thanks Andrew ..

Just working through your post and reflecting on what you have written ...

Most grateful for the help ...

Peter