Functor between the category of Hilbert Space and the category of sets

[snypehype46]

I have a question that is related to categories and physics. I was reading this paper by John Baez in which he describes a TQFT as a functor from the category nCob (n-dimensional cobordisms) to Vector spaces. https://arxiv.org/pdf/quant-ph/0404040.pdf.
At the beginning of the paper @john baez mentions this

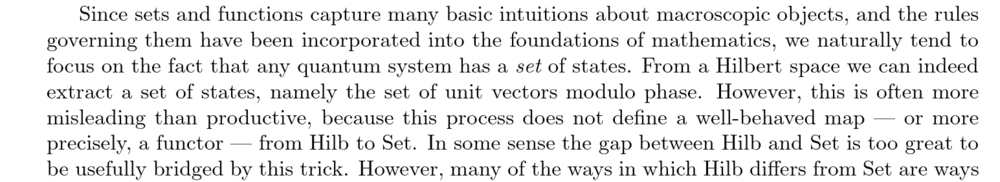
Now I am familiar with (very) basic category theory and quantum mechanics, but could someone expand on what exactly is meant by the fact there is not a well defined functor from the category of hilbert spaces and the category of sets?

 

[nuuskur]

A functor is a set of rules of how to associate objects and arrows between them to objects and arrows in the target category. Given a Hilbert space $H$, there is some set of states extracted from it, call it $X_H$. So the natural candidate is to put $H\mapsto X_H$. The problem is, as I understand it, we don't know what sort of map we associate with an arrow $H\to H'$ in $\mathrm{Hilb}$ such that the correspondence would be functorial (i.e well behaved).

 

[snypehype46]

Thanks for the reply. Yes, I think I understand that is what's intended. My question is more: why is it not possible to find such a functor?

 

[fresh_42]

Thanks for the reply. Yes, I think I understand that is what's intended. My question is more: why is it not possible to find such a functor?

We always have the forgetful functor from any category to the category of sets: $\mathcal{F}:\mathcal{H}\longrightarrow \mathcal{S}$ which simply "forgets" the structure, here the structure of the Hilbert spaces. Now the problem described above is a different one: We have some mapping $\mathbf{F}:\mathcal{H}\longrightarrow \mathcal{S}$ given by the mapping to the set of unit vectors, however, this does not define a functor. A functor should preserve subsets, quotients, i.e. mappings in general:
$$
\varphi : {H}_1\longrightarrow {H}_2 \Longrightarrow \mathcal{F}(\varphi ): \mathcal{F}({H}_i) \longrightarrow \mathcal{F}({H}_j)
$$
with $(i,j)=(1,2)$ for covariant functors $\mathcal{F}$ and $(i,j)=(2,1)$ for contravariant functors $\mathcal{F}.$ In short: A functor maps objects to objects and arrows to arrows between those objects. The forgetful (covariant) functor does this: if we have a map between Hilbert spaces, then we get the same map between the underlying sets, without bothering linearity or any other structure.
If a mapping between categories does not relate the mappings between the two categories, then it is no functor. A relationship only between objects does not count.

An example:
Given the category of Lie Algebras $\mathcal{G}$. Then $$\mathfrak{A(g)}=\{\alpha:\mathfrak{g}\longrightarrow \mathfrak{g}\, : \,[\alpha (X),Y]+[X,\alpha (Y)]=0 \}$$ for a Lie algebra $\mathfrak{g}$ defines another Lie algebra $\mathfrak{A(g)}$. Hence
$$
\mathfrak{A}(.)\, : \,\mathcal{G}\longrightarrow \mathcal{G}
$$
is a mapping between the objects of two categories (which are the same in this case). But it is not a functor. E.g. consider the inclusion map $\iota\, : \,\mathfrak{h}\stackrel{\subseteq }{\longrightarrow }\mathfrak{g}$ between two Lie algebras. Then there is no natural way to give $\mathfrak{A}(\iota)$ a meaning, since $\mathfrak{A(h)}$ and $\mathfrak{A(g)}$ must no longer be included or otherwise related.