- #1
lightlightsup
- 95
- 9
- Homework Statement
- Graph ##θ=\frac{π}{4}## on a Polar Coordinate System.
- Relevant Equations
- Why does the line go into the opposite quadrant as well?
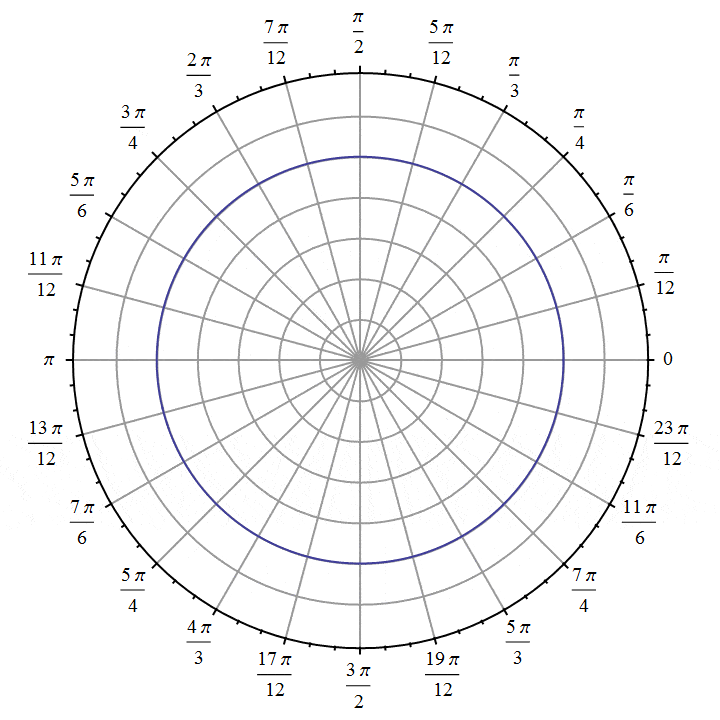
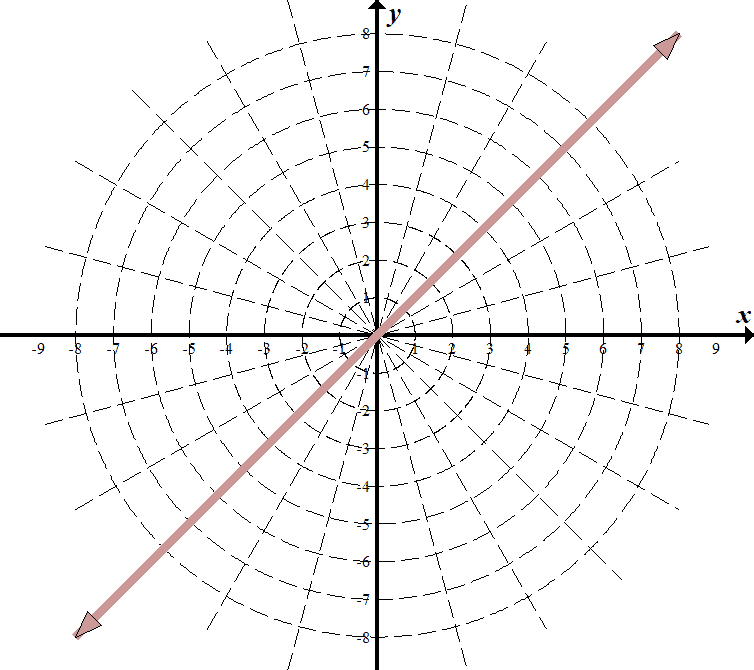
When you graph something like ##θ=\frac{π}{4}## on a Polar Coordinate System:
Why does the line go into the opposite quadrant as well?
I can intuitively understand why it is in the first quadrant: ##θ = 45°## there and so all possible values of ##r## would apply there, giving you a straight line headed outwards at an angle of ##45°##.
So, why does the line go into the opposite quadrant as well?
Isn't ##r## always positive? Or, is that something we define beforehand as being either positive or negative?
Why does the line go into the opposite quadrant as well?
I can intuitively understand why it is in the first quadrant: ##θ = 45°## there and so all possible values of ##r## would apply there, giving you a straight line headed outwards at an angle of ##45°##.
So, why does the line go into the opposite quadrant as well?
Isn't ##r## always positive? Or, is that something we define beforehand as being either positive or negative?