How does a Bell State lead to a probability in the CHSH game

[IsaiahvH]

Hi,

I am a student in the Netherlands, and I'll be attending university next year. However, I am doing some form of research on Quantum Computing with another student for our so-called "profielwerkstuk" but my understanding of Quantum Physics and math is sometimes not at the level that is needed. I am hoping some great person who does have the knowledge and skill to explain can help me with the following problem.

I have trouble understanding how the CHSH game, as described in this paper (and shortly explained in this post), works. I understand that 75% is the maximum probability of winning in a classical system. I understand the state given,

$$\frac{\left| 00 \right> + \left| 11 \right>}{\sqrt{2}}$$

As I understand this, this refers to 50% chance that both qubits are | 0>, and 50% chance that they are both | 1>. This can be prepared as such,

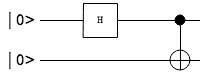
However, does this Bell State in the CHSH game lead to a probability of
$$\cos\left(\frac{1}{8}\pi\right)\approx0.85$$
I have made a visual representation of the Bloch Sphere from one side in Desmos, and I see how a certain angle corresponds to a certain probability. This might be completely incorrect and at the very least immensely incomplete, but this is how I picture a qubit.

How does the CHSH game conclude that the probability to 'win' in a quantum system is cos(1/8*pi), or an angle of 45° in my Desmos example?

Kind regards,

Isaiah van Hunen

 

[IsaiahvH]

Hi,
Thank you for your answer!

I particularly found your widgets very useful.
However, I still don't understand some aspects.

Firstly, I don't exactly understand what it means to measure 'on the Z-axis' and to apply rotations 'on the X-axis'.
How does the rotation on one axis relate to measuring the agreement rate on another axis?
As said on the widget page, the rate of agreement is $\cos\left(\frac{\theta}{2}\right)^2$, where $\theta$ is the angle between axes. I don't know how to picture this angle.
Is $\theta$ $90^{\circ}$ if there is a $0^{\circ}$ rotation on the X-axis, and the qubit is measured in the Z-axis?
What is $\theta$ if there is a $-45^{\circ}$ rotation on the X-axis?

At first, I incorrectly thought of $\theta$ that it was the difference in angle on the X-axis between the two qubits, and imagined the start offset between the angles to be that $-45^{\circ}$, but if then Alice and Bob are both given a $1$ and both rotate their qubit by $90^{\circ}$, (thus the angle of qubit A:$45^{\circ}$ and the angle of qubit B:$90^{\circ}$), there was a difference of $45^{\circ}$ instead of the $135^{\circ}$ mentioned in the text on the widget page.

About the actions of Alice and Bob on their qubits, you said

and their contributions combine.

Do you mean that if Alice rotates her qubit by $90^{\circ}$, so does Bob's qubit? That they have the same rotation among the X-axis at all times, as each rotation is copied to the other?

The text on the widget page says

The trick quantum strategies can use is that, if you start with the measurement axes separated -45°, rotating by 90° once gets you to +45° but rotating by 90° twice gets you to 135°.

However, Alice only rotates her qubit $90^{\circ}$ and so does Bob, so the angles must add up?

Kind regards,

Isaiah van Hunen

 

[Strilanc]

These are all good questions, but they're also very basic-bootstrapping-ish questions. They're things explained by textbooks, that require a bit of exercise to really grok.

For the rotations and angles stuff, you need to understand the Bloch sphere. You should find out how the state of a qubit is placed on the Bloch sphere and how a single-qubit operation acts like a rotation around the Bloch sphere. And vice versa: going from the Bloch sphere stuff back to matrices and vectors.

For some of the other stuff, you might find the video series Quantum Computing for the Determined to be useful. Other than that... I'd recommend grabbing a quantum information textbook.

 

[IsaiahvH]

These are all good questions, but they're also very basic-bootstrapping-ish questions. They're things explained by textbooks, that require a bit of exercise to really grok.

For the rotations and angles stuff, you need to understand the Bloch sphere. You should find out how the state of a qubit is placed on the Bloch sphere and how a single-qubit operation acts like a rotation around the Bloch sphere. And vice versa: going from the Bloch sphere stuff back to matrices and vectors.

For some of the other stuff, you might find the video series Quantum Computing for the Determined to be useful. Other than that... I'd recommend grabbing a quantum information textbook.

Thank you for your reply.

The actual problem is that I do not have the required knowledge for a thorough understanding of quantum phsyics.
I chose this as my subject for my sort-of 'essay', although a bit different, but I have never been educated on the subject except by myself.
I'm fully aware the vast majority of quantum physics is inaccessible for me, and finding any information on the matter has proven to be hard; everything on the internet is at expert level and I'm surprised how anyone could ever start understanding any of it.
Some really nice examples use images of arrows to represent qubits, which is for me a better approach as I can understand those, but not the math used everywhere that I don't master.

I already watched the entirety of the video series you recommended, and sadly don't have the time to buy a textbook (or learn the more complicated math).
If I'm stuck at some point, I'll have to accept that and that's a shame but that'll be all.
However, I strive to understand as much of it as I can.

Therefore I'd still like to ask you some questions.
The Bloch sphere, as shown below, indeed includes an angle $\theta$ seemingly between the Z-axis and the XY plane.
(Also, I now understand why the Z-axis is chosen for measurement, this is the chosen axis to represent $\left|0\right>$ or $\left|1\right>$?)

However, I still fail to picture something rotating along the X-axis, or how this would affect $\theta$.

I realize it is hard to properly explain something to someone who does not have the education on the matter one would expect.
However, if anyone is up for a challenge, that would be great! (And my essay would be better)

 

[Strilanc]

That angle isn't the same thing as the rotation angle, though they both use the symbol $\theta$.

I'm not sure what you mean by failing to picture something rotating along the X axis. I mean... just stick the state to the sphere, turn the sphere, and now the state is at a new point. Mostly you just have to convince yourself that, for the entangled state we start with, Alice and Bob rotating their individual qubits around the X axis will end up rotating the parity of their entangled state instead.

So you'd figure out the unitary matrix that corresponds to those single-qubit rotations, parametrized by the amount of rotation, use the tensor product to expand the matrices to apply to a 2-qubit system, hit the entangled state vector with the matrix, and see how the combination of the two affected how much amplitude was in bits-agree states vs bits-disagree states.

Here's another blog post that might help, in that it gives a simplified way to understand what's happening to two entangled qubits.

 

[IsaiahvH]

Hmm I think I'm close to possibly understanding this bit. But then again, it's quantum.

However, is this representation of the two qubits held by Alice and Bob correct?

It follows the steps described in https://redirect.viglink.com/?format=go&jsonp=vglnk_147853232141816&key=6afc78eea2339e9c047ab6748b0d37e7&libId=iv83fbdd010009we000DL1o9mrbfdilnm7&loc=https%3A%2F%2Fwww.physicsforums.com%2Fthreads%2Fhow-does-a-bell-state-lead-to-a-probability-in-the-chsh-game.892244%2F&v=1&out=http%3A%2F%2Falgorithmicassertions.com%2Fquantum%2F2015%2F10%2F11%2FBell-Tests-vs-No-Communication.html&title=How%20does%20a%20Bell%20State%20lead%20to%20a%20probability%20in%20the%20CHSH%20game%20%7C%20Physics%20Forums%20-%20The%20Fusion%20of%20Science%20and%20Community&txt=the%20widgets%20I%20made%20in%20this%20blog%20post mentioned earlier.
It basically tells Alice to rotate her qubit -45°, and then to both Alice and Bob to turn their qubit 90° if given a 1 by the referee, and not to do anything if given a 0.
Then, they both measure their qubit and give that back as their move.

However, something must be wrong with my understanding because I do not see the outcomes in the widget correlating to my diagram.
Looking at each possible combination of given bits;
If both given a 0, the angle between them is 45°, and thus they agree $\cos(\frac{45°}{2})^2\approx0.85$ of the time, and thus winning $0.85$ of the time.
If Alice is given a 0 and Bob a 1, the angle between them is 135°, and thus they agree $\cos(\frac{135°}{2})^2\approx0.15$, and thus winning $0.15$ of the time while this should be $0.85$.
Similarly, if Alice is given a 1 and Bob a 0, the angle between them is 45°, and thus they agree $\cos(\frac{135°}{2})^2\approx0.85$, and thus winning $0.85$ of the time.
Lastly, if Alice is given a 1 and Bob a 1, the angle between them is 45°, and thus they agree $\cos(\frac{135°}{2})^2\approx0.85$, and thus winning $0.15$ of the time, for they should return different bits to win, while this should be $0.85$ .

I suppose this has something to do with the fact that

1) The Z-parity of two qubits can be thought of as a qubit.
2) Both sides can operate on this parity (e.g. by applying X rotations), and their contributions combine.

This is still not clear to me, however. What does it mean to combine their contributions?

Many thanks,

Isaiah

 

[Strilanc]

The issue you're having is that you're working with states separately, but they're entangled. Parts of the up up case will interfere destructively with parts of the down down case. You have to think in terms of what's happening to the amplitudes to understand the overall picture.

(Thinking in terms of the amplitudes is how the concept of the relationship between the two qubits itself acting like a qubit is justified. I see now that you're not able to understand that concept yet.)

So, figure out what matrix and X rotation corresponds to. Figure out how to apply that matrix to individual qubits. Figure out how to apply it to the entangled system. Then you'll see how the amplitudes end up mostly in the winning states.