- #1
TeslaPow
- 40
- 1
Need some help on how to solve the integration formula for Maxwell speed distribution, here is the procedure on
how to solve for the kinetic energy:
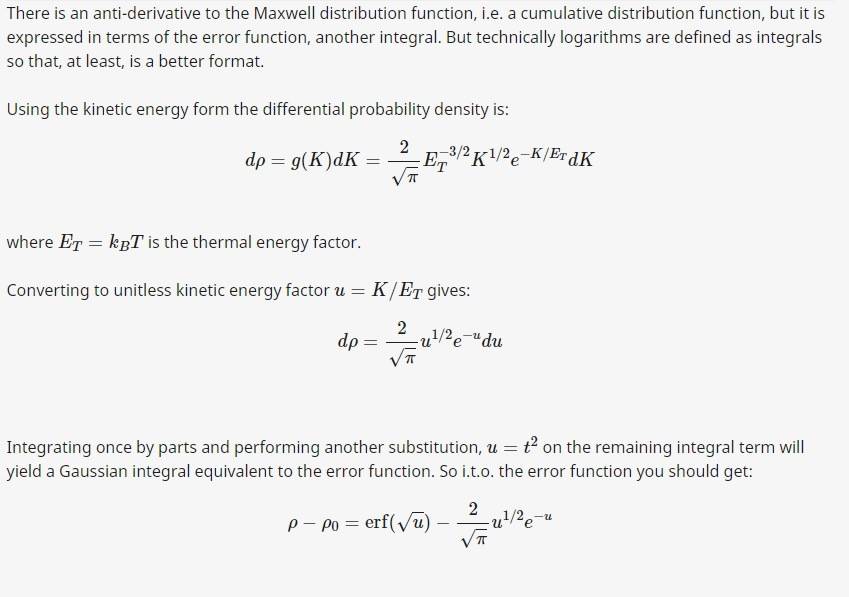
Not familiar with the error function yet, but the result for the kinetic energy integration is:
https://www.wolframalpha.com/input/?i=d/du(erf(sqrt(u))-2/(sqrt(pi))*sqrt(u)*e^(-u))
How to go on about solving for the speed distribution is the topic for this problem.
http://integral-table.com/
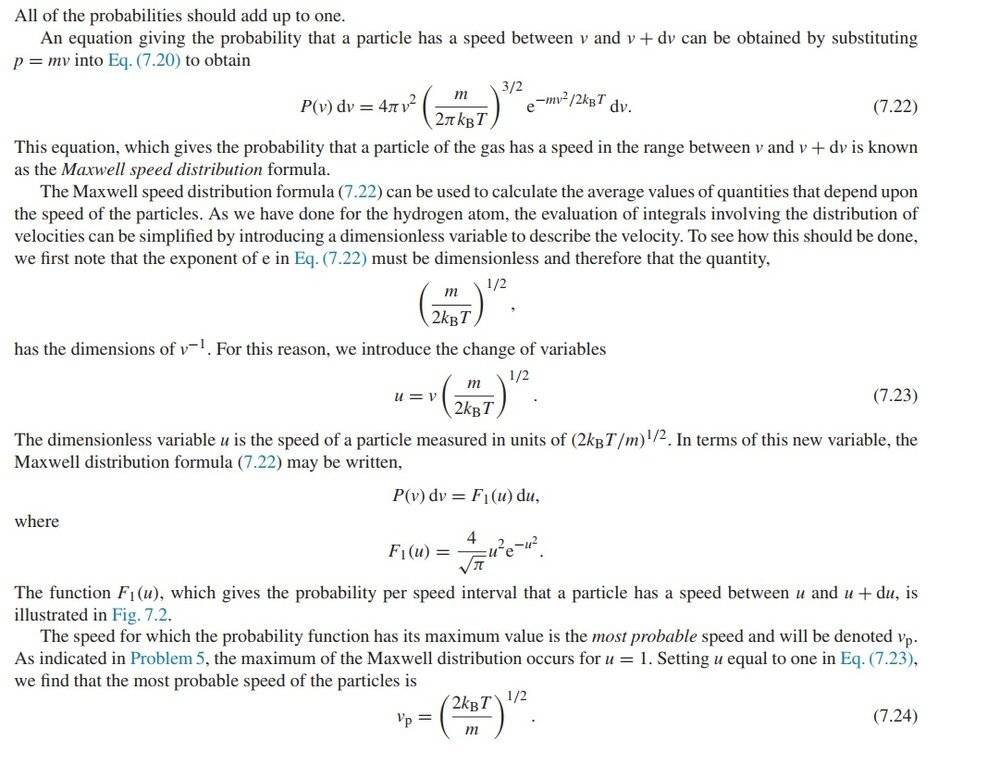
how to solve for the kinetic energy:
Not familiar with the error function yet, but the result for the kinetic energy integration is:
https://www.wolframalpha.com/input/?i=d/du(erf(sqrt(u))-2/(sqrt(pi))*sqrt(u)*e^(-u))
How to go on about solving for the speed distribution is the topic for this problem.
http://integral-table.com/
Last edited: