- #1
ing_it
- 27
- 1
Homework Statement
We have a wire of the length L=0.2m which is uniformly charged with a linear density of Lambda = 3nC/cm.
Find the intensity (E) of the electric field in point A1 whose perpendicular distance from the wire is a=0.05m.
The wire is in a vacuum.
Compare the intensity with the intensity you calculate through Gauss' Law and explain any differences in your answers.
Homework Equations
I will be using h in my calculations. h=L. I just used it because it's easier to think about a cylinder while using h.
No Gauss law:
[tex] E = {\frac {Q}{4 \pi \epsilon_0 r^2}}[/tex]
[tex] Q = \lambda h[/tex]
With Gauss' law.
[tex] \phi= {\frac {Q}{\epsilon_0}}[/tex]
[tex] \phi = E \cdot S_{surface}[/tex]
[tex] Q= \lambda h
The Attempt at a Solution
Please look at the picture I added. I have the problem drawn out there.
First I'm going to use Gauss' law because I feel like I've got that one right (but I might just feel that way and will end up way off)
[tex] \phi = \oint _S \vec{E} \cdot \vec{dS} = \int_{base1} \vec{E} \cdot \vec{dS} + \int_{base2} \vec{E} \cdot \vec{dS} + \int_{surface} \vec{E} \cdot \vec{dS} = \int_{base1} E dS cos {\frac{\pi}{2}} + \int_{base2} E dS cos {\frac{\pi}{2}} + \int_{surface} E dS cos 0 = E \int_{surface} dS = ES_{surface}[/tex]
since
[tex] \phi = E \cdot S_{surface}[/tex] and [tex] \phi= {\frac {Q}{\epsilon_0}}[/tex]
then
[tex] E \cdot S_{surface} = {\frac{Q}{\epsilon_0}}[/tex]
[tex] E 2 \pi a h = {\frac{\lambda h}{\epsilon_0}}[/tex]
[tex] E = {\frac{\lambda}{2 \pi \epsilon_0 a}}[/tex]
Is this part correct?
And then onto the part where I'm not so sure.
I started out with the same thing as above.
[tex] \phi = \oint _S \vec{E} \cdot \vec{dS} = \int_{base1} \vec{E} \cdot \vec{dS} + \int_{base2} \vec{E} \cdot \vec{dS} + \int_{surface} \vec{E} \cdot \vec{dS} = \int_{base1} E dS cos {\frac{\pi}{2}} + \int_{base2} E dS cos {\frac{\pi}{2}} + \int_{surface} E dS cos 0 = E \int_{surface} dS = ES_{surface}[/tex]
[tex] \phi = E S_{surface} = E 2 \pi a h [/tex]
But once I got to this point I didn't use Gauss' law (I hope. We used this in class before we started using Gauss' law so I hope it's not just some variation of it) I substituted this for my E in "phi = E Ssurface" :
[tex] E = {\frac {Q}{4 \pi \epsilon_0 a^2}}[/tex]
[tex] \phi = {\frac {Q}{4 \pi \epsilon_0 a^2}} 2 \pi a h = {\frac{Qh}{2 \epsilon_0}}[/tex]
I plugged in
[tex] Q = \lambda h [/tex]
So I got
[tex] \phi = {\frac{\lambda h^2}{2 \epsilon_0}}[/tex]
And then I again used E.S = phi
[tex] E 2 \pi a h ={\frac{\lambda h^2}{2 \epsilon_0}}[/tex]
[tex] E = {\frac{\lambda h}{4 \pi a \epsilon_0}} [/tex]
It seems a bit too easy and I'm pretty sure that when something like this seems easy, there must be a mistake.
And as to what I think of the differences: through Gauss' law, the length of the wire does not factor into the equation, so it might be okay if we're thinking of some theoretical, endless wire, but for a wire of finite length the method that does not use Gauss' law should be more precise.
The difference between my equation and Gauss' law is that my equation has an added h/2. But why are we only counting with only half the length of the wire I do not know.
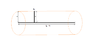
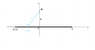
 I'll work on that. :D
I'll work on that. :D 
