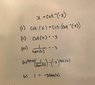Inverse Trig Functions and Reciprocals

[opus]

Homework Statement

Evaluate and express your answer in radians:

$$cot^{-1}\left(1\right)$$

Homework Equations

The Attempt at a Solution

I start by identifying that the domain of Arccotangent is all real numbers. So 1 is in the domain.

From here, I looked at the unit circle and saw that $\frac{π}{4}$ has a cotangent of 1, but this was incorrect.

So I pulled out my calculator and evaluated $\frac{1}{arctan\left(1\right)}$

However, if you'll look at my attached image, the step-by-step instructions say that $cot\left(x\right)=\frac{1}{tan\left(x\right)}$ BUT $arccot\left(x\right)≠\frac{1}{arctan\left(x\right)}$
Why is this true?

I'm not sure what this page is telling me.

 

[jedishrfu]

Think about it for a moment using sine or cosine.

The sin(30) = 0.5 but so does the sin(390) = sin(30 + 360) = sin(30 + n*360) for n=0,1,2,3...

(EDIT: fixed sin(210) typo error)

so now given the sin of an angle you will get a whole bunch of angles with the same sine value.

and so the $cot^{-1} (x) = tan^{-1}(1/x)$

(EDIT:$arccot$ is often written as $cot^{-1}$ in math books implying an inverse but not a multiplicative inverse.)

In your case, they're warning you not to make that mistake in general considering the x=1 and 1/1 is 1.

 

[opus]

Ok so in terms of all the values that a function can take on, I understand that there is more than one angle that can produce a specific value. This is why we restrict the domain of the inverse trig functions- to make them invertible.

So in terms of reciprocals, the cotangent function is equal to the reciprocal of the tangent function. But the page says that the inverse cotangent function is NOT equal to the reciprocal of the inverse tangent function. I guess I still don't see why the inverses cannot be reciprocals but the basic functions can. The only difference is that for the inverses, they have a restricted domain.

 

[Mark44]

This is a terrrible problem, at least from what I can see in the screen shot you posted. At the beginning, it asks you to evaluate $\cot^{-1}(1)$ rounding the answer to four decimal places. The exact answer is $\frac \pi 4$, or rounded, it is approx. .7854.

The answer they show at the bottom of the screen shot is not the answer to the question above, which is at least misleading.

If $x = \cot^{-1}(1)$
then $\cot(x) = 1$
so $\tan(x) = 1$, and it is this 1 that they expect you to put in the box, at least based on what they show is the correct answer.

However that is not the value of $\cot^{-1}(1)$ -- the equation $\tan(x) = 1$ is just an intermediate step.

 

[opus]

Getting tan by itself as not a reciprocal is giving me trouble as well. Say it wasn't a 1, but rather a -3. Then $\frac{1}{tan\left(x\right)}=-3$
How do I go about getting tan(x) rather than just the reciprocal? I would say multiply both sides by tan(x), but that would leave me with $1=-3tan\left(x\right)$

 

[Mark44]

Getting tan by itself as not a reciprocal is giving me trouble as well. Say it wasn't a 1, but rather a -3. Then $\frac{1}{tan\left(x\right)}=-3$
How do I go about getting tan(x) rather than just the reciprocal? I would say multiply both sides by tan(x), but that would leave me with $1=-3tan\left(x\right)$

Sure that would work, but then divide both sides by -3, so tan(x) = -1/3.

Something simpler:
1/tan(x) = -3
=> tan(x) = -1/3
Just take the reciprocal of each side.

If a = b, then 1/a = 1/b, provided that neither a nor b is zero.

 

[opus]

As an example to my question, I wrote out my train of thought here.

 

[opus]

Ahh ok. I see. The 1 in the tangent problem threw me off because 1tan(x) is just tan(x) and I was assuming the coefficient in front of the function should be 1.

 

[opus]

So here is what is still confusing me, and I'll throw in a new but similar problem because I've been trying different ones trying to get it to click:

$sec^{-1}\left(3\right)$

Right off the bat, I see if it's in the restricted domain, and it is.

Next, I think if there are any common angles that I know that would give a secant value of 3. There are none.

Now I know that I have to pop out the calculator. What I want to do (and what I apparently am not allowed to do) is plug $\frac{1}{arccos\left(3\right)}$ into the calculator. This is where I am confused, as I don't know why this is not allowed.

If $sec\left(x\right)=\frac{1}{cos\left(x\right)}$ why is it also NOT true that $arcsec\left(x\right)=\frac{1}{arccos\left(x\right)}$ ?

Why would I have to even put this in terms of cosine?

 

[jedishrfu]

There are some videos under the topic inverse functions here at mathispower4u that will give you a better understanding of how to work with them.

http://www.mathispower4u.com/trigonometry.php

 

[opus]

Thank you jedishrfu. I'll have a look!

 

[opus]

Just found on that website a problem almost identical to the last one I posted. Please see attached image. This seems like a contradiction to the screenshot of my first post.

So let me ask this question- Is the following a true statement?
$arcsec\left(3\right)=arccos\left(\frac{1}{3}\right)$

 

[jedishrfu]

$sec(x)=2$ then $1/cos(x)=2$ then $cos(x)=1/2$

$x = sec^{-1}(2)$ and from the second one $x = cos^{-1}(1/2)$

And you can see how it works.

 

[Mark44]

Just found on that website a problem almost identical to the last one I posted. Please see attached image. This seems like a contradiction to the screenshot of my first post.

So let me ask this question- Is the following a true statement?
$arcsec\left(3\right)=arccos\left(\frac{1}{3}\right)$

Here's a drawing to help you out, using a right triangle.

cos(a) = 1/3, so sec(a) = 3
From this, $a = \sec^{-1}(3) = \text{arcsec}(3)$
From cos(a) = 1/3, we also have $a = \cos^{-1}(\frac 1 3) = \text{arccos}(\frac 1 3)$
Since a is equal to itself, we have $\text{arcse}c(3) = \text{arccos}(\frac 1 3)$
So yeah, yours is a true statement.

BTW, the third side's length is $\sqrt 8 = 2\sqrt{2}$, but it doesn't enter the picture with these functions.

 

[opus]

That makes great sense thinking about it like that! Thanks Mark!

 

[opus]

Thanks guys for the help. I think I got a grip on it now.