- #1
DocZaius
- 365
- 11
For fun, I thought I would try to derive the moment of inertia of a square using different approaches (in each case, changing the differential area being integrated). Everything went well until I tried the approach of first considering the disk in the center of the square, then adding the bits at the corners.
Consider a square with sides of length L. The moment of inertia of the disk of radius L/2 in the center is:
[tex]I_{disk}=\frac{1}{2}MR^2=\frac{1}{2}M({\frac{L}{2}})^2=\frac{1}{8}ML^2[/tex]
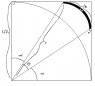
Now consider a corner of the square (attached image). If we figure out the contribution to the moment of inertia of that corner, we can account for the others by multiplying it by 4 because of radial symmetry. We will take arcs with differential thickness from the edge of the circle to the very corner of the square and add up their contributions to the moment of inertia. Here is the formula for the differential area:
[tex]
\cos(\alpha)=\frac{\frac{L}{2}}{r}\\
\theta=\frac{\pi}{2}-2\alpha\\
\theta=\frac{\pi}{2}-2\arccos(\frac{L}{2r})\\
\\
da=r\,\theta\,dr\\
da=r(\frac{\pi}{2}-2\arccos(\frac{L}{2r}))dr
[/tex]
Now the integral for the contribution of the corner to the moment of inertia is:
[tex]
\rho=\frac{M}{A}=\frac{M}{L^2}=\frac{dm}{da}\\
dm=\rho\,da=\rho \, r(\frac{\pi}{2}-2\arccos(\frac{L}{2r}))dr\\
I_{corner}=\int_M r^2 \, dm=\int_{\frac{L}{2}}^{\frac{\sqrt{2}}{2}L} r^2 \, \rho \, r(\frac{\pi}{2}-2\arccos(\frac{L}{2r}))dr\\
[/tex]
Which leads to the following for the total moment of inertia:
[tex]
I_{square}=I_{disk}+4\,I_{corner}=\frac{1}{8}ML^2+4 \int_{\frac{L}{2}}^{\frac{\sqrt{2}}{2}L} r^2 \, \rho \, r(\frac{\pi}{2}-2\arccos(\frac{L}{2r}))dr
[/tex]
My problem is that the integral for the moment of inertia of a corner does not give me what it should ([itex]\frac{1}{96}ML^2[/itex]) but rather gives me something with pi in it. I cannot figure out at what point I went wrong. Can someone see the error?
Thanks.
edit: Despite this not being homework, I realize it might belong in the homework forum. I wasn't sure and went with this forum. Mods, please move it if it needs to be moved.
Consider a square with sides of length L. The moment of inertia of the disk of radius L/2 in the center is:
[tex]I_{disk}=\frac{1}{2}MR^2=\frac{1}{2}M({\frac{L}{2}})^2=\frac{1}{8}ML^2[/tex]
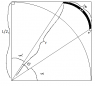
Now consider a corner of the square (attached image). If we figure out the contribution to the moment of inertia of that corner, we can account for the others by multiplying it by 4 because of radial symmetry. We will take arcs with differential thickness from the edge of the circle to the very corner of the square and add up their contributions to the moment of inertia. Here is the formula for the differential area:
[tex]
\cos(\alpha)=\frac{\frac{L}{2}}{r}\\
\theta=\frac{\pi}{2}-2\alpha\\
\theta=\frac{\pi}{2}-2\arccos(\frac{L}{2r})\\
\\
da=r\,\theta\,dr\\
da=r(\frac{\pi}{2}-2\arccos(\frac{L}{2r}))dr
[/tex]
Now the integral for the contribution of the corner to the moment of inertia is:
[tex]
\rho=\frac{M}{A}=\frac{M}{L^2}=\frac{dm}{da}\\
dm=\rho\,da=\rho \, r(\frac{\pi}{2}-2\arccos(\frac{L}{2r}))dr\\
I_{corner}=\int_M r^2 \, dm=\int_{\frac{L}{2}}^{\frac{\sqrt{2}}{2}L} r^2 \, \rho \, r(\frac{\pi}{2}-2\arccos(\frac{L}{2r}))dr\\
[/tex]
Which leads to the following for the total moment of inertia:
[tex]
I_{square}=I_{disk}+4\,I_{corner}=\frac{1}{8}ML^2+4 \int_{\frac{L}{2}}^{\frac{\sqrt{2}}{2}L} r^2 \, \rho \, r(\frac{\pi}{2}-2\arccos(\frac{L}{2r}))dr
[/tex]
My problem is that the integral for the moment of inertia of a corner does not give me what it should ([itex]\frac{1}{96}ML^2[/itex]) but rather gives me something with pi in it. I cannot figure out at what point I went wrong. Can someone see the error?
Thanks.
edit: Despite this not being homework, I realize it might belong in the homework forum. I wasn't sure and went with this forum. Mods, please move it if it needs to be moved.
Attachments
Last edited: