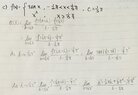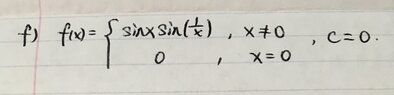Need help, are these functions differentiable?

[FallArk]

View attachment 6498
View attachment 6499

I want to figure out whether the functions are differentiable at c. I think I should use some of the trig identities, but I'm not sure which ones. Any tips?

 

[I like Serena]

I want to figure out whether the functions are differentiable at c. I think I should use some of the trig identities, but I'm not sure which ones. Any tips?

Hey FallArk! (Smile)

Let's start with the proper definition of the derivative.
That is:
$$f(x)=\begin{cases}\tan x &\text{if } -\frac\pi 2 \le x <\frac \pi 3 \\
x^2 & \text{if } x \ge \frac \pi 3
\end{cases} \quad\Rightarrow\quad
f'(\frac\pi 3) = \lim_{h\to 0} \frac{f(\frac\pi 3+h)-f(\frac\pi 3)}{h}
$$
Now let's look at the lower limit:
$$\lim_{h\to 0^-} \frac{f(\frac\pi 3+h)-f(\frac\pi 3)}{h}
= \lim_{h\to 0^-} \frac{\tan(\frac\pi 3+h)-(\frac\pi 3)^2}{h}
\to \frac{\sqrt 3 - \frac{\pi^2}{9}}{0^-} = -\infty
$$
It's not convergent is it? (Wondering)

 

[Euge]

Hi FallArk,

The function in part (c) is not differentiable at $x = c$, for it is discontinuous at that point. Just check the one-sided limits.

The function in part (f), although continuous at $x = c$, is not differentiable at $x = c$; the limit $\lim\limits_{x\to 0} \dfrac{f(x)}{x}$ does not exist. Indeed, consider the sequences $x_n = \dfrac{2}{(4n+1)\pi}$ and $y_n = \dfrac{2}{(4n-1)\pi}$. Then $x_n, y_n \to 0$, but $$\lim\limits_{n\to \infty} \frac{f(x_n)}{x_n} = 1 \neq -1 = \lim\limits_{n\to \infty} \frac{f(y_n)}{y_n}$$

 

[FallArk]

Hey FallArk! (Smile)

Let's start with the proper definition of the derivative.
That is:
$$f(x)=\begin{cases}\tan x &\text{if } -\frac\pi 2 \le x <\frac \pi 3 \\
x^2 & \text{if } x \ge \frac \pi 3
\end{cases} \quad\Rightarrow\quad
f'(\frac\pi 3) = \lim_{h\to 0} \frac{f(\frac\pi 3+h)-f(\frac\pi 3)}{h}
$$
Now let's look at the lower limit:
$$\lim_{h\to 0^-} \frac{f(\frac\pi 3+h)-f(\frac\pi 3)}{h}
= \lim_{h\to 0^-} \frac{\tan(\frac\pi 3+h)-(\frac\pi 3)^2}{h}
\to \frac{\sqrt 3 - \frac{\pi^2}{9}}{0^-} = -\infty
$$
It's not convergent is it? (Wondering)

I was so concentrated on getting rid of the h, I did not even see that I can just evalute it. Thanks!

- - - Updated - - -

Hi FallArk,

The function in part (c) is not differentiable at $x = c$, for it is discontinuous at that point. Just check the one-sided limits.

The function in part (f), although continuous at $x = c$, is not differentiable at $x = c$; the limit $\lim\limits_{x\to 0} \dfrac{f(x)}{x}$ does not exist. Indeed, consider the sequences $x_n = \dfrac{2}{(4n+1)\pi}$ and $y_n = \dfrac{2}{(4n-1)\pi}$. Then $x_n, y_n \to 0$, but $$\lim\limits_{n\to \infty} \frac{f(x_n)}{x_n} = 1 \neq -1 = \lim\limits_{n\to \infty} \frac{f(y_n)}{y_n}$$

Thanks! I get it now

 

[HallsofIvy]

While the derivative, f', of a differentiable function, f, is not necessarily continuous, it does satisfy the "intermediate value property" (f'(x), for x between a and b, takes on all values between f'(a) and f'(b)). In particular, the two limits, $$\lim_{x\to a^-} f'(x)$$ and $$\lim_{x\to a^+} f'(x)$$ must be equal.