Need help understanding "Electrostatic Boundary Conditions"

[NicolaiTheDane]

We are using griffith's 4 edition in my electromagnetic course atm. and there's something I just don't understand about boundary conditions.

It says that if we have a surface charge, and we put a pillbox on it, in such a way that half of it extends under the surface charge, and the other half extends above it. That is the top and buttom are parallel with the surface charge.

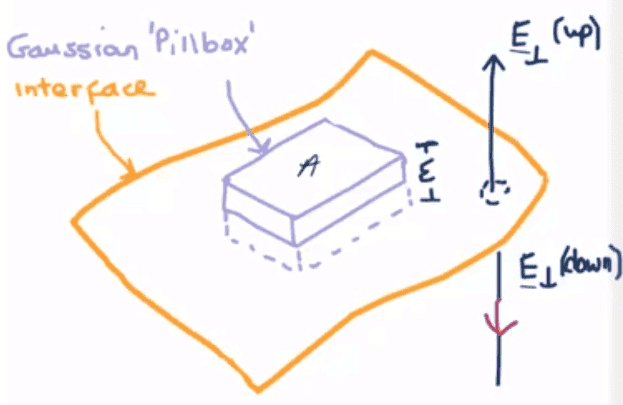
The the book says, to which I agree, that Gausses law tells us:

$$\oint \vec E \cdot d \vec a=\frac{1}{\epsilon_0} \cdot Q_{enc}=\frac{1}{\epsilon_0} \cdot \sigma \cdot A$$

where A = lid of the pillbox. However then it says that if the side height $\epsilon_0$ of the pillbox, which are running perpendicular to the surface charge, goes to zero, we get the following:

$$E^{\perp} _\rm{above} - E^{\perp} _\rm{below} = \frac{1}{\epsilon_0} \cdot \sigma$$

This I don't understand. If $\epsilon_0 \rightarrow 0$ then how does that change anything? If it hits 0, then the top and bottom are inside the surface charge, and the equations break down (as we cannot be inside the charge, at least not that i have learned yet). If it doesn't hit 0, then we still have the two surfaces, which are still the same size, and the result should be:

$$E^{\perp} _\rm{above} - E^{\perp} _\rm{below} = \left(\frac{1}{\epsilon_0} \cdot \sigma \cdot A \right) - \left(-\frac{1}{\epsilon_0} \cdot \sigma \cdot A\right) = \frac{2}{\epsilon_0} \cdot \sigma \cdot A$$

(I'm assuming ofc the field has different signs on each side. Otherwise it'd be zero instead)

Now when I say "should", I know I'm wrong. There is something about limits that I'm forgetting here I'm sure (which is embarrassing as I'm working with infinite series in another course atm), but what is it that I'm missing here?

 

[SchroedingersLion]

Hmm... I don't really understand his reasoning with $\epsilon \rightarrow 0$ either.

However, his second equation is simply the first equation divided by A.
You can rewrite the first integral into two parts: one surface integral over the "upper A", and, ADDED to it (not substracted), the surface integral over the "lower A".
The E's are parallel to the normalvectors of the A's, so the scalar products in the integrands is simply the product of the absolute values. That means you can pull the E's out of the two integrals (one is $E_{above}$, the other is $E_{below}$). Then each of the integrations only give A. The result of the total integration is the right hand side of your first equation. You divide the whole thing by A, et voila, you arrive at your second equation.

edit: even though that doesn't seem to explain the "-" on the lefthand side of eq. 2. Someone else might have a better answer.

edit2: Ahh, wait a second. If $\epsilon \rightarrow 0$, it means we only have one surface A with a single normal vector that we choose to point upwards. If you now do what I explained above, you will get the minus because the A and the $E_{below}$ vector are antiparallel now.

 

[NicolaiTheDane]

Hmm... I don't really understand his reasoning with $\epsilon \rightarrow 0$ either.

However, his second equation is simply the first equation divided by A.
You can rewrite the first integral into two parts: one surface integral over the "upper A", and, ADDED to it (not substracted), the surface integral over the "lower A".
The E's are parallel to the normalvectors of the A's, so the scalar products in the integrands is simply the product of the absolute values. That means you can pull the E's out of the two integrals (one is $E_{above}$, the other is $E_{below}$). Then each of the integrations only give A. The result of the total integration is the right hand side of your first equation. You divide the whole thing by A, et voila, you arrive at your second equation.

edit: even though that doesn't seem to explain the "-" on the lefthand side of eq. 2. Someone else might have a better answer.

Ofcourse! The E's in the second equation aren't vectors! I keep failing to notice these things, which makes electromagnetism a real pain. But yea I see it now! I still don't understand the significance of this, even though I my lecturer told us it is crucial. Perhaps I'll figure it out in due time.

 

[SchroedingersLion]

Hey, see my second edit, the minus sign is easily explained as well :D

 

[vanhees71]

I don't understand how one can get to the 3rd equation in #1. Of course the 2nd one is right, and you gave perfectly well the correct reasoning by quoting #1.

If you have an ideal(ized) surface charge, no matter how small $\epsilon$ might get, you always get $\sigma A/\epsilon_0$ on the right-hand side of Gauß's Law for the electric field. The left-hand side is also easily evaluated for $\epsilon \rightarrow 0$, leading to
$$\vec{A}_{\text{up}} \cdot \vec{E}_{\text{up}}+\vec{A}_{\text{down}} \cdot \vec{E}_{\text{down}}=A \sigma/\epsilon_0.$$
Now for $\epsilon \rightarrow 0$ $\vec{A}_{\text{down}}=-\vec{A}_{\text{up}}=-\vec{n}_{\text{up}} A,$
and thus
$$A \vec{n}_{\text{up}} \cdot (\vec{E}_{\text{up}}-\vec{E}_{\text{down}})=A \sigma/\epsilon_0$$
and thus
$$\text{Div} \vec{E}=\sigma/\epsilon_0,$$
i.e., the "jump divergence" of $\vec{E}$ is just the surface-charge density modulo the SI conversion factor $1/\epsilon_0$.

 

[NicolaiTheDane]

I don't understand how one can get to the 3rd equation in #1. Of course the 2nd one is right, and you gave perfectly well the correct reasoning by quoting #1.

If you have an ideal(ized) surface charge, no matter how small $\epsilon$ might get, you always get $\sigma A/\epsilon_0$ on the right-hand side of Gauß's Law for the electric field. The left-hand side is also easily evaluated for $\epsilon \rightarrow 0$, leading to
$$\vec{A}_{\text{up}} \cdot \vec{E}_{\text{up}}+\vec{A}_{\text{down}} \cdot \vec{E}_{\text{down}}=A \sigma/\epsilon_0.$$
Now for $\epsilon \rightarrow 0$ $\vec{A}_{\text{down}}=-\vec{A}_{\text{up}}=-\vec{n}_{\text{up}} A,$
and thus
$$A \vec{n}_{\text{up}} \cdot (\vec{E}_{\text{up}}-\vec{E}_{\text{down}})=A \sigma/\epsilon_0$$
and thus
$$\text{Div} \vec{E}=\sigma/\epsilon_0,$$
i.e., the "jump divergence" of $\vec{E}$ is just the surface-charge density modulo the SI conversion factor $1/\epsilon_0$.

You get there by being a derp frankly. Its clearly impossible to get from the #1 to the #2 equations, and honestly can't even see how I got there myself anymore. Sometimes I completely **** up when I view equation steps that I haven't made myself, and I get stuck in my error. As soon as SchroedingersLion made his post, I saw my error. When I get stuck I found that doing the steps myself helps a lot, though I still get suck now and then. Vector functions, and vector math in general, doesn't come as fluently to me yet. Actually working problems helps a lot more then read in my case.

 

[vanhees71]

Yes, that's the trick. Do a lot of calculations, and particularly vector calculus takes time to get used to. For me the most difficult theory lecture was electrodynamics because all the vector calculus stuff. A very nice book with a lot of examples and worked problems is

Schaum's Outline, Vector Analysis, M. Spiegel, McGraw (1959)

 

[NicolaiTheDane]

Yes, that's the trick. Do a lot of calculations, and particularly vector calculus takes time to get used to. For me the most difficult theory lecture was electrodynamics because all the vector calculus stuff. A very nice book with a lot of examples and worked problems is

Schaum's Outline, Vector Analysis, M. Spiegel, McGraw (1959)

Thanks I'll look into that for sure. Had a primer on the eksamen in 10 weeks, and I couldn't even understand the problems presented. Well I could understand them, but I hadn't got a clue on where to start. I have had hard times a university, but this might be the subject that breaks me