- #1
Juanda
- 292
- 100
- TL;DR Summary
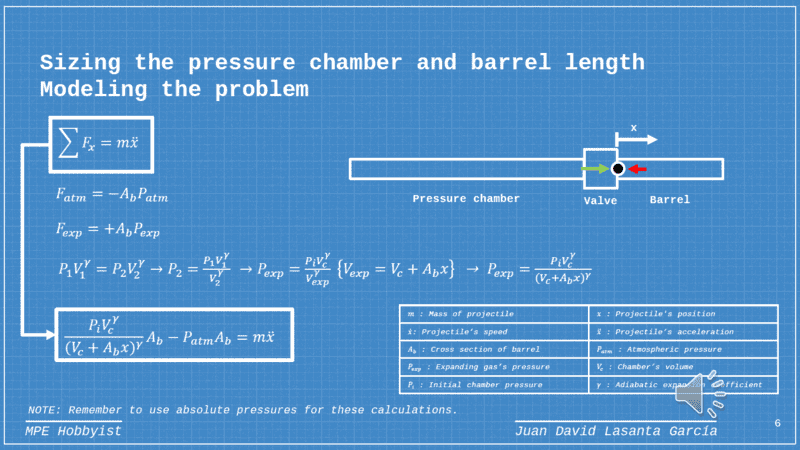
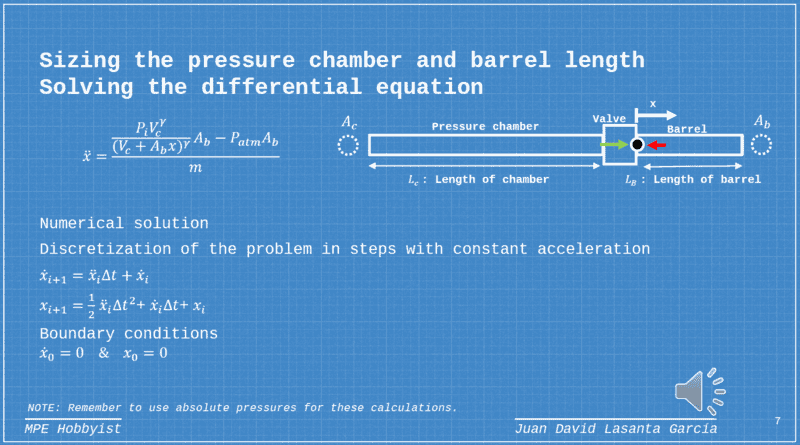
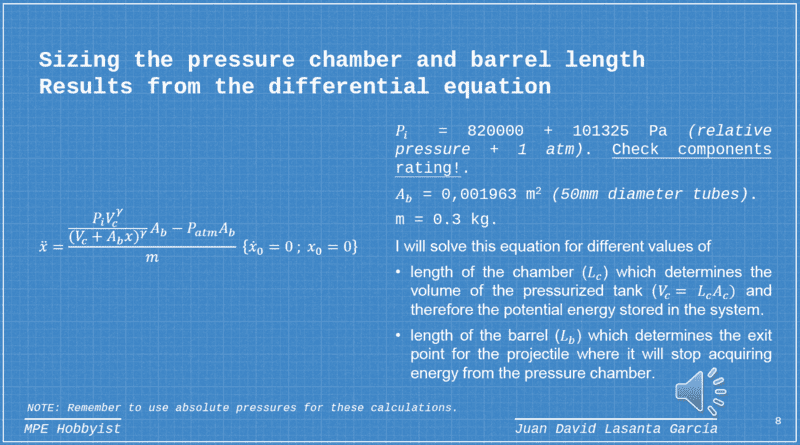
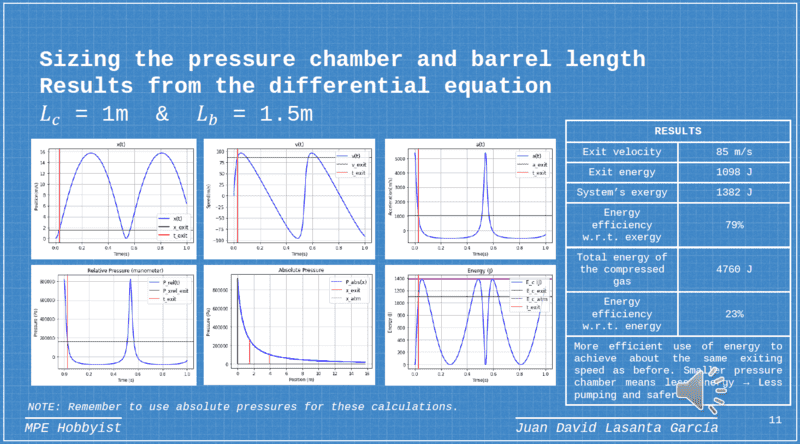
- Derivation of the optimum ratio for the volume chamber and the cannon chamber.
I was checking bait cannons and potato guns on the internet because they are fun. Maybe one day I'll build my own.
First of all, these cannons use multiple sources of energy (combustion using hair spray, dry ice, etc.). I'll just consider compressed air cannons because I think they are the most reliable option.
There are plenty of videos detailing the process to make them but I couldn't find much information about what size you should make the barrel for a given pressure chamber. Some people provide rules of thumb for it or experiments for a given cannon and things like that. I tried to solve the generic math to be able to adapt it to any size.
I created a PPT with the analysis.
Some people prefer reading because it's faster so I attached the slides too as a PDF.
As a summary, here are a few key slides:
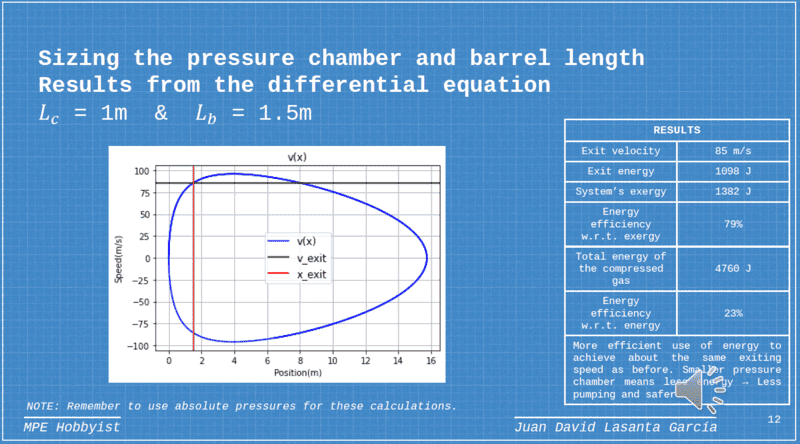
I hope you found that interesting and maybe we can have some cool conversations about it.
First of all, these cannons use multiple sources of energy (combustion using hair spray, dry ice, etc.). I'll just consider compressed air cannons because I think they are the most reliable option.
There are plenty of videos detailing the process to make them but I couldn't find much information about what size you should make the barrel for a given pressure chamber. Some people provide rules of thumb for it or experiments for a given cannon and things like that. I tried to solve the generic math to be able to adapt it to any size.
I created a PPT with the analysis.
Some people prefer reading because it's faster so I attached the slides too as a PDF.
As a summary, here are a few key slides:
- Do you see any fundamental errors in the derivation?
- How far away from reality do you think are the simplifications?
- Ideal gas behavior
- Adiabatic expansion
- No friction
- Perfect seal
- Instantaneous valve opening time
- Would you model the system differently?
- Since the energy in the system comes from the internal energy in the ideal gas, through energy conservation I should be able to find the exiting velocity with ##\Delta U_i=m_{air}c_v\Delta T##. However, at no point, I defined the temperature of the gas or the amount of gas present. I feel I should be able to obtain all the information about the gas with the given data but I'm missing how.
- From the equations, I could technically achieve supersonic speeds by either increasing the initial pressure or the size of the pressure chamber. However, I am pretty sure that is not how it works. Do you recommend any particular book to be able to understand that scenario? Is it possible to get away with this while using regular air and simple geometries? It's not like I'm going to build such a thing but it'd be cool to consider the case.
I hope you found that interesting and maybe we can have some cool conversations about it.