- #1
kravky
- 22
- 3
Hello,
in every book and on every website (e.g. here http://farside.ph.utexas.edu/teaching/315/Waves/node13.html) i found for driven harmonic osciallation the same solution for phase angle:θ=atan(ωb/(k−mω^2)) where ω is driven freq., m is mass, k is spring constant. I agree with it =it follows from solution of diff.eq.of motion
But everywhere i see the same graph for phase angle as a function of ω where phase angle θ goes fromθ=0 when ω=0 thru θ=pi/2 whenω=ω0=resonance, to θ=pi when ω is infinitely large.
How is it possible for phase angle θ be more than pi/2 if θ=atan(ωb/(k−mω^2))
Is it not arctan and is it not limited from -pi/2 to pi/2 ?
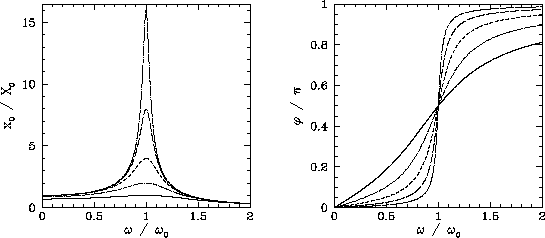
here u can see the classical picture (second one - right one). phase angle there is phi instead of theta (just another symbol but same meaning) and u can see it in dimensionless values. left picture is amplitude as a function of ω. IT makes sense. But i don't understand the second one.
Also here: http://lampx.tugraz.at/~hadley/physikm/apps/resonance.en.php. This formula does not work.
it has to be sth else that pure arctang. it is ok for x greater than 0 (arctg(x)) but for x less then 0 this forumla doesn't work. it has to be like θ=pi-atan(ωb/(k−mω^2))
I think it cannot be pure arctan. it has to be theta=pi - atan(ωb/(k−mω^2)) for argument (ωb/(k−mω^2)) less than zero. But i don't know why.
What is worng with that? Simply: arctan has range from -pi/2 to pi/2. So i am really confused how it can be pi according to books and websites.
*phase angle=angle by which the driving force leads the displacement of the system
in every book and on every website (e.g. here http://farside.ph.utexas.edu/teaching/315/Waves/node13.html) i found for driven harmonic osciallation the same solution for phase angle:θ=atan(ωb/(k−mω^2)) where ω is driven freq., m is mass, k is spring constant. I agree with it =it follows from solution of diff.eq.of motion
But everywhere i see the same graph for phase angle as a function of ω where phase angle θ goes fromθ=0 when ω=0 thru θ=pi/2 whenω=ω0=resonance, to θ=pi when ω is infinitely large.
How is it possible for phase angle θ be more than pi/2 if θ=atan(ωb/(k−mω^2))
Is it not arctan and is it not limited from -pi/2 to pi/2 ?
here u can see the classical picture (second one - right one). phase angle there is phi instead of theta (just another symbol but same meaning) and u can see it in dimensionless values. left picture is amplitude as a function of ω. IT makes sense. But i don't understand the second one.
Also here: http://lampx.tugraz.at/~hadley/physikm/apps/resonance.en.php. This formula does not work.
it has to be sth else that pure arctang. it is ok for x greater than 0 (arctg(x)) but for x less then 0 this forumla doesn't work. it has to be like θ=pi-atan(ωb/(k−mω^2))
I think it cannot be pure arctan. it has to be theta=pi - atan(ωb/(k−mω^2)) for argument (ωb/(k−mω^2)) less than zero. But i don't know why.
What is worng with that? Simply: arctan has range from -pi/2 to pi/2. So i am really confused how it can be pi according to books and websites.
*phase angle=angle by which the driving force leads the displacement of the system




