- #1
Math Amateur
Gold Member
MHB
- 3,990
- 48
I am reading David S. Dummit and Richard M. Foote : Abstract Algebra ...
I am trying to understand the proof of Proposition 37 in Section 13.5 Separable and Inseparable Extensions ...The Proposition 37 and its proof (note that the proof comes before the statement of the Proposition) read as follows:

In the above text by D&F we read the following:
"If ##p(x)## were inseparable then we have seen that ##p(x) = q(x^p)## for some polynomial ##q(x) \in \mathbb{F} [x]##. ... ... "I cannot understand exactly why this is true ...Can someone please explain exactly why, as D&F assert, the following is true ... ...##p(x)## inseparable ## \ \ \Longrightarrow \ \ p(x) = q(x^p)## for some polynomial ##q(x) \in \mathbb{F} [x]##I cannot find where D&F establish exactly this implication ... but maybe there is some idea in the proof of Corollary 34 which reads as follows:
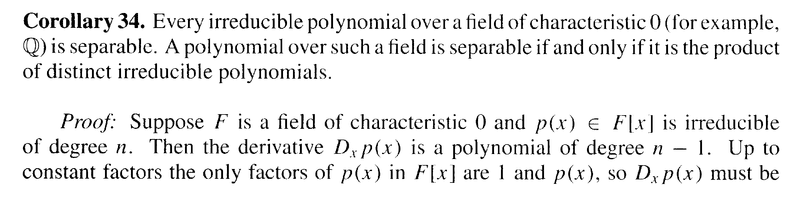
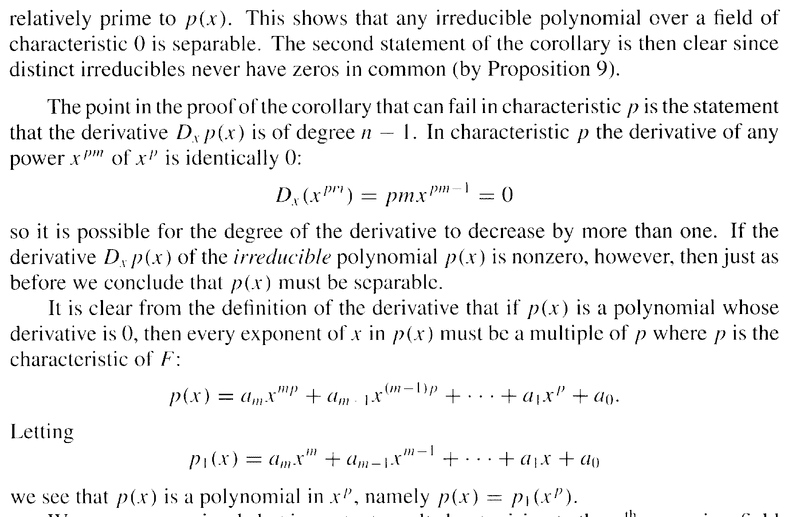
Corollary 36 may also be relevant ... so I am providing that as well ... as follows:
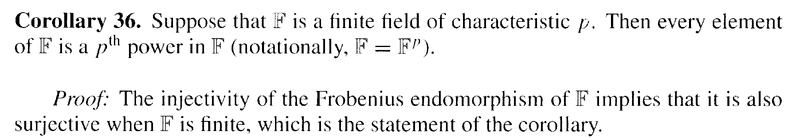
Hope someone can help explain the assertion by D&F mentioned above ..
Peter
I am trying to understand the proof of Proposition 37 in Section 13.5 Separable and Inseparable Extensions ...The Proposition 37 and its proof (note that the proof comes before the statement of the Proposition) read as follows:
In the above text by D&F we read the following:
"If ##p(x)## were inseparable then we have seen that ##p(x) = q(x^p)## for some polynomial ##q(x) \in \mathbb{F} [x]##. ... ... "I cannot understand exactly why this is true ...Can someone please explain exactly why, as D&F assert, the following is true ... ...##p(x)## inseparable ## \ \ \Longrightarrow \ \ p(x) = q(x^p)## for some polynomial ##q(x) \in \mathbb{F} [x]##I cannot find where D&F establish exactly this implication ... but maybe there is some idea in the proof of Corollary 34 which reads as follows:
Corollary 36 may also be relevant ... so I am providing that as well ... as follows:
Hope someone can help explain the assertion by D&F mentioned above ..
Peter
Attachments
Last edited:

