Stokes' Theorem and Curvature on a Torus

In summary: The two vertical edges seem identified but the top and bottom do not. If so, then it is a case of Stokes Theorem because the boundary of this cylinder is ##C_1-C_2## or ##C_2-C_1## depending on the orientation.
  • #1
lichen1983312
85
2
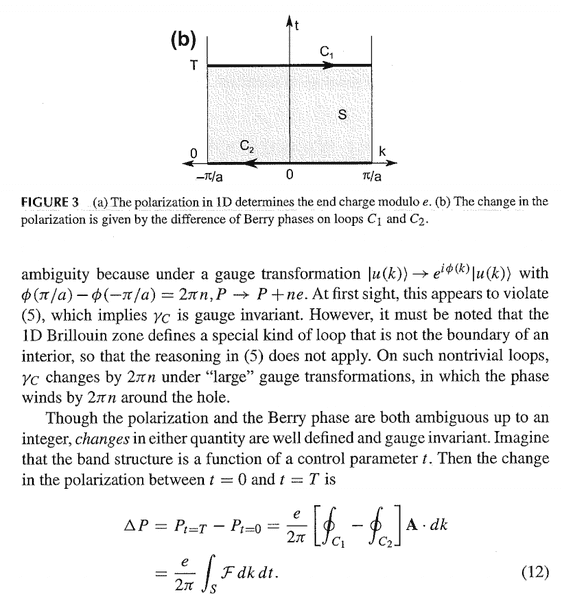
I am now looking at a physics problem that should be a use of stokes' theorem on a torus. The picture (b) here is a torus that the upper and bottom sides are identified as the same, so are the left and right sides. ##A## is a 1-form and ##F = dA## is the corresponding curvature. As is shown in the equation, the author says the integration of ##F## over the whole torus is the same thing as the difference between the two line integral along C1 and C2. Is this a case of stokes' theorem? I don't understand how C1 and C2 is the boundary of S. Please help.
Untitled-1.png
 
Physics news on Phys.org
  • #2
Notice they using radial measure on the horizontal axis and they mention that the difference is ##2\pi##

That means C1 is a closed loop and C2 is a closed loop.

Does that make sense?
 
  • #3
jedishrfu said:
Notice they using radial measure on the horizontal axis and they mention that the difference is ##2\pi##

That means C1 is a closed loop and C2 is a closed loop.

Does that make sense?
Sorry for the confusion, the horizontal axis is parameterized with angle, both C1 and C2 are closed loops because the left and right sides are identified.
 
  • #4
So what don't you understand about the boundary of S? It's like a ring with C2 as the outer boundary and C1 as the inner boundary.

When they plot it using radial measure S looks like a rectangle.
 
  • #5
So this is not a torus? I though the upper side and bottom side are identified as the same edge.
 
  • #6
Stokes theorem for torus: ##\int_{\mathbb{T}^m} d\omega=0## :) The torus does not have boundary
 
  • #7
lichen1983312 said:
So this is not a torus? I though the upper side and bottom side are identified as the same edge.

I do no think this is a torus. Rather, it seems to be a cylinder. The two vertical edges seem identified but the top and bottom do not. If so, then it is a case of Stokes Theorem because the boundary of this cylinder is ##C_1-C_2## or ##C_2-C_1## depending on the orientation.

If the top and bottom were also identified then you would have a torus but then the integral of ##dA## would be zero since as zwierz pointed out, the boundary of a torus is empty.

Another way to think of it is that if ##C_1## and ##C_2## are identified to make a torus then the integrals of ##A## along them would be the same except for a sign and would cancel out to give zero.
 
Last edited:
  • #8
Hi guys, Thanks for the help, I think it really is a ring not torus.
 

1. What is Stokes' theorem on a torus?

Stokes' theorem on a torus is a mathematical theorem that relates the surface integral of a vector field over a surface on a torus to the line integral of the same vector field along the boundary of the surface. It is a generalization of the classical Stokes' theorem in three-dimensional space.

2. How is Stokes' theorem on a torus applied in real-world situations?

Stokes' theorem on a torus has many practical applications in physics and engineering. It is often used to calculate the circulation of a fluid or the work done by a force field on a rotating object. It is also used in electromagnetism to calculate the magnetic flux through a closed loop on a torus.

3. What are the key components of Stokes' theorem on a torus?

The key components of Stokes' theorem on a torus are a surface on a torus, a vector field defined on that surface, and a closed curve that bounds the surface. The theorem states that the surface integral of the curl of the vector field over the surface is equal to the line integral of the vector field along the boundary curve.

4. Can Stokes' theorem be extended to higher dimensions?

Yes, Stokes' theorem can be extended to higher dimensions. In fact, it is a special case of a more general theorem called the generalized Stokes' theorem, which applies to manifolds of any dimension. However, the specific application of Stokes' theorem on a torus is limited to three-dimensional space.

5. What is the significance of the torus in Stokes' theorem?

The torus is a unique geometric shape that has both a boundary curve and a surface, making it an ideal object for demonstrating the relationship between surface integrals and line integrals in Stokes' theorem. It also has applications in various fields, such as fluid mechanics and electromagnetism, making it a useful tool in solving real-world problems.

Similar threads

A About Chern number of U(1) principal bundle on a torus
    • Differential Geometry
Replies
28
Views
5K
A Can I find a smooth vector field on the patches of a torus?
    • Differential Geometry
Replies
4
Views
2K
Understanding the math of definition of electromotive force
    • Introductory Physics Homework Help
Replies
1
Views
138
Singularities when applying Stokes' theorem
    • Calculus and Beyond Homework Help
Replies
2
Views
1K
I Any surface bounded by the same curve in Stokes' theorem
    • Calculus
Replies
6
Views
1K
I Stokes Theorem: Vector Integral Identity Proof
    • Calculus
Replies
2
Views
2K
Evaluating ∫cF⋅dr Using Stokes' Theorem
    • Calculus and Beyond Homework Help
Replies
2
Views
1K
B Preferred directions on a torus?
    • Topology and Analysis
Replies
8
Views
2K
Applying Stoke's theorem on an s,φ,z vector when the given shape is in x,y,z
    • Calculus and Beyond Homework Help
Replies
20
Views
2K
Faraday's Law and Stokes Theorem
    • Calculus and Beyond Homework Help
Replies
1
Views
2K

Hot Threads

Recent Insights

Back
Top