Visual effect in special relativity

[andy95220]

I have an exercise:
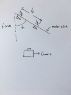
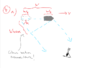
A distant camera snaps a photograph of a speeding bullet (speed v) with length b in its rest frame. Behind the bullet and parallel to its path is a meter stick, at rest with respect to the camera. The direction to the camera is an angle $\alpha$ from the direction of the bullet’s velocity. What will be the apparent length of the bullet as seen in the photo? (That is, how much of the meter stick is hidden?)
If I understand it correctly, the idea of the question should go like this:

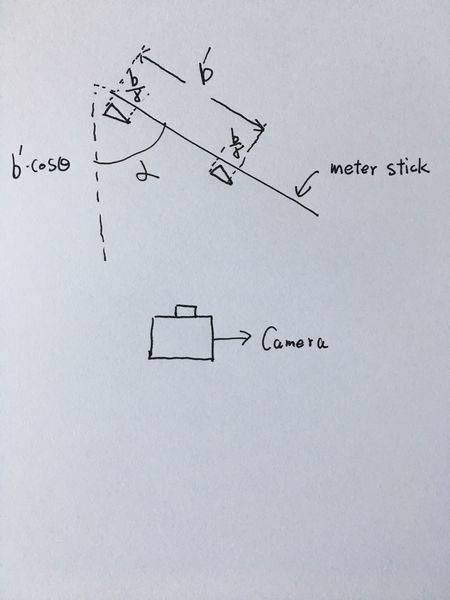
So the problem is how to find $b'$ as the hidden length?
To solve for the hidden length we should know the time for the shutter I think, however, this is not given in the problem. How do I solve for it?

 

[robphy]

Since this is a homework problem, you should provide more of an attempt toward solving this... [like at SE].

 

[Nugatory]

To solve for the hidden length we should know the time for the shutter I think, however, this is not given in the problem.

You've identified a weakness in this problem: as phrased it's somewhat ambiguous what's being asked, what exactly it means to capture an image, and how to handle the effects of shutter duration.

One way of interpreting the problem: consider all the lightlike paths between points on the meter stick and a single event (point in space and time) at the shutter of the camera. Which of these paths intersect the bullet? That will tell you which part of the stick is obscured by the bullet in an ideal image taken by an ideal camera with infinite shutter speed and film sensitivity.

 

[PAllen]

Another ambiguity in the question is whether you are taking the picture when the bullet is at angle alpha in the camera rest frame, or when you can receive the light from when the bullet was at angle alpha, in the camera frame. They are two different pictures. I would guess they mean the second, but the problem should really specify. Yet another ambiguity is whether the angle alpha is for the front, middle or back of the bullet. They are three slightly different cases, given that the bullet has a specified finite rest length.

 

[Nugatory]

Another ambiguity in the question is whether...

Ah - right - thanks, that had also bothered me when I first read the post.

With tongue only slightly in cheek, I suggest that this thread is most interesting as an object lesson in just how tricky it can be to properly specify a relativity problem. (And also that OP was right to think that the question was underspecified).

 

[anorlunda]

Can we take that as a challenge to state the problem properly?

 

[andy95220]

Ah - right - thanks, that had also bothered me when I first read the post.

With tongue only slightly in cheek, I suggest that this thread is most interesting as an object lesson in just how tricky it can be to properly specify a relativity problem. (And also that OP was right to think that the question was underspecified).

This is a solution given by my teacher, but I don't understand it.
can anyone help to explain it?
‪

 

[Ibix]

The answer assumes that the camera is so far away that rays of light reaching it from the bullet are very, very nearly parallel. It's also treating the exposure time as negligible. As set up, the tail of the bullet is further from the camera than the nose, so light from the tail takes longer to reach the camera because it has further to go. But in that extra time, the bullet has moved significantly. So some light from the nose that reaches the camera at the same time as some light from the tail set off later, when the bullet was in a different position (the solid outline of the bullet compared to the dotted outline).

The diagram you posted relates the extra time to the bullet speed and angle. I haven't checked the maths.

 

[PAllen]

Later I will write, I think, a clearer and slightly more complete explanation of what your teacher is getting at. But for now, I want to say that it is clear to me that there is a math error. The formula you present does not approach b' = b for slow speeds, independent of angle alpha, as it must do. Correcting algebra, with the teacher's assumptions (I also have a more rigorous formula), the formula should actually be:

b' = b / [γ(1-βcosα)], β=v/c

which does approach b'=b for slow speeds.

 

[robphy]

Note that this problem is
Problem 1.5 from the Problem Book in Relativity and Gravitation (by Lightman, et al.)
https://books.google.com/books?isbn=1400889014

 

[PAllen]

I will give a fuller explanation, using the diagram and most variables as specified in post #7. Note, my guess is that the teacher did not make a mistake, instead the OP made a copying error. Trivial algebra from the equations in #7 leads to the formula I give in #9.

To start with, given the discussion of ambiguities, I will explicitly state those used by the teacher, which were also what Nugatory and I posited as the most likely:

0) All description is in the frame of the camera. Quite interesting things can be discovered also considering the bullet frame, but that is not the current problem (e.g. the whole problem can be reduced to aberration that way, without consideration of any signal travel times. The arguments are quite sophisticated and tricky, but the result is almost no computation to write down the answer, even for much more complex problems than this).
1) The camera is an infinitesimal pinhole camera (no optical issues to consider), with infinite shutter speed, and infinitely sensitive film.
2) It is snapped when the light from when the bullet being located so its line to the camera forms angle α to the velocity of the bullet, arrives at the camera. At this picture capture time, the bullet is far to the right.
3) More specifically, even though an approximation is made, the logic of the argument is based on the angle α being to the back of the bullet. This is the most convenient case to consider when the bullet is approaching, as in the diagram. For the bullet receding, it would be more convenient to consider angle to the front of the bullet. That is, it is most convenient to analyze from the end of the bullet from which the signal takes longer to reach the camera, for emission events simultaneous in the camera frame.
4) The bullet is considered a 1 dimensional object.
5) The ruler is really considered to be a line along which the bullet moves, i.e. there are no issues of distance between the bullet and the infinitely long ruler.

This answers Anorlunda's challenge ad nauseum.

I prefer (within these assumptions) to set up the problem rigorously, then approximate. I find it easier to explain this way. So, in reference to the diagram in #7, we consider a skinny triangle formed by the camera, bullet rear emission event, and the bullet front emission event that arrives at the camera the same time as the rear emission event. The bullet velocity is taken to be horizontal, per that diagram. For now, I take L to be the distance between the front emission event and the camera, b' is the horizontal side of the skinny triangle, and the long side of the triangle is readily seen to be:

L cos θ + b' cos α

where θ is the angle at the camera between the signals from the emission events. Now, if we were to treat this problem without approximation, neither L nor θ are independent variables, as they both depend (in part) on α. Better is to introduce one new variable k, as the bullet's closest approach distance to the camera. Then it can be seen that:

tan θ = b'sin α / (( k/sin α) - b' cos α)
and
L = (( k/sin α) - b' cos α) / cos θ

Thus, we have only variables k, α, and b', so far.

Now, if we consider the time of the rear emission event to be 0, its arrival time at the camera is:

(b'cos α + L cos θ)/c

The forward transmission event must start later (the simultaneous one would have passed the camera before the rear one arrives). I will call the delay in the forward emission event simply t, insteand of introducing deltas or more primes as post #7 does. Then the arrival time of the forward emission event is simply:

t + L/c

We want to equate these, to require simultaneous signal arrival time at the camera, this:

(b'cos α + L cos θ)/c = t + L/c , eqn (1)

Further, we can see the b' is simply given by:

b' = b/γ + vt , eqn (2)

that is, the contracted length of the bullet plus its travel distance from the rear emission event. Given that L and θ can be expressed in terms of k and the other variables, we se that we can solve (implicitly, at least) for b' in terms of k, b and α.

Now, only, we make an approximation. We assume k is large enough that θ is extremely small, so we may take L cos θ to be simply L. Then we have from equation (1):

b' cos α / c = t

Plugging this in (2) immediately gives:b' = b / [γ(1-βcosα)], β=v/c

 

[A.T.]

View attachment 232530
View attachment 232531
This is a solution given by my teacher, but I don't understand it.
can anyone help to explain it?
‪

This might help you to visualize it:
https://www.spacetimetravel.org/bewegung/bewegung3.html

 

[andy95220]

I will give a fuller explanation, using the diagram and most variables as specified in post #7. Note, my guess is that the teacher did not make a mistake, instead the OP made a copying error. Trivial algebra from the equations in #7 leads to the formula I give in #9.

To start with, given the discussion of ambiguities, I will explicitly state those used by the teacher, which were also what Nugatory and I posited as the most likely:

0) All description is in the frame of the camera. Quite interesting things can be discovered also considering the bullet frame, but that is not the current problem (e.g. the whole problem can be reduced to aberration that way, without consideration of any signal travel times. The arguments are quite sophisticated and tricky, but the result is almost no computation to write down the answer, even for much more complex problems than this).
1) The camera is an infinitesimal pinhole camera (no optical issues to consider), with infinite shutter speed, and infinitely sensitive film.
2) It is snapped when the light from when the bullet being located so its line to the camera forms angle α to the velocity of the bullet, arrives at the camera. At this picture capture time, the bullet is far to the right.
3) More specifically, even though an approximation is made, the logic of the argument is based on the angle α being to the back of the bullet. This is the most convenient case to consider when the bullet is approaching, as in the diagram. For the bullet receding, it would be more convenient to consider angle to the front of the bullet. That is, it is most convenient to analyze from the end of the bullet from which the signal takes longer to reach the camera, for emission events simultaneous in the camera frame.
4) The bullet is considered a 1 dimensional object.
5) The ruler is really considered to be a line along which the bullet moves, i.e. there are no issues of distance between the bullet and the infinitely long ruler.

This answers Anorlunda's challenge ad nauseum.

I prefer (within these assumptions) to set up the problem rigorously, then approximate. I find it easier to explain this way. So, in reference to the diagram in #7, we consider a skinny triangle formed by the camera, bullet rear emission event, and the bullet front emission event that arrives at the camera the same time as the rear emission event. The bullet velocity is taken to be horizontal, per that diagram. For now, I take L to be the distance between the front emission event and the camera, b' is the horizontal side of the skinny triangle, and the long side of the triangle is readily seen to be:

L cos θ + b' cos α

where θ is the angle at the camera between the signals from the emission events. Now, if we were to treat this problem without approximation, neither L nor θ are independent variables, as they both depend (in part) on α. Better is to introduce one new variable k, as the bullet's closest approach distance to the camera. Then it can be seen that:

tan θ = b'sin α / (( k/sin α) - b' cos α)
and
L = (( k/sin α) - b' cos α) / cos θ

Thus, we have only variables k, α, and b', so far.

Now, if we consider the time of the rear emission event to be 0, its arrival time at the camera is:

(b'cos α + L cos θ)/c

The forward transmission event must start later (the simultaneous one would have passed the camera before the rear one arrives). I will call the delay in the forward emission event simply t, insteand of introducing deltas or more primes as post #7 does. Then the arrival time of the forward emission event is simply:

t + L/c

We want to equate these, to require simultaneous signal arrival time at the camera, this:

(b'cos α + L cos θ)/c = t + L/c , eqn (1)

Further, we can see the b' is simply given by:

b' = b/γ + vt , eqn (2)

that is, the contracted length of the bullet plus its travel distance from the rear emission event. Given that L and θ can be expressed in terms of k and the other variables, we se that we can solve (implicitly, at least) for b' in terms of k, b and α.

Now, only, we make an approximation. We assume k is large enough that θ is extremely small, so we may take L cos θ to be simply L. Then we have from equation (1):

b' cos α / c = t

Plugging this in (2) immediately gives:b' = b / [γ(1-βcosα)], β=v/c

I will give a fuller explanation, using the diagram and most variables as specified in post #7. Note, my guess is that the teacher did not make a mistake, instead the OP made a copying error. Trivial algebra from the equations in #7 leads to the formula I give in #9.

To start with, given the discussion of ambiguities, I will explicitly state those used by the teacher, which were also what Nugatory and I posited as the most likely:

0) All description is in the frame of the camera. Quite interesting things can be discovered also considering the bullet frame, but that is not the current problem (e.g. the whole problem can be reduced to aberration that way, without consideration of any signal travel times. The arguments are quite sophisticated and tricky, but the result is almost no computation to write down the answer, even for much more complex problems than this).
1) The camera is an infinitesimal pinhole camera (no optical issues to consider), with infinite shutter speed, and infinitely sensitive film.
2) It is snapped when the light from when the bullet being located so its line to the camera forms angle α to the velocity of the bullet, arrives at the camera. At this picture capture time, the bullet is far to the right.
3) More specifically, even though an approximation is made, the logic of the argument is based on the angle α being to the back of the bullet. This is the most convenient case to consider when the bullet is approaching, as in the diagram. For the bullet receding, it would be more convenient to consider angle to the front of the bullet. That is, it is most convenient to analyze from the end of the bullet from which the signal takes longer to reach the camera, for emission events simultaneous in the camera frame.
4) The bullet is considered a 1 dimensional object.
5) The ruler is really considered to be a line along which the bullet moves, i.e. there are no issues of distance between the bullet and the infinitely long ruler.

This answers Anorlunda's challenge ad nauseum.

I prefer (within these assumptions) to set up the problem rigorously, then approximate. I find it easier to explain this way. So, in reference to the diagram in #7, we consider a skinny triangle formed by the camera, bullet rear emission event, and the bullet front emission event that arrives at the camera the same time as the rear emission event. The bullet velocity is taken to be horizontal, per that diagram. For now, I take L to be the distance between the front emission event and the camera, b' is the horizontal side of the skinny triangle, and the long side of the triangle is readily seen to be:

L cos θ + b' cos α

where θ is the angle at the camera between the signals from the emission events. Now, if we were to treat this problem without approximation, neither L nor θ are independent variables, as they both depend (in part) on α. Better is to introduce one new variable k, as the bullet's closest approach distance to the camera. Then it can be seen that:

tan θ = b'sin α / (( k/sin α) - b' cos α)
and
L = (( k/sin α) - b' cos α) / cos θ

Thus, we have only variables k, α, and b', so far.

Now, if we consider the time of the rear emission event to be 0, its arrival time at the camera is:

(b'cos α + L cos θ)/c

The forward transmission event must start later (the simultaneous one would have passed the camera before the rear one arrives). I will call the delay in the forward emission event simply t, insteand of introducing deltas or more primes as post #7 does. Then the arrival time of the forward emission event is simply:

t + L/c

We want to equate these, to require simultaneous signal arrival time at the camera, this:

(b'cos α + L cos θ)/c = t + L/c , eqn (1)

Further, we can see the b' is simply given by:

b' = b/γ + vt , eqn (2)

that is, the contracted length of the bullet plus its travel distance from the rear emission event. Given that L and θ can be expressed in terms of k and the other variables, we se that we can solve (implicitly, at least) for b' in terms of k, b and α.

Now, only, we make an approximation. We assume k is large enough that θ is extremely small, so we may take L cos θ to be simply L. Then we have from equation (1):

b' cos α / c = t

Plugging this in (2) immediately gives:b' = b / [γ(1-βcosα)], β=v/c

Thank you so much!
I totally get it now!
Do you think my teacher make a mistake in post#7?

 

[andy95220]

This might help you to visualize it:
https://www.spacetimetravel.org/bewegung/bewegung3.html

Thanks!

 

[PAllen]

Thank you so much!
I totally get it now!
Do you think my teacher make a mistake in post#7?

Either you, copying, or the teacher made a mistake, as I noted in my post #9.