I What is the relationship between the Hamiltonian and Lorentz invariance?

[niko_.97]

Hi, I hope this is in the right section. It's for EM which I guess is a relativistic theory but the question itself is not to do with any Lorentz transformations or anything similar.
I'm reading through Jackson with my course for EM and I'm on the section where he is generalising the Hamiltonian and is trying to justify why the Hamiltonian density is the canonical stress tensor. I've included a little extract for reference.

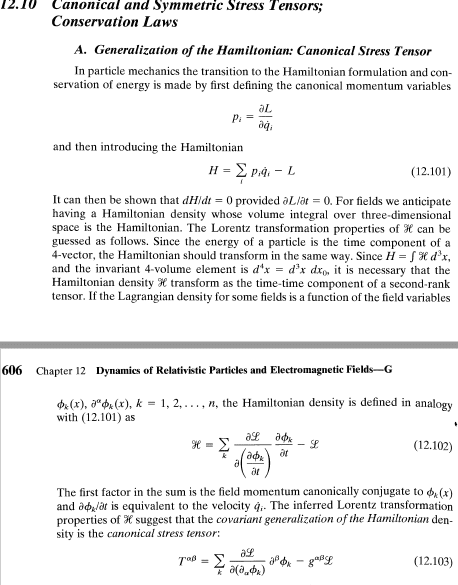
Firstly, why should the Hamiltonian be Lorentz invariant? Is it because, under certain conditions, it is equal to the energy, which we know to be Lorentz invariant? (if so what about when those conditions don't hold? I think the conditions are that the Lagrangian doesn't depend on the conjugate velocities, which it doesn't so that's good, and that the holonomic contrinsts aren't time dependent but I'm not sure what these would be aprt from the ME).
Secondly, when they generalise it to the canonical stress tensor, is it only the 00 component that is the Hamiltonian density? Since that is the component that would have time derivatives and also the component that would give you the energy if integrated wrt d^(3)x.

 

[Orodruin]

Firstly, why should the Hamiltonian be Lorentz invariant?

It should not. He is discussing a covariant generalisation of the Hamiltonian density, not Hamiltonian density.

Is it because, under certain conditions, it is equal to the energy, which we know to be Lorentz invariant?

Energy is not Lorentz invariant.

What he is doing is trying to find some sort of tensorial quantity whose time component is the Hamiltonian density.

 

[niko_.97]

It should not. He is discussing a covariant generalisation of the Hamiltonian density, not Hamiltonian density.Energy is not Lorentz invariant.

What he is doing is trying to find some sort of tensorial quantity whose time component is the Hamiltonian density.

Ah right, thanks. But my question as to why we should expect the Hamiltonian to transform like the energy still stands?

 

[vanhees71]

Well, Hamiltonian formulations for relativistic theories are tricky. Usually, it's save to stick to the non-manifestly (1+3)-formalism or, in my opinion much more convenient, sticking to the Lagrangian version, where you work with manifestly covariant actions. That's why the breakthrough also for manifestly covariant QFT finally has been Feynman's path-integral formalism which after a detour through the Hamiltonian formalism, necessary to ensure unitarity, you almost always can get back to the Lagrangian version with its manifestly covariant actions, leading finally to manifestly covariant Feynman rules and S-matrix elements.

In the case of electromagnetism the next obstacle with Noether's approach to derive the conservation laws is gauge invariance, i.e., you have to take into account that not the potentials themselves build the physical fields, but the potentials modulo gauge transformations. With this approach you immediately get the symmetric energy-momentum tensor of the em. field, which also satisfies the demand of being gauge invariant, i.e., you end up with the Belinfante tensor. Jackson is not the best book for that. Here, I very much prefer Scheck's treatment:

https://www.amazon.com/dp/3662555778/?tag=pfamazon01-20

 

[niko_.97]

Well, Hamiltonian formulations for relativistic theories are tricky. Usually, it's save to stick to the non-manifestly (1+3)-formalism or, in my opinion much more convenient, sticking to the Lagrangian version, where you work with manifestly covariant actions. That's why the breakthrough also for manifestly covariant QFT finally has been Feynman's path-integral formalism which after a detour through the Hamiltonian formalism, necessary to ensure unitarity, you almost always can get back to the Lagrangian version with its manifestly covariant actions, leading finally to manifestly covariant Feynman rules and S-matrix elements.

In the case of electromagnetism the next obstacle with Noether's approach to derive the conservation laws is gauge invariance, i.e., you have to take into account that not the potentials themselves build the physical fields, but the potentials modulo gauge transformations. With this approach you immediately get the symmetric energy-momentum tensor of the em. field, which also satisfies the demand of being gauge invariant, i.e., you end up with the Belinfante tensor. Jackson is not the best book for that. Here, I very much prefer Scheck's treatment:

https://www.amazon.com/dp/3662555778/?tag=pfamazon01-20

Thank, you. I'll take a lok if I can find a version online.
Do you know what is meant when Jackson says the stress energy tensor is not gauge invariant? For me, gauge invariance is when a change inthe potentials doesn't change the fields, but this is different from the fields. Is it to do with the fact that there are still physical quantities inside this stress energy tensor upon integration? So for it to be gauge invariant, those physical quantities have to remain the same when the potentials are changed?

 

[vanhees71]

The naive canonical energy-momentum tensor resulting from Noether's Theorem with the naive realization of space-time translation transformations for the potentials (which is only partially correct, if you ask me),
$$x^{\prime \mu} =x^{\prime \mu} + a^{\mu}, \quad A^{\prime \mu}(x')=A^{\mu}(x)=A^{\mu}(x-a),$$
leads to the canonical energy-momentum tensor
$$\Theta_{\mu \nu}^{(\text{can})} = \frac{1}{4} F_{\rho \sigma} F^{\rho \sigma} \eta_{\mu \nu} - F_{\mu \rho} \partial_{\nu} A^{\rho}$$
with the usual Faraday tensor
$$F_{\mu \nu} = \partial_{\mu} A_{\nu} - \partial_{\nu} A_{\mu}.$$
While this tensor is obviously gauge invariant, the canonical EM tensor is not.

There are two ways out, based on the fact that the symmetries do not uniquely define the densities of the conserved "Noether charges", i.e., you can always add a total derivative
$$T^{\mu \nu} = \Theta_{\mu \nu}^{(\text{can})} + \partial_{\rho} \Omega^{\rho \mu \nu},$$
where $\Omega^{\rho \mu \nu}=-\Omega^{\mu \rho \nu}$. Then automatically (a) if $\Theta_{\mu \nu}^{(\text{can})}$ is conserved, i.e., $\partial^{\mu} \Theta_{\mu \nu}^{(\text{can})}=0$ also $\partial^{\mu} T_{\mu \nu}=0$, i.e., $T_{\mu \nu}$ is as valid an EM tensor as $\Theta_{\mu \nu}^{(\text{can})}$. Then you can choose $\Omega^{\rho \mu \nu}$ such as to make $T^{\mu \nu}$ gauge invariant. Also the additional term doesn't change the total energy and momentum of the fields since due to the conservation of the currents we have
$$P_{\nu} = \int_{\mathbb{R}^3} \mathrm{d}^4 x \Theta_{0 \nu}^{(\text{can})}(x)=\int_{\mathbb{R}^3} \mathrm{d}^4 x T_{0 \nu}(x)=\text{const}.$$

The other, imho more elegant method, is to realize that the physical meaning of the potentials is unchanged by gauge transformations, i.e., any potential differing only by a gauge transformation describes the same physics. This implies that together with all symmetry transformations you can always add a gauge transformation to the potentials. For the translations you rather define
$$A^{\prime \mu}(x')=A^{\mu}(x) + \partial^{\mu} a_{\rho} \chi^{\rho}(x).$$
Here $\chi^{\rho}$ is an arbitrary vector field, i.e., it doesn't alter the physics content of the potentials. Using Noether's theorem this leads to an additional term in the resulting energy-momentum tensor
$$T_{\mu \nu}=\Theta_{\mu \nu}^{(\text{can})} -F_{\mu \rho} ] \partial^{\rho} \chi_{\nu}.$$
Since we are allowed to choose anything for $\chi_{\nu}$, a glance at the definition of the canonical EM tensor tells us that the choice of
$$\chi_{\nu}=-A_{\nu}$$
leads to a gauge-invariant EM tensor,
$$T_{\mu \nu} = {F_{\mu}}^{\rho} F_{\rho \nu} + \frac{1}{4} F_{\rho \sigma} F^{\rho \sigma} \eta_{\mu \nu}.$$
This is not only gauge invariant but also symmetric.

Now the question is, why we should believe that this is the correct energy-momentum-stress tensor as a physical quantity. The obvious place, where the density rather than the integrated total energy and momentum of the fields plays a physically observable roll is general relativity, where any energy-momentum-stress tensor is the source of the gravitational field in the Einstein-Hilbert equations. If you define the EM tensor of any field such, then there's a unique prescription, since it follows from the Lagrangian of the Einstein-Hilbert action together with the Lagrangian of the matter and radiation fields from variations of the metric $g_{\mu \nu}$, leading to the Einstein-Hilbert equations of the gravitational field. For electrodynamics this procedure leads to the above derived EM tensor and thus is the physically relevant energy-momentum-stress tensor of the em. field.

 

[niko_.97]

Sorry for the late reply, I didn't see the notification in mt emails. Thanks for the very detailed answer though. Jackson's explanation is quite smilar to your first one, although he doesn't mention that you can always add a derivative which is useful to know.