Why do two like currents attract (frame of reference)?

[FallenApple]

So the picture is this.

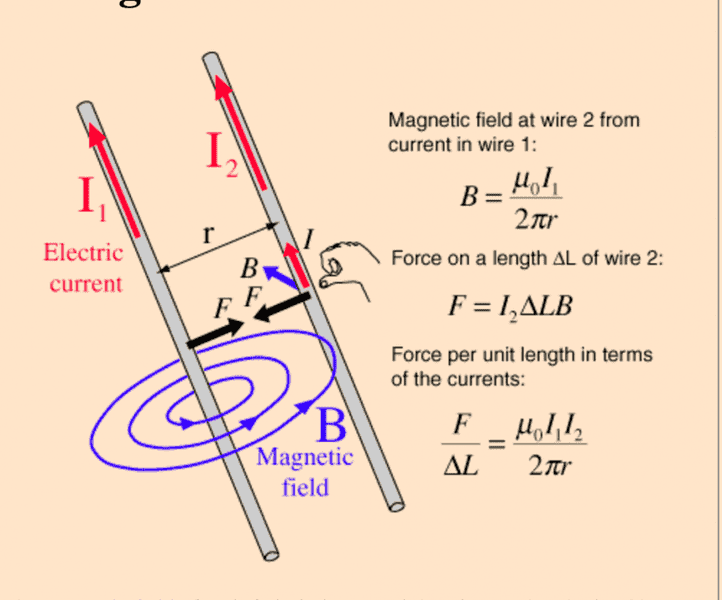
Basically an application of amperes law and Newtons third law shows that they attract.But what about from the frame of reference of the moving currents. Say I have a stream of charges moving to the right, and another stream of charges moving to the right. Both with same velocity and charge density.

Well, in the frame of reference of the moving charges, I just have one row of charges and another row of charges below it. They exert electric forces on each other but not the magnetic force since in this frame, none of the charges are moving relative to each other. If I look at one charge, the left and right charges' effects from the same line cancel. And the left and right charges' effects from the line below will add positively in the vertical while only canceling in the left right direction. And of course, the charge directly below will exert a net force up. In the end, the two currents should be repelling each other when viewed from the frame of reference of the currents.

What resolves this?

 

[BvU]

In the end, the two currents should be repelling each other when viewed from the frame of reference of the currents.

Oh, why ? Are the wires charged ? or just a conduit for the mobile charge carrierss ?

 

[FallenApple]

Oh, why ? Are the wires charged ? or just a conduit for the mobile charge carrierss ?

I'm just using a bunch of positive test charges moving to the right at the same speed and the same configuration separated a distance below and parallel. Not wires per se.

So in the frame of the moving charges, I just have two rows of charges, that are stationary in that frame. And like charges repel like charges.

 

[FallenApple]

I've thought about it from a actual wire's perspective. So wires are fixed positive nucleuses with negative mobile electrons flowing over them.

So from the electrons perspective, assuming that the electron flow on both wires are at the same rate and in the same direction(say the right), from one of the electrons persepctive, none of the other electrons are moving. But the positive nucleuses would be moving to the left. But due to relativistic effects, the postive charges would be lorentz contracted. So in this frame, both wires would still each still have a net charge density. Both being positive from the electrons frame due to the contraction of the protons. So here, the wires should still repel because in this frame, the nucleuses from one wire would repel the nucleuses from the other wire.
However here, the two positive currents would still attract in the electron frame because of ampres law.

Thats why I used moving charges of a single type instead of a wire, because I want to transform away the magnetic effect.

 

[Dale]

What resolves this?

Nothing to resolve. Two lines of the same charge repel in all frames. You have correctly analyzed it.

I will post a link that includes the opposite charges also.

Edit: here it is http://physics.weber.edu/schroeder/mrr/MRRtalk.html

 

[A.T.]

But the positive nucleuses would be moving to the left. But due to relativistic effects, the postive charges would be lorentz contracted.

Yes. See also:

 

[vanhees71]

Let's do a simpler case first. Consider a wire along the $z$ axis carrying a current density $\vec{j}$, i.e.,
$$j^{\mu}=\begin{pmatrix} 0 \\ j \vec{e}_z \end{pmatrix}, \quad \rho<R,$$
where $R$ is the radius of the wire and $\rho=\sqrt{x^2+y^2}$ the usual cylinder coordinate.

Using a circle parallel to the $xy$ plane with radius $\rho>R$ in Ampere's law with the ansatz $\vec{B}=B(\rho) \vec{e}_{\varphi}$ leads to
$$B_{\rho}=\frac{I}{2 \pi c \rho}, \quad I=\pi R^2 j$$.
Now consider a charge $q$ running with velocity $\vec{v}=v \vec{e}_z$ along the $z$ axis. The spatial part of the Lorentz force is
$$\vec{K} = mc \frac{\mathrm{d} \vec{u}}{\mathrm{d} \tau}=q \vec{u} \times \vec{B}=-\frac{\gamma q v}{c} B_{\rho} \vec{e}_{\rho},$$
where
$$c \vec{u}=\gamma \vec{v}=\frac{\vec{v}}{\sqrt{1-\vec{v}^2/c^2}}.$$
Since further $u \cdot K=0$, the time component is given by
$$u^0 K^0=\vec{u} \cdot \vec{K} \; \Rightarrow \; K^0=\frac{\vec{v}}{c} \cdot \vec{K}=0.$$
So we have
$$(K^{\mu})=\begin{pmatrix} 0 \\ -\frac{\gamma q v}{c} B_{\rho} \vec{e}_{\rho} \end{pmatrix}.$$
Now consider the same situation in the rest frame of the particle. We have to Lorentz boost with $\vec{v}$ in $z$ direction, such that in the new frame $u^{\prime 0}=1$, $\vec{u}'=0$, i.e., with the Lorentz matrix
$$({\Lambda^{\mu}}_{\nu})=\begin{pmatrix} \gamma & 0 & 0 & -\gamma v/c \\
0 & 1 & 0 & 0 \\ 0 & 0 & 1 & 0\\
-\gamma v/c & 0 & 0 & \gamma \end{pmatrix}.$$
This immediately leads to
$$K^{\prime \mu}=K^{\mu}.$$
Now, how comes this force about in the rest frame of the particle? That's easily answered by taking the Lorentz transform of the current vector,
$$(j^{\prime \mu}) = ({\Lambda^{\mu}}_{\nu} j^{\nu}) = \begin{pmatrix}-\gamma v j/c \\ 0 \\ 0 \\ \gamma j\end{pmatrix}.$$
As we see, there's a charge density $\varrho=-\gamma v j/c^2$. If you evaluate the corresponding electric field you get
$$\vec{E}=-\frac{\gamma v I}{2 \pi c^2 \rho},$$
and you see that the force is precisely the electrostatic force on the particle at rest in the new reference frame,
$$\vec{K}'=q \vec{E}.$$
So everything is consistent.

You can of course generalize this to the charges in a second current conducting wire, using the superposition principle.

 

[Khashishi]

Normal everyday wires are uncharged, so don't expect a charged wire to act the same way!

 

[lychette]

Let's do a simpler case first. Consider a wire along the $z$ axis carrying a current density $\vec{j}$, i.e.,
$$j^{\mu}=\begin{pmatrix} 0 \\ j \vec{e}_z \end{pmatrix}, \quad \rho<R,$$
where $R$ is the radius of the wire and $\rho=\sqrt{x^2+y^2}$ the usual cylinder coordinate.

Using a circle parallel to the $xy$ plane with radius $\rho>R$ in Ampere's law with the ansatz $\vec{B}=B(\rho) \vec{e}_{\varphi}$ leads to
$$B_{\rho}=\frac{I}{2 \pi c \rho}, \quad I=\pi R^2 j$$.
Now consider a charge $q$ running with velocity $\vec{v}=v \vec{e}_z$ along the $z$ axis. The spatial part of the Lorentz force is
$$\vec{K} = mc \frac{\mathrm{d} \vec{u}}{\mathrm{d} \tau}=q \vec{u} \times \vec{B}=-\frac{\gamma q v}{c} B_{\rho} \vec{e}_{\rho},$$
where
$$c \vec{u}=\gamma \vec{v}=\frac{\vec{v}}{\sqrt{1-\vec{v}^2/c^2}}.$$
Since further $u \cdot K=0$, the time component is given by
$$u^0 K^0=\vec{u} \cdot \vec{K} \; \Rightarrow \; K^0=\frac{\vec{v}}{c} \cdot \vec{K}=0.$$
So we have
$$(K^{\mu})=\begin{pmatrix} 0 \\ -\frac{\gamma q v}{c} B_{\rho} \vec{e}_{\rho} \end{pmatrix}.$$
Now consider the same situation in the rest frame of the particle. We have to Lorentz boost with $\vec{v}$ in $z$ direction, such that in the new frame $u^{\prime 0}=1$, $\vec{u}'=0$, i.e., with the Lorentz matrix
$$({\Lambda^{\mu}}_{\nu})=\begin{pmatrix} \gamma & 0 & 0 & -\gamma v/c \\
0 & 1 & 0 & 0 \\ 0 & 0 & 1 & 0\\
-\gamma v/c & 0 & 0 & \gamma \end{pmatrix}.$$
This immediately leads to
$$K^{\prime \mu}=K^{\mu}.$$
Now, how comes this force about in the rest frame of the particle? That's easily answered by taking the Lorentz transform of the current vector,
$$(j^{\prime \mu}) = ({\Lambda^{\mu}}_{\nu} j^{\nu}) = \begin{pmatrix}-\gamma v j/c \\ 0 \\ 0 \\ \gamma j\end{pmatrix}.$$
As we see, there's a charge density $\varrho=-\gamma v j/c^2$. If you evaluate the corresponding electric field you get
$$\vec{E}=-\frac{\gamma v I}{2 \pi c^2 \rho},$$
and you see that the force is precisely the electrostatic force on the particle at rest in the new reference frame,
$$\vec{K}'=q \vec{E}.$$
So everything is consistent.

You can of course generalize this to the charges in a second current conducting wire, using the superposition principle.

I was just about to write that everything is consistent