- #1
Happiness
- 679
- 30
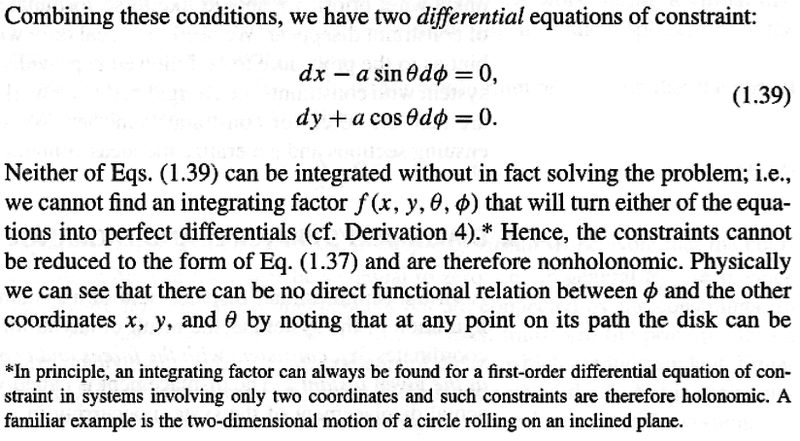
Why can't (1.39) be put in the form of an exact differential? Seems like I could and the solution to the first equation is
##x-a\phi\sin\theta=c##, where ##c## is an arbitrary constant.
Let ##x-a\phi\sin\theta## be ##h##.
By considering ##dh=\frac{\partial h}{\partial x}dx+\frac{\partial h}{\partial \phi}d\phi=0##, we get the first equation of (1.39). So it must be a solution. Isn't it?
Derivation 4:
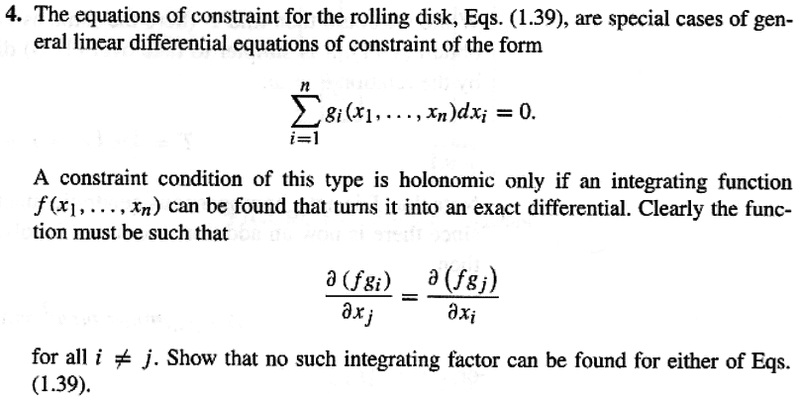
##x-a\phi\sin\theta=c##, where ##c## is an arbitrary constant.
Let ##x-a\phi\sin\theta## be ##h##.
By considering ##dh=\frac{\partial h}{\partial x}dx+\frac{\partial h}{\partial \phi}d\phi=0##, we get the first equation of (1.39). So it must be a solution. Isn't it?
Derivation 4: