- #1
mattlfang
- 28
- 8
- Homework Statement
- See below chart. A ball with hit a two ball system with a spring in between. What's the maximum ratio of masses so the balls will collide one more time.
- Relevant Equations
- The law of momentum conservation, The law of energy conservation
I honeslty don't quite know how to start. It seems like the Hooke's coefficent k is independent of the answer to this problem.
I would also appreciate any clue of expressing the condition when "balls will collide again". The fact that all balls can keep moving make this rather difficult.
It seems to me that "balls will collide again" when the speed of M is greater than the speed of the spring attached system (I could be wrong)? But that doesn't help much since the problem only tells me the collision is elastic.
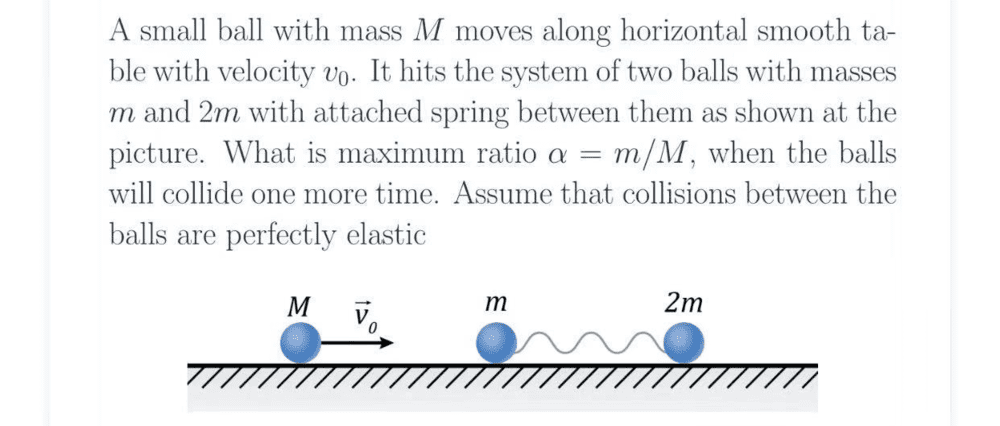
I would also appreciate any clue of expressing the condition when "balls will collide again". The fact that all balls can keep moving make this rather difficult.
It seems to me that "balls will collide again" when the speed of M is greater than the speed of the spring attached system (I could be wrong)? But that doesn't help much since the problem only tells me the collision is elastic.
Last edited by a moderator: