Work done during a collision -- Change in Kinetic Energy & change in Momentum

[Aerisk16]

Homework Statement

work done in an collision which lasted for a very brief time interval

Relevant Equations

J=∆p=p_2-p_1=mv_2-mv_1
J=∑F∆t=F_ave ∆t=F_ave (t_2-t_1 )
W=Fs=∆K=K_2-K_1=1/2 mv_2-1/2 mv_1

Hello guys,

I need help on this problem,

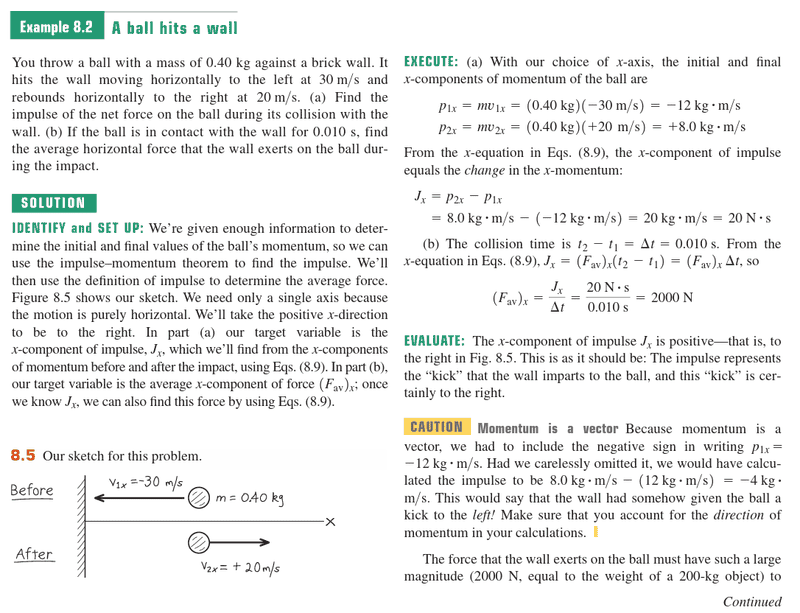
"You throw a ball with a mass of 0.4kg against a brick wall. It hits the wall moving horizontally to the left at 30 m/s and rebounds horizontally to the right at 20m/s. (a) Find the Impulse of the net force on the ball during its collision with the wall (b) If the ball is in contact with the wall for 0.010s, find the average horizontal force that the wall exerts on the ball during the impact."

in the book, the answer for the Impulse is 20 N.s (there's a change in momentum) and the average force is 2000 N determine by dividing the Impulse 20 N.s by the time interval 0.01 s. Since there is change in velocity or speed, there is a change in kinetic energy and since the total work is equal to change in kinetic energy, I divided the change in kinetic energy which is -100 J by the average force 2000 N and get -0.05 m or 5 cm as displacement, I'm thinking this is a deformation of the ball. I'm having a doubt with my answer and also the size of the ball is not given...

 

[jbriggs444]

divided the change in kinetic energy which is -100 J by the average force 2000 N and get -0.05 m or 5 cm as displacement, I'm thinking this is a deformation of the ball. I'm having a doubt with my answer and also the size of the ball is not given...

Your thinking is good. The conclusion may not be as solid as you hope.

Under the assumption of a constant force of 2000 N there must be a 5 cm difference between the compression and the rebound. That would account for a permanent 5 cm deflection of the ball's surface, just as you conclude.

Let us pause for a quick sanity check. The momentum lost and the momentum regained are in a 3:2 proportion. If force is constant then the compression takes 0.006 seconds and rebound takes 0.004 seconds.

That is 0.006 seconds times an average speed of 30 m/s divided by two. That is a 9 cm compression
That is 0.004 seconds times an average speed of 20 m/s divided by two. That is a 4 cm rebound.

Net 5 cm deflection as expected. Sanity check passes.

So we have a ball with a minimum diameter of 9 cm that now has a 5 cm deep dent.But we do not know that force is constant. Indeed, we would expect otherwise. There might, for instance be a force greater than 2000 N during compression and a force less than 2000 N during the rebound. The average force could still be 2000 N and the deflection during compression and rebound could be identical.

Like a slightly under-inflated basketball bouncing from a wall but retaining its original size and shape.It may be worth noting that the momentum analysis allows you to deduce the time-weighted average force. But for an energy analysis, you need a displacement-weighted force. The two averages need not match.