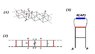
- #1
Tusike
- 139
- 0
Homework Statement
This problem was posted in february in my country's math-physics magazine for high school students, as part of the competition. I couldn't do it then, and every now and again I try it, but still I can't do it, so any help on where to start would be very much appreciated.
http://www.komal.hu/verseny/feladat.cgi?a=honap&h=201002&t=fiz&l=en"
Scroll down and see problem P. 4231. Unfortunately for me, this site has excellent math, physics, and informatics problems, but they usually only provide the solution for the math and informatics ones.
Homework Equations
Not much. R = U/I may help and Kirchoff's equations.
The Attempt at a Solution
As I said, I didn't really get anywhere. I tried to draw down the whole thing as a circuit in 2D, but what I got wasn't pretty, with overlapping wires and all. Normally in problems like these, the idea I saw was that you write down the resistance after n number of cubes have been attached to either side, then the same for n+1, and you say as n nears infinity, they are equal. Normally that equation gives a value for the whole resistance. But I just couldn't write down those equations.
Thanks for any help!
-Tusike
Last edited by a moderator: