Calculating the Component of the weight that acts along a line

[JudgeA]

Homework Statement

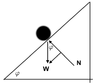
A cyclist rides along a road up an incline at a steady speed of 9.0 m s–1. The mass of the rider and bicycle is 70kg and the bicycle travels 15 m along the road for every 1.0 m gained in height. Neglect energy loss due to frictional forces.

Calculate the component of the weight of the bicycle and the rider that acts along the incline.

2. Homework Equations
sinθ=opp/hyp, not sure what else I need to use

The Attempt at a Solution

Apparently the answer is 46N but I'm really not sure how to get to that. I found an angle of 3.8 degrees by doing 1.0/15 but I'm not sure where to go from there. The Mark scheme says F=sinθ=Wx1.0/15 I don't quite understand what it's saying.

Thanks

 

[CWatters]

Try drawing a diagram showing the weight vector. Then resolve the weight vector into two components, one parallel with the incline and one perpendicular to the incline.

 

[CWatters]

The Mark scheme says F=sinθ=Wx1.0/15 I don't quite understand what it's saying.

I think that's wrong. It should say..

F = W * sin(θ) = W * 1.0/15

 

[JudgeA]

Try drawing a diagram showing the weight vector. Then resolve the weight vector into two components, one parallel with the incline and one perpendicular to the incline.

Yeah I did try that but ended up with 4.6 not 46. I did Sin3.8*70 and got 4.6 so I'm not sure if my angle is wrong?

 

[JudgeA]

I think that's wrong. It should say..

F = W * sin(θ) = W * 1.0/15

Ah ok thanks

 

[Vector1962]

I think every incline problem should start with a sketch, something like the attached file. 3.8 degrees is correct and you found that by knowing the sine of the angle is the sin = opp/hyp = 1.0/15. Referring to the attached pic, if you know W, (the hypotenuse) how do you calculate the opposite side (assuming phi is the angle) of the triangle?

 

[JudgeA]

I think every incline problem should start with a sketch, something like the attached file. 3.8 degrees is correct and you found that by knowing the sine of the angle is the sin = opp/hyp = 1.0/15. Referring to the attached pic, if you know W, (the hypotenuse) how do you calculate the opposite side (assuming phi is the angle) of the triangle?

I think every incline problem should start with a sketch, something like the attached file. 3.8 degrees is correct and you found that by knowing the sine of the angle is the sin = opp/hyp = 1.0/15. Referring to the attached pic, if you know W, (the hypotenuse) how do you calculate the opposite side (assuming phi is the angle) of the triangle?

I assume you'd do Sin3.8*70 as in Opp=Sinθ*hyp however that left me with the answer 4.6 not 46.
(thanks for helping btw)

 

[Vector1962]

I assume you'd do Sin3.8*70 as in Opp=Sinθ*hyp however that left me with the answer 4.6 not 46.
(thanks for helping btw)

What does the force, W equal in terms of the mass, m? W=m x _____

 

[JudgeA]

I assume you'd do Sin3.8*70 as in Opp=Sinθ*hyp however that left me with the answer 4.6 not 46

What does the force, W equal in terms of the mass, m? W=m x _____

Oh of course mass doesn't taken into account gravity, I understand now. Thank you!