Can someone retrace this easy random walk calculation?

[SansaStark]

Okay, it's not easy for me but probably for you ;)

Hello first of all!

I have two questions:
1. Why is there a minus before the expression in the red circle?
2. How did the x((n-1)-1) in the last line come to be?More precise: I understand the first parts. It's the random walk and x signifies the position of a particle after n steps. Then (n-1) ist the position of teh particle having moved to either the left or right side (this is 1-dimensional) and the δ is the distance the particle has travelled. But why is there a minus in the third line before the second 1/N? I mean how do I imagine that visually? I kind of have a notion but I can't really grasp it. And then in the last line why is the [x(n-1)] the same as [x((n-1)-1)]? Huh? oO

Okay, here is the equation:

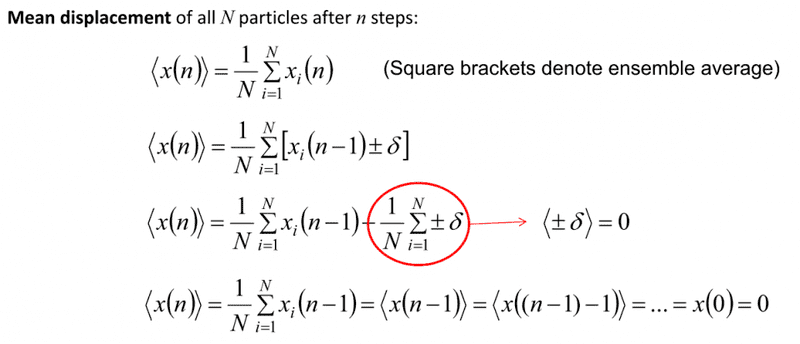
Thanks a lot already!

Vera

 

[SansaStark]

Okay I've looked up the answer to the first question in a book and there it says plus before the term encircled. So maybe it's not minus? Or might be that both are right? Ummm... anyone in the mood for some random walk? ;)

 

[SansaStark]

Ahh... now I get the second question also! HAHA...

So if anyone should care:

In the second equation the author simply plugged in a 1 for the δ meaning that the particles (or the particle) are at zero after 1 step (actually have averaged out each other)! I reckon...

Would be cool if some math guru would leave a comment o my math monologue ;)

 

[Samy_A]

The way I interpret what they do:

1. Why is there a minus before the expression in the red circle?

Don't think it matters, as on average $\displaystyle \sum_{i=1}^n \pm \delta = 0$

2. How did the x((n-1)-1) in the last line come to be?

In the last line, they get $\displaystyle \langle x(n) \rangle=\frac{1}{N}\sum_{i=1}^n x_i(n-1)$, but that is equal to $\langle x(n-1) \rangle$ (see the first formula). And then they repeat the same process down to $\langle x(0) \rangle=0$.

 

[SansaStark]

Okay, I guess I got number one where the minus is simply based on the knowledge of +/- δ being zero thus having no further meaning.

And to the second problem: So does x(0) actually mean n is equal to the value which is substracted from n?
And does (n-1)-1 simply mean n-2?

Thanks!

 

[Samy_A]

Okay, I guess I got number one where the minus is simply based on the knowledge of +/- δ being zero thus having no further meaning.

And to the second problem: So does x(0) actually mean n is equal to the value which is substracted from n?
And does (n-1)-1 simply mean n-2?

Thanks!

I think the $x(0)$ should have been $\langle x(0) \rangle$.
And yes, $(n-1)-1=n-2$. They wrote it that way to emphasize that they are repeating the previous step.
In the last line, they first get $\langle x(n) \rangle=\langle x(n-1) \rangle$. As this hold for any $n$, it can be applied to $n-1$, yielding $\langle x(n-1) \rangle=\langle x((n-1)-1) \rangle=\langle x(n-2) \rangle$ and so on ...

 

[SansaStark]

Oh I guess I have it now. Thanks lot!

 

[fresh_42]

Probably <x(0)> = x(0) = 0 is also true. The ensemble average on nothing (empty sum) results in nothing.