Electric Field due to a line of charge

[stunner5000pt]

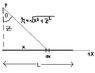
Have a look at the diagram

Find the elctric field a distance z above one end of a astraight line segment of length L which carries uniform line charge lambda. Check that your formula is consistent with what you would expect for the case z >> L

SOlution:
\lambda= q/L
dq = \lambda dx

For the electric Field in the horizontal (points to the left and is negative)

dE_{x} = \frac{1}{4 \pi \epsilon_{0}} \frac{dq}{(z^2 + x^2)} \sin \theta

Subsituting what we know about sin theta and dq

dE_{x} = \frac{1}{4 \pi \epsilon_{0}} \frac{\lambda dx}{(z^2 + x^2)} \frac{x}{\sqrt{z^2 + x^2}}

integrating x = 0 to x = L

E_{x} = \int dE_{x} = \frac{\lambda}{4 \pi \epsilon_{0}} \int_{x=0}^{x=L} \frac{xdx}{(z^2+x^2)^{\frac{3}{2}}} = \frac{\lambda}{4 \pi \epsilon_{0}} \left[ \frac{-1}{\sqrt{z^2 + x^2}} \right]_{x=0}^{x=L} = \frac{\lambda}{4 \pi \epsilon_{0}} \left[ \frac{-1}{\sqrt{z^2 + L^2}} + \frac{1}{z} \right]

E_{x} = \frac{\lambda}{4 \pi \epsilon_{0}} \left[ \frac{1}{z} -\frac{1}{\sqrt{z^2 + L^2}} \right]

ok so suppose z >> L then the electric field is zero?? Shouldnt it reduce to that of a point charge and not zero?? this should be regardless of whether i solve for x or z right??

for the Z direction i got
E_{z} = \frac{\lambda}{4 \pi \epsilon_{0}z} \frac{L}{\sqrt{L^2 + z^2}}
unlike the last one this one does reduce to the equatiopn for a point charge but is off my a factor of 1/z ...

combining the two yields

\vec{E} = \frac{\lambda}{4 \pi \epsilon_{0}} \left[ \frac{1}{z} -\frac{1}{\sqrt{z^2 + L^2}} \right] \hat{x} + \frac{\lambda}{4 \pi \epsilon_{0}z} \frac{L}{\sqrt{L^2 + z^2}} \hat{z}is there soemthing wrong with the calculationg ofr hte X Horizontal Direction?? Please help

Thank you in advance for your help and advice!

 

[Doc Al]

E_{x} = \frac{\lambda}{4 \pi \epsilon_{0}} \left[ \frac{1}{z} -\frac{1}{\sqrt{z^2 + L^2}} \right]

ok so suppose z >> L then the electric field is zero?? Shouldnt it reduce to that of a point charge and not zero?? this should be regardless of whether i solve for x or z right??

If you get far enough away, the field from anything (finite) goes to zero! But you need to understand how it approaches zero. In this case, think about it: You are very far from a point charge--What's the direction of the field?

for the Z direction i got
E_{z} = \frac{\lambda}{4 \pi \epsilon_{0}z} \frac{L}{\sqrt{L^2 + z^2}}
unlike the last one this one does reduce to the equatiopn for a point charge but is off my a factor of 1/z ...

It's not off. You just need to examine its behavior as z goes to infinity. Use a binomial expansion:
(L^2 + z^2)^{-1/2} \approx (1/z)(1 - \frac{L^2}{2z^2})

 

[Peppe]

I'm studying from Griffiths too :) and I've a question about this exercize.

The electric potential:

V = \frac{ \lambda }{4\epsilon_0 \pi} ln\left(\frac{L+\sqrt{z^2+L^2}}{z}\right)


Calculating the electric field from the V's gradient, it has x component always at zero.
Where the problem?

I'm sorry for my poor english, good evening mate!

 

[lepton5]

Yeah, Griffith's book is good.

combining the two yields

\vec{E} = \frac{\lambda}{4 \pi \epsilon_{0}} \left[ \frac{1}{z} -\frac{1}{\sqrt{z^2 + L^2}} \right] \hat{x} + \frac{\lambda}{4 \pi \epsilon_{0}z} \frac{L}{\sqrt{L^2 + z^2}} \hat{z}


is there soemthing wrong with the calculationg ofr hte X Horizontal Direction?? Please help

Thank you in advance for your help and advice!

From your calculation for \vec{E} (yes i think it's correct for both direction), for z >> L, the electric field of component - x will disappear (since \frac{1}{z} - \frac{1}{z} = 0) and leaves for us the z - component as below:


after simplifying, \vec{E} = \frac{\lambda L}{4 \pi \epsilon_{0}z^2}\hat{z} for z>>L.


the case is the same as above example from griffith's book (that is for E at a distance z above midpoint of line L). I quoted it : From far away the line

"looks" like a point charge q = 2\lambda L.

I'm studying from Griffiths too :) and I've a question about this exercize.

The electric potential:

V = \frac{ \lambda }{4\epsilon_0 \pi} ln\left(\frac{L+\sqrt{z^2+L^2}}{z}\right)


Calculating the electric field from the V's gradient, it has x component always at zero.
Where the problem?

I think x and y component will be gone since you do grad \nabla-operation to potential (the potential only depend on z). so,

\vec{E} = -\nabla V = -\frac{\lambda}{4\pi \epsilon_{0}}\frac{\partial}{\partial z}\ln (\frac{L + \sqrt{z^2 + L^2}}{z}).

suppose k = \sqrt{z^2 + L^2},


it will give \vec{E} = \frac{\lambda}{4 \pi \epsilon_{0}}(\frac{z}{k(L + k)} - \frac{1}{z})\hat{z}


btw, i can't find this problem in griffith's book...