Find the value of ##t## when ##P## returns to ##X##- Kinematics

[chwala]

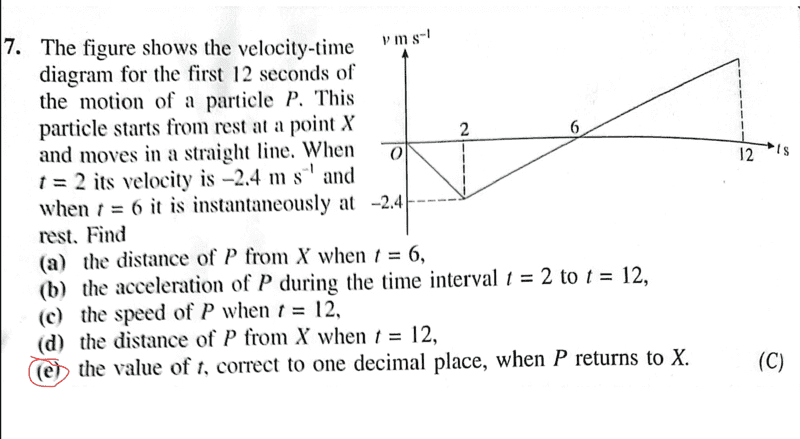
Homework Statement:

Find the value of $t$ when $P$ returns to $X$

Relevant Equations:

Kinematics

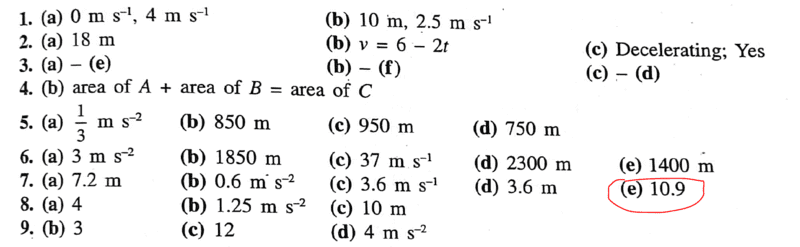
My interest is on part (e) only parts a - d were quite easy. I seek an alternative approach for part (e)

...phew this was a nice one ...took me time to figure out.

Find the text solution here:

My take/approach;

We have $a=0.6 m/s^2$, therefore;

using $s=ut+\dfrac{1}{2}at^2$, we shall have;

$-0.3t^2+3.6t-3.6=0$

$t_1=1.101$seconds (which we shall reject as $6<t<12$).

$t_2=10.89≈10.9$seconds.

cheers! Bingo!!

 

[haruspex]

In my head I did:
3 triangles, 0-2, 2-6, 6-t.
Need first two areas to add up to the third.
First area is half of second (same height, half the base).
So third is 1.5 times area of second.
Similar triangles, so ratio of areas is square of ratio of sides.
t=6+(6-2)√1.5

 

[mjc123]

I don't understand where you get your quadratic equation from. You can't apply s = ut + 1/2at² because a is not constant over the time period. (It works, however, if you work backwards from the end point. Then t = 12 - t₁ = 10.9 s.)

 

[chwala]

I don't understand where you get your quadratic equation from. You can't apply s = ut + 1/2at² because a is not constant over the time period. (It works, however, if you work backwards from the end point. Then t = 12 - t₁ = 10.9 s.)

I actually worked backwards from the end point...is that approach wrong? It should work as he is returning back ... using the same initial acceleration value as start point (deceleration) ...

Furthermore, the question envisages/expects use of an approach in form of calculation that would eventually yield an approximate solution that ought to be rounded to 1 decimal point. Therefore, it requires use of calculations...@haruspex does your approach give an exact or approximate solution?

Cheers...

 

[haruspex]

I actually worked backwards from the end point...is that approach wrong? It should work as he is returning back ... using the same initial acceleration value as start point (deceleration) ...

Furthermore, the question envisages/expects use of an approach in form of calculation that would eventually yield an approximate solution that ought to be rounded to 1 decimal point. Therefore, it requires use of calculations...@haruspex does your approach give an exact or approximate solution?

Cheers...

What I posted was an exact solution.

 

[chwala]

What I posted was an exact solution.

The question expected a numerical approach?that is, for them to specify;

'Give your answer to 1 decimal place?'

If they expected an exact value, then they would not have specified one to give solution to a specified accuracy, in our case to 1 decimal place.

 

[chwala]

I don't understand where you get your quadratic equation from. You can't apply s = ut + 1/2at² because a is not constant over the time period. (It works, however, if you work backwards from the end point. Then t = 12 - t₁ = 10.9 s.)

Which other approach can one apply if not quadratic as you indicate?

 

[haruspex]

The question expected a numerical approach?that is, for them to specify,

'give your answer to 1 decimal place?'

If they expected an exact value, then they would not have specified one to give solution to a specified accuracy, in our case to 1 decimal place.

As I wrote, that was what I worked out in my head. I do not have a calculator in my head. I left you that small step.

 

[mjc123]

Which other approach can one apply if not quadratic as you indicate?

Yes, quadratic equation is the right approach, but it wasn't at all obvious how you derived your equation from your premises. You say you were working backwards from the end point, but you didn't say that originally. Your variable t was not the time from the end, but from the start (you specified 6<t<12). You can't use s = ut + 1/2 at² for t as thus defined, because a is not a constant over the time period. You'd have to split the calculation into two parts (before and after t = 2s).

You need to be much more clear and explicit in

• explaining your approach to tackling the problem
• showing all steps of your derivation and working
• being consistent in notation

 

[chwala]

What is the difficulty in understanding that the motion of the particle $P$ is now reversed in the opposite direction with an unknown $t$ value? with initial deceleration value of $a=0.6$?
using; $s=ut+\dfrac{1}{2}at^2$ we shall have;

$3.6=(3.6×t)+\dfrac{1}{2}(-0.6 ×t^2)$

$3.6=3.6t-0.3t^2$

$-0.3t^2+3.6t-3.6=0$

or

$0.3t^2-3.6t+3.6=0$

...from here the steps to solution will follow.

 

[mjc123]

What is the difficulty in understanding that the motion of the particle P is now reversed in the opposite direction with an unknown t value? with deceleration a=0.6?

You never said that that was what you were doing. You said

Homework Statement:: Find the value of $t$ when $P$ returns to $X$
Relevant Equations:: Kinematics

My take/approach;

We have a=0.6m/s2, therefore;

using s=ut+12at2, we shall have;

−0.3t2+3.6t−3.6=0

t1=1.101seconds (which we shall reject as 6<t<12).

t2=10.89≈10.9seconds.

cheers! Bingo!!

Nothing there about working back from the endpoint! You make a statement about a which is not true over the whole time interval, and state (without deriving) a quadratic equation which is not valid over the whole time interval (because a is not constant). One must assume you are working over the whole time interval from the start of the motion, because this is what t means in the diagram and the question, and you have not said that you intend it to mean anything else, and you say explicitly 6<t<12. I suspect you are using t in your equation in a different sense from t in the question, and perhaps not yourself fully realising that that's what you're doing. If you redefine a variable you should use a different symbol.

If that was the answer to a question in an exam, it would get no marks, even though the numerical answer was coincidentally correct. An examiner would have no idea what you were doing. To get good marks, you MUST state the assumptions you are using, define the symbols in your equation (especially if you're changing the meaning of a symbol), and give initial values or boundary conditions where appropriate. And show your working in full.

It would be better to proceed something like this:

Let us consider the particle's motion backwards from the endpoint at t=12 s. Let us define the time from the endpoint as T = 12 - t. At T = 0, s = 3.6 m, u = -3.6 m/s and a = 0.6 m/s². At the point when s = 0,
0 = 3.6 - 3.6T + 0.3T²
Then you solve the quadratic equation; as T<6, you take the solution T = 1.1 s, so t = 10.9 s.

 

[chwala]

Alternatively, i think we may use integration; that is,

From;

$$v=0.6t-3.6$$

$$\int_t ^{12} (0.6t-3.6) \, dt=3.6$$ Integration yields,

$$[0.3t^2-3.6t]=3.6$$ with limits from $t_m$ to $12$. On substituting our limits we end up with;

$$[43.2-43.2]-[0.3t_m^2-3.6t_m]=3.6$$


$$-0.3t_m^2+3.6t_m-3.6=0$$

from here the steps to solution would follow. In this approach, we deliberately avoided use of acceleration.
Cheers.

 

[chwala]

On second thought, i think we cannot use $s=ut+\dfrac {1}{2} at^2$ as the acceleration (deceleration in our case) is not constant on the entire return journey. The initial deceleration is $0.6$ but this would mean that for us to have $t_1=10.899$, deceleration would have to be increasingly changing to a value much greater than $0.6$

Integration approach would suffice.

 

[SammyS]

On second thought, i think we cannot use $s=ut+\dfrac {1}{2} at^2$ as the acceleration (deceleration in our case) is not constant on the entire return journey. The initial deceleration is $0.6$ but this would mean that for us to have $t_1=10.899$, deceleration would have to be increasingly changing to a value much greater than $0.6$

Integration approach would suffice.

Of course you can use $s=ut+\dfrac {1}{2} at^2$. You do have to know what you are doing. That comes directly from integrating $\displaystyle v=u+at$ provided that you adequately define $s,\,u,\, a \text{, and } t$.

The initial acceleration, from $t=0$ to $t=2.0 \text{ s}$ is $-1.2 \text{ m/s}^2$

After that, from from $t=2.0$ to $t=12.0 \text{ s}$ , $a=(+)0.6 \text{ m/s}^2$

We call it deceleration, from $t=2.0$ to $t=6.0$ because the particle is slowing, but the acceleration does not change at this time ($t = 6.0$).

 

[chwala]

Of course you can use $s=ut+\dfrac {1}{2} at^2$. You do have to know what you are doing. That comes directly from integrating $\displaystyle v=u+at$ provided that you adequately define $s,\,u,\, a \text{, and } t$.

You cannot use it directly as it is. The $s=ut+\dfrac {1}{2} at^2$ has to come from integration.

 

[SammyS]

You cannot use it directly. It has to come from integration.

Why? Is that some rule for this problem?

(I'm still Editing the previous post)

Done editing the previous post.

I will now endeavor to address your Post #12, which shaows some serious confusion.

 

[SammyS]

Alternatively, i think we may use integration; that is,

From;

$$v=0.6t-3.6$$

$$\int_t ^{12} (0.6t-3.6) \, dt=3.6$$ Integration yields,

That is the correct velocity from $t=2.0$ to $t=12.0$ .

Then you write that integral, which is fine, but you don't say why you equate it to $3.6$.

$3.6$ what?

To quote @mjc123 :

Yes, quadratic equation is the right approach, but it wasn't at all obvious how you derived your equation from your premises. You say you were working backwards from the end point, but you didn't say that originally.

How can you work backwards from a situation which you don't explain?

Yes, I figured out that somehow you figured out that at $t=12.0$, the particle was located $+3.6 \text{ m}$ from the starting position.

How you arrived at that is a complete mystery, although @haruspex 's triangles would have gotten you there and even farther.

 

[chwala]

That is the correct velocity from $t=2.0$ to $t=12.0$ .

Then you write that integral, which is fine, but you don't say why you equate it to $3.6$.

$3.6$ what?

To quote @mjc123 :


How can you work backwards from a situation which you don't explain?

Yes, I figured out that somehow you figured out that at $t=12.0$, the particle was located $+3.6 \text{ m}$ from the starting position.

How you arrived at that is a complete mystery, although @haruspex 's triangles would have gotten you there and even farther.

@sammy, the distance of $P$ from $X$ when the particle is at $t=12$ seconds is equal to $3.6$ metres. Unless, you also want me to show this?

Ok, let me take you through this;

1. The distance of $P$ from $X$ when $t=6$ is given by;
$s=\dfrac{1}{2} ×6 × 2.4= 7.2$ metres

2. The distance of $P$ from $X$ when $t=12$ is given by;

i.e from the point where $t=6$ with initial velocity $u=0$.

$v^2=u^2+ 2as$

$3.6^2=0^2+ (2 ×0.6×s)$

$12.96=0+ (2 ×0.6×s)$

$s=10.8$ metres

Therefore, the required $s$ value is given by;

$s_{required}=10.8-7.2=3.6$ metres.

I hope its no longer a mystery ... Cheers.

 

[SammyS]

@sammy, the distance of $P$ from $X$ when the particle is at $t=12$ seconds is equal to $3.6$ metres. Unless, you also want me to show this?

Ok, let me take you through this; . . .

Thank you for providing those details - showing how you determined that at $t=12\text{ seconds, }$ the particle was located $3.6\text{ metres }$ from point $X$ in the positive direction.

For Item 1, it appears that you used the formula for the area of a triangle to find the distance of the particle from $X$ at $t=6\text{ s, }$ rather than performing the integration of $v(t)\,.$ That's fine. The fact that this area falls below the horizontal axis tells us that the integration would give a negative result. Therefore, at $t=6$ seconds, $s=-7.2\text{ metres, }$ indicating that the particle is a distance of $7.2\text{ metres }$ from point $X$ in the negative direction.

Note: Here's an alternate way to find the time at which the particle returns to point $X$.

Integrate $v(t)$ from $6$ to $t_m$, set that equal to $7.2\text{ metres }$ and solve for $t_m$ .