- #1
loginorsinup
- 54
- 2
I am trying to understand the derivation behind the equations for the phase shift incurred when light hits an interface between two lossless dielectrics under total internal reflection (TIR) from what I gathered in S. O. Kasap's Optoelectronics and Photonics.
On the final pair of pages presented here for context there are two equations for the phase shift. I have several questions.
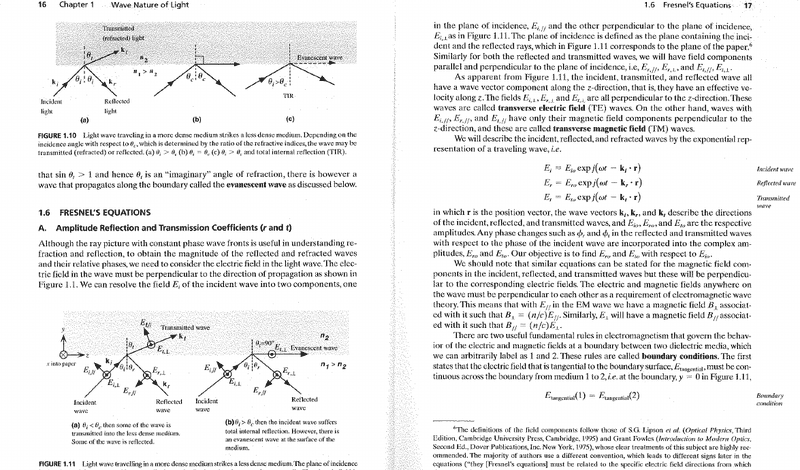
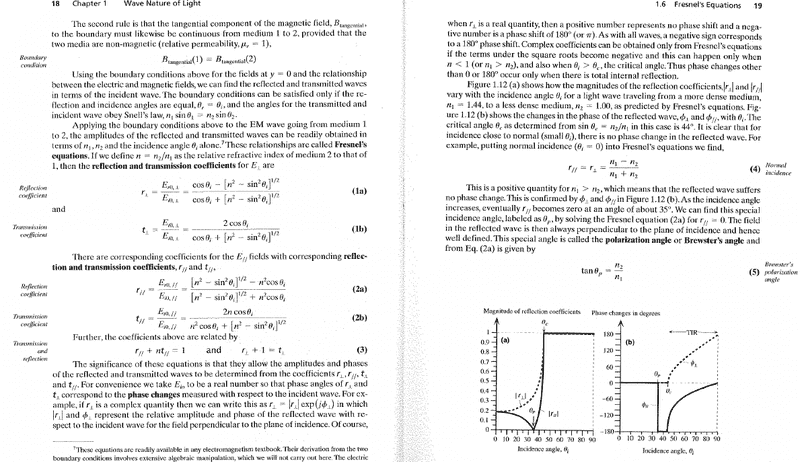

1) Where did this expression come from?
3) How can I use the fact that I know that somehow when TIR occurs, the reflection coefficients for both TE and TM light become complex-valued, and can therefore be represented in polar coordinates (amplitude and phase)?
Lecture slides from just about everywhere on the Internet just take this for granted. I don't even know what to Google for because half the time what I search for turns up stuff about transmission lines and the other half of the time I just get this stuff thrown at me without context.
Thanks in advance for the help.
On the final pair of pages presented here for context there are two equations for the phase shift. I have several questions.
1) Where did this expression come from?
- I get to the point where this comes from Fresnel's equations and that the reflection coefficients can be expressed as complex numbers with magnitudes and phases, but I don't see how that follows to the end result. This book often just jumps to a conclusion, and unless you've seen it before, you end up feeling like you're memorizing something you'll forget in like 3 months.
- Typically, when I see an equation like this I think of some imaginary part divide some real part of something. I am guessing what they somehow did is took the reflection coefficient and expressed it in rectangular coordinates as a complex number, then divided the imaginary component by the real component.
3) How can I use the fact that I know that somehow when TIR occurs, the reflection coefficients for both TE and TM light become complex-valued, and can therefore be represented in polar coordinates (amplitude and phase)?
Lecture slides from just about everywhere on the Internet just take this for granted. I don't even know what to Google for because half the time what I search for turns up stuff about transmission lines and the other half of the time I just get this stuff thrown at me without context.
Thanks in advance for the help.
