Help needed for transformation of stresses in beer and johnston book

[chiraganand]

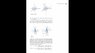
Transformation of stresses in beer and johnston mechanics of materials. While reading the section on trsnformation of stresses they have solved by using the force components in x' and y' directions. I have attached a screenshot of the relevant page and the figure. I have few doubt as to how the force components in the x direction are resolved as shown in the book.

When i tried resolving the forces in x' and y' direction i am not getting the solution as in the book but a totally differnt solution. using the pythagoras theorem to determine the resolved forces.

Could someone please help me out as to how the answer in the book is done?

 

[vela]

Can you show us what you got when you tried? It's a pretty straightforward problem of resolving vectors into their components.

 

[chiraganand]

whenever is try to resolve the horizontal force in the x' direction i am getting the force component as sigma x into area.. whereas in the book he's getting it as sigma x into (cos theeta) ^2 ... don't know where i am gong wrong..

 

[vela]

Well, I can't make sense of what you're trying to say. Could you write it out in math?

 

[chiraganand]

Well, I can't make sense of what you're trying to say. Could you write it out in math?

When i resolve the component of the horizontal force σ ΔA cos θ in the x' direction i am getting = σ ΔA
whereas in the book it is σ (ΔA cos θ)cosθ

 

[vela]

Why are you dividing by the cosine?

 

[chiraganand]

coz when i a m resolving it i am getting cos θ = σΔAcosθ/(component in x' direction)

 

[vela]

You do realize that σΔAcosθ is the hypotenuse, right? It's not one of the legs go the triangle as you appear to be assuming.

 

[chiraganand]

yep now i got it.. taking the reference wrong..