Interchange of limits and integrals

[Lajka]

Hi,

I was wondering about one particular example of this interchange.

In Mallat's book, at the proof of Poisson Formula

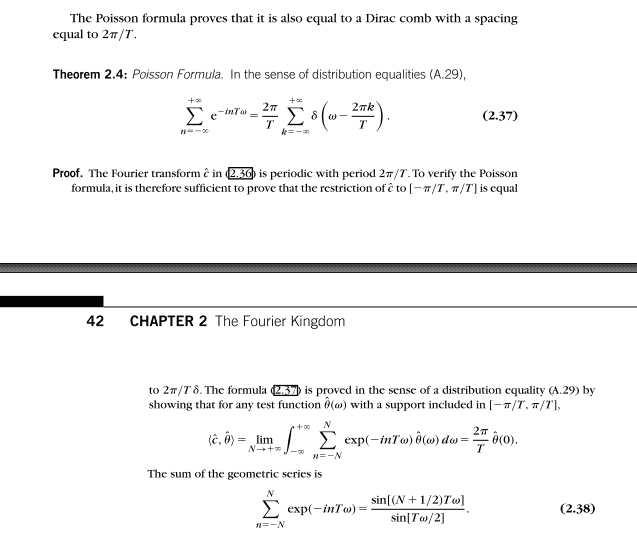
it's visible that the equation at the beginning of the 42nd page features the limit outside of the integral. It is my understanding that this limit had to be in, together with the sum it puts limits on, and then Mallat interchanged the limit and the integral.

I was wondering how would I justifty it, and I wasn't sure. I can't use the dominated convergence theorem, since this sum doesn't converge to anything, (unless we allow Dirac impulses, then it converges in weak sense to this distribution; if I am wrong and someone could correct me on this, I'd be much obliged).

So, could anyone help me with this? I should say I am not that good at math, I am just a simple engineer student.

Many thanks!

 

[pwsnafu]

It says equality as distributions, so you don't interchange the limit and integral.

 

[Lajka]

I am afraid I don't understand, I still see the limit which is put outside of an integral, when I look at the formula.

Would you please elaborate a little?

 

[I like Serena]

Hi Lajka!

Just an engineering student? Hah!

To "just an engineering student" it would suffice that the result exists, from which you can draw the weak implication that the integral converges.
In the engineering world and in the world of theoretical physics that is considered to be enough proof, especially if backed up by experimental results.

Ah well, would the limit and integral converge if the test function $\hat θ(ω)$ was zero?
And what if $\hat θ(ω)$ is "sufficiently small" so that the part that is integrated will be dominated by some function?
Would it hold then (with the help of the dominated convergence theorem)?

 

[Lajka]

Hey Serena! :)

Well, I am just trying to understand it well enough so I can "move on". Perhaps I am aiming too high.

Well, if $\hat θ(ω)=0$, then the whole integral is zero, I guess, problem solved :D

The way I see it, the "problem" is that this term

("the discrete sinc function" some people call it, I believe) grows unboundedly. There is no function that could dominate it, not for all N. So I can't justify the interchange using that theorem.

Of course, this term does converge to the Dirac comb, if I am not mistaken, and if we allow this type of functions.

What pwsnafu says is

It says equality as distributions, so you don't interchange the limit and integral.

which, in other words, means that this is not happening

$\int_{- \infty} ^{+ \infty} \lim_{N\rightarrow +\infty} \sum _{n = -N} ^{+N} e^{-j\omega nT} \hat{\theta}(\omega)d \omega = \lim_{N\rightarrow +\infty} \int_{- \infty} ^{+ \infty} \sum _{n = -N} ^{+N} e^{-j\omega nT} \hat{\theta}(\omega)d \omega$

and I have a hard time seeing it how is it true, judging by the picture above. I am not saying that it isn't, of course, I just can't see it.

Good to hear from you Serena, how have you been? :)

 

[pwsnafu]

In the sequence definition of generalized functions:

Two generalized functions, f = (f₁, f₂, ...) and g = (g₁, g₂, ...), are equal if for all test functions $\theta(x)$ we have
$\lim_{n\rightarrow\infty} \int_{-\infty}^{\infty} f_n(x) \, \theta(x) \, dx = \lim_{n\rightarrow\infty} \int_{-\infty}^\infty g_n(x)\, \theta(x) \, dx$

 

[Lajka]

I am sorry, I know you are trying to help, but I just can't see how that is relevant, although it's nice to know that as well. Maybe the question I am asking is so trivial to you that you simply oversee it, I honestly can't tell.

How did we get from
$\int_{- \infty} ^{+ \infty} \lim_{N\rightarrow +\infty}$
to
$\lim_{N\rightarrow +\infty} \int_{- \infty} ^{+ \infty}$

is literally the only thing I am interested in, at the moment. I guess I'll have to go on without understanding it.

Thanks for the help.

 

[pwsnafu]

Maybe the question I am asking is so trivial to you that you simply oversee it, I honestly can't tell.

How did we get from
$\int_{- \infty} ^{+ \infty} \lim_{N\rightarrow +\infty}$
to
$\lim_{N\rightarrow +\infty} \int_{- \infty} ^{+ \infty}$

We didn't! That's what I'm trying to drill into you. At not time did the interchange happen. The limit was never inside the integral to begin with. It starts with $\langle \hat{c}, \hat{\theta}\rangle$, and by definition of distributions, the limit is on the outside.

In detail: for each N we define
$\hat{c}_N = \sum_{n = -N}^N e^{-inT\omega}$
that is a smooth function (for precisely the Fourier transform of a smooth function).
The distribution, $\hat c$, on the test function $\hat \theta$, is defined as
$\langle \hat{c}, \hat{\theta}\rangle := \lim_{N\rightarrow\infty} \int_{-\infty}^{\infty} \hat{c}_N(\omega) \, \theta(\omega) \, d\omega$

 

[Lajka]

Sorry, I was away.

Ah, I see! That makes sense. I will ponder upon it some more later, but at the moment I have nothing more to ask, it seems crystal clear now.

Thank you! :)