- #1
fog37
- 1,568
- 108
Hello,
I am trying to understand how to apply Bernoulli's equation (which is valid along a single streamline and in the case of zero viscosity) to the situation below.
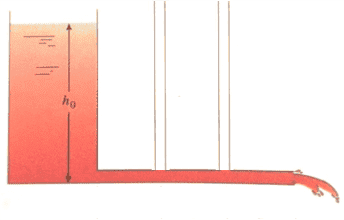
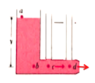
The ideal fluid moves at constant speed along the horizontal pipe. Because the fluid is ideal (zero viscosity), the ideal fluid will reach zero height inside any of the vertical piezometers. Why? I know there is no pressure drop along the horizontal pipe since the viscosity is zero.
If the viscosity was not zero, the fluid would actually reach a nonzero height along the first piezometer and a lower, nonzero height in the second piezometer (to indicate the pressure drop). How come the viscous fluid would manage to go up the piezometric tubes while the ideal fluid does not?
Thanks.
I am trying to understand how to apply Bernoulli's equation (which is valid along a single streamline and in the case of zero viscosity) to the situation below.
The ideal fluid moves at constant speed along the horizontal pipe. Because the fluid is ideal (zero viscosity), the ideal fluid will reach zero height inside any of the vertical piezometers. Why? I know there is no pressure drop along the horizontal pipe since the viscosity is zero.
If the viscosity was not zero, the fluid would actually reach a nonzero height along the first piezometer and a lower, nonzero height in the second piezometer (to indicate the pressure drop). How come the viscous fluid would manage to go up the piezometric tubes while the ideal fluid does not?
Thanks.