Unsteady state mass balance -- Draining of a tank

[MexChemE]

Homework Statement

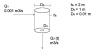
A cylindrical tank, with a diameter D_b, open to the atmosphere, is drained through an orifice in the bottom of the tank with a diameter D_o. The speed of the fluid flowing through the orifice is given by $v = \sqrt{2gh}$, where h is the height of the liquid measured from the bottom of the tank.
Calculate the amount of liquid spilled in 10 minutes (600 seconds), when h₀ = 2 m, D_b = 1 m, and D_o = 0.01 m. The tank is also fed with a constant flow of 0.001 m³ s^-1.

Homework Equations

$$\frac{dm}{dt} = \dot{m}_{in} - \dot{m}_{out}$$

The Attempt at a Solution

I sketched an schematic diagram of the problem, which I attached below. First, since I'm dealing with a pure substance I went ahead and skipped the mass balance and instead started from the dummy volume "balance."
$$\frac{dV}{dt} = Q_1 - Q_2$$
But V = A_bh and Q₂ = A_ov. Also, $v = \sqrt{2gh}$, so:
$$A_b \frac{dh}{dt} = Q_1 - A_o \sqrt{2gh}$$
Separating variables and integrating:
$$\int_{h_0}^h \frac{dh}{\sqrt{h}} = \left( \frac{Q_1}{A_b} - \frac{A_o}{A_b} \sqrt{2g} \right) \int_0^t dt$$
Yields:
$$h = \left( \sqrt{h_0} + 0.5 \left( \frac{Q_1}{A_b} - \frac{A_o}{A_b} \sqrt{2g} \right) t \right)^2$$
Now, calculating both areas yields A_b = 0.785 m² and A_o = 7.854x10^-5 m². This is where trouble begins. Plugging all given values into h(t) yields h = 2.767 m. I would normally calculate the volume of spilled liquid with:
$$V_{\textrm{spilled}} = A_b (h_0 - h)$$
But plugging in these values yields a negative result. Obviously the amount of liquid entering the tank is greater than the amount flowing out, but then, how does one measure the amount of the spilled liquid only? Also, I'm assuming unlimited tank capacity. I tried integrating the exiting flow:
$$V_{\textrm{spilled}} = \int_0^{600} Q(t) dt = A_o \sqrt{2g} \int_0^{600} \sqrt{h}(t) \ dt$$
But it yielded the same negative result. Any ideas?

Thanks in advance for any input!

 

[rollingstein]

With my crude spreadsheet approximation I get 0.3 m3

 

[rollingstein]

Separating variables and integrating:

​

You seem to have a math error here at this step. I don't get your expression

 

[MexChemE]

With my crude spreadsheet approximation I get 0.3 m3

Yes, the given solution was approximately 0.36 m³, IIRC.

You seem to have a math error here at this step. I don't get your expression

I've just realized the mistake I made. I did a similar problem earlier where there was no flow entering the tank so you could just divide the whole equation by h^0.5 in order to separate variables. I just did the same move mechanically without noticing this:
$$\frac{1}{\sqrt{h}} \frac{dh}{dt} = \frac{1}{\sqrt{h}} \frac{Q_1}{A_b} - \frac{A_o}{A_b} \sqrt{2g}$$
This equation is not separable. I will try with the integrating factor method tomorrow. Thanks for your insight!

Edit: Wait, I just noticed I can't solve it by integrating factor either. Maybe Laplace transform?

 

[rollingstein]

Edit: Wait, I just noticed I can't solve it by integrating factor either. Maybe Laplace transform?

Would this help?

http://www4c.wolframalpha.com/Calculate/MSP/MSP7781hc41d1423ia76fd000068i13i36gd2hf2b0?MSPStoreType=image/gif&s=53&w=337.&h=41.

 

[MexChemE]

Would this help?

I couldn't manage to rearrange my equation as shown in the integral above, what I did was to linearize the following DE using a first-order Taylor series expansion, with a = 2:
$$\frac{dh}{dt} + \frac{A_o}{A_b} \sqrt{2g} \sqrt{h} = \frac{Q_1}{A_b}$$
$$\sqrt{h} = \sqrt{2} + \frac{\sqrt{2}}{4} (h-2)$$
$$\frac{dh}{dt} + \frac{A_o}{A_b} \sqrt{2g} \left(\sqrt{2} + \frac{\sqrt{2}}{4} (h-2) \right) = \frac{Q_1}{A_b}$$
$$\frac{dh}{dt} + 0.5 \frac{A_o}{A_b} \sqrt{g} \ h = \frac{Q_1}{A_b} - \frac{A_o}{A_b} \sqrt{g}$$
Letting:
$$G = 0.5 \frac{A_o}{A_b} \sqrt{g}$$
$$K = \frac{Q_1}{A_b} - \frac{A_o}{A_b} \sqrt{g}$$
We arrive at a much simpler first-order linear DE, which I decided to solve by Laplace transform because my LTs were rusty and I wanted to review them:
$$\frac{dh}{dt} + Gh = K$$
$$sH(s) -2 + GH(s) = K \frac{1}{s}$$
$$H(s) = K \left( \frac{1}{s(s-(-G))} \right) + 2 \left( \frac{1}{s-(-G)} \right)$$
Using partial fractions:
$$\frac{1}{s(s-(-G))} = \frac{1}{G} \left(\frac{1}{s} \right) - \frac{1}{G} \left( \frac{1}{s-(-G)} \right)$$
$$H(s) = \frac{K}{G} \left(\frac{1}{s} \right) + \left( 2- \frac{K}{G} \right) \left( \frac{1}{s-(-G)} \right)$$
Applying inverse LT:
$$h = \frac{K}{G} + \left(2- \frac{K}{G} \right) e^{-Gt}$$
Now, calculating the constants yields G = 1.5668x10^-4, K = 9.6052x10^-4, and K/G = 6.1305. Plugging in these values and t = 600 s into h(t) yields h = 2.371 m. If I calculate the volume of spilled liquid using the formula $V_{\textrm{spilled}} = A_b (h_0 - h)$ I get the same result as you did, but negative: -0.3 m³. Am I missing something? Would it work if I drew a control volume in the bottom orifice and set up a new volume balance, taking into account the correct function for h, the one I derived in this post? That would yield the same result but positive, right?

Yes, the given solution was approximately 0.36 m3, IIRC.

The given solution was actually 0.306 m³, so we're pretty close.

 

[rollingstein]

I couldn't manage to rearrange my equation as shown in the integral above,

Take all terms including h to the denominator of the left hand side. Then divide both Numerator & Denominator by Ao * sqrt ( 2g)

 

[MexChemE]

Take all terms including h to the denominator of the left hand side. Then divide both Numerator & Denominator by Ao * sqrt ( 2g)

Let's see:
$$\frac{A_b}{Q_1 - A_o \sqrt{2gh}} \frac{dh}{dt} = 1$$
$$\frac{ \frac{A_b}{A_o \sqrt{2g}}}{\frac{Q_1}{A_o \sqrt{2g}} - \sqrt{h}} \frac{dh}{dt} = 1$$
Letting:
$$E = \frac{A_b}{A_o \sqrt{2g}}$$
$$Z = \frac{Q_1}{A_o \sqrt{2g}}$$
$$E \int_{h_0=2}^h \frac{dh}{Z - \sqrt{h}} = \int_{t=0}^t dt$$
After integrating and some algebra:
$$\sqrt{h} - Z \ln (Z+ \sqrt{h}) = \frac{t}{2E} + \sqrt{2} - Z \ln (Z+ \sqrt{2})$$
Now, I really have no idea how to solve for h, I've been juggling for a while with logarithms and anti-logs, but haven't got anywhere. Thanks for all your insight so far, rollingstein!